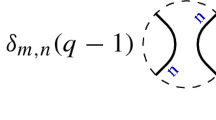Skip to main content
SpringerLink
Log in

DAHAs and Skein Theory

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

We give a skein-theoretic realization of the \(\mathfrak {gl}_n\) double affine Hecke algebra of Cherednik using braids and tangles in the punctured torus. We use this to provide evidence of a relationship we conjecture between the skein algebra of closed links in the punctured torus and the elliptic Hall algebra of Burban and Schiffmann.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Notes

  1. Technically, Frohman and Gelca showed skein algebra is isomorphic to the \(t_{DAHA}=1\), \(q_{DAHA}=s_{skein}\) specialization, but the presentations of Koornwinder and Terwilliger show that this spherical subalgebra is isomorphic to the spherical subalgebra in the \(t_{DAHA} = q_{DAHA} = s_{skein}\) specialization, which is a nontrivial statement.

  2. To be precise, the presentation of \(\mathrm {Sk}(T^2)\) does not depend on the parameter v, so technically the right hand side of the isomorphism should be \(\mathcal {E}_{s,s}\otimes _k \mathbb {C}[v^{\pm 1}]\). Also, see Remark 2.5 for a comparison of this specialization to the \(q=1\) specialization.

  3. The sign difference between the right hand side of (4.1) and the corresponding relation in [MS17] comes from the fact that our convention for the product in the skein algebra here (left element goes below the right element) is the opposite from [MS17].

  4. The existence of this map depends on the assumption that Conjecture 1.5 is true.

References

  1. Aiston, A.K., Morton, H.R.: Idempotents of Hecke algebras of type \(A\). J. Knot Theory Ramif. 7(4), 463–487 (1998)

    Article  MathSciNet  Google Scholar 

  2. Aganagic, M., Shakirov, S.: Knot homology and refined Chern–Simons index. Commun. Math. Phys. 333(1), 187–228 (2015)

    Article  MathSciNet  ADS  Google Scholar 

  3. Bellingeri, P.: On presentations of surface braid groups. J. Algebra 274(2), 543–563 (2004)

    Article  MathSciNet  Google Scholar 

  4. Bullock, D., Frohman, C., Kania-Bartoszyńska, J.: Understanding the Kauffman bracket skein module. J. Knot Theory Ramif. 8(3), 265–277 (1999)

    Article  MathSciNet  Google Scholar 

  5. Bullock, D., Przytycki, J.H.: Multiplicative structure of Kauffman bracket skein module quantizations. Proc. Am. Math. Soc. 128(3), 923–931 (2000)

    Article  MathSciNet  Google Scholar 

  6. Burban, I., Schiffmann, O.: On the Hall algebra of an elliptic curve, I. Duke Math. J. 161(7), 1171–1231 (2012)

    Article  MathSciNet  Google Scholar 

  7. Burella, G., Watts, P., Pasquier, V., Vala, J.: Graphical calculus for the double affine \(Q\)-dependent braid group. Ann. Henri Poincaré 15(11), 2177–2201 (2014)

    Article  MathSciNet  Google Scholar 

  8. Cherednik, I.: Double Affine Hecke Algebras, London Mathematical Society Lecture Note Series, vol. 319. Cambridge University Press, Cambridge (2005)

    Book  Google Scholar 

  9. Carlsson, E., Mellit, A.: A proof of the shuffle conjecture. J. Am. Math. Soc. 31(3), 661–697 (2018)

    Article  MathSciNet  Google Scholar 

  10. Frohman, C., Gelca, R.: Skein modules and the noncommutative torus. Trans. Am. Math. Soc. 352(10), 4877–4888 (2000)

    Article  MathSciNet  Google Scholar 

  11. Jordan, D., Vazirani, M.: The rectangular representation of the double affine Hecke algebra via elliptic Schur-Weyl duality. arXiv e-prints (2017). arXiv:1708.06024

  12. Koornwinder, T.H.: Zhedanov’s algebra \({\rm AW}(3)\) and the double affine Hecke algebra in the rank one case. II. The spherical subalgebra. SIGMA Symmetry Integrability Geom. Methods Appl. vol. 4, Paper 052, 17 (2008)

  13. Lukac, S.G.: Idempotents of the Hecke algebra become Schur functions in the skein of the annulus. Math. Proc. Camb. Philos. Soc. 138(1), 79–96 (2005)

    Article  MathSciNet  Google Scholar 

  14. Morton, H.R.: Skein theory and the Murphy operators. J. Knot Theory Ramif. 11(4), 475–492 (2002) Knots 2000 Korea, Vol. 2 (Yongpyong)

  15. Morton, H., Samuelson, P.: The HOMFLYPT skein algebra of the torus and the elliptic Hall algebra. Duke Math. J. 166(5), 801–854 (2017)

    Article  MathSciNet  Google Scholar 

  16. Morton, H.R., Traczyk, P.: Knots and algebras. In: Martin-Peinador, E., Rodez Usan, A. (eds.) Contribuciones Matematicas en homenaje al profesor D. Antonio Plans Sanz de Bremond , pp. 201–220. University of Zaragoza (1990)

  17. Ni, Y.: Dehn surgeries on knots in product manifolds. J. Topol. 4(4), 799–816 (2011)

    Article  MathSciNet  Google Scholar 

  18. Przytycki, J.H., Sikora, A.S.: On skein algebras and \({\rm Sl}_2({ C})\)-character varieties. Topology 39(1), 115–148 (2000)

    Article  MathSciNet  Google Scholar 

  19. Simental, J.: Notes from lecture two: double affine Hecke algebras

  20. Schiffmann, O., Vasserot, E.: The elliptic Hall algebra, Cherednik Hecke algebras and Macdonald polynomials. Compos. Math. 147(1), 188–234 (2011)

    Article  MathSciNet  Google Scholar 

  21. Schiffmann, O., Vasserot, E.: The elliptic Hall algebra and the \(K\)-theory of the Hilbert scheme of \(\mathbb{A}^2\). Duke Math. J. 162(2), 279–366 (2013)

    Article  MathSciNet  Google Scholar 

  22. Terwilliger, P.: The universal Askey–Wilson algebra and DAHA of type \((C^\vee _1,C_1)\). SIGMA Symmetry Integrability Geom. Methods Appl. 9, Paper 047, 40 (2013)

  23. Turaev, V.G.: The Conway and Kauffman modules of the solid torus with an appendix on the operator invariants of tangles, Progress in knot theory and related topics, Travaux en Cours, vol. 56, pp. 90–102. Hermann, Paris (1997)

Download references

Acknowledgements

This work was initiated during the authors participation in the Research in Pairs program at Oberwolfach in the spring of 2015, and we gratefully acknowledge their support for our stay there, and for their excellent working conditions. More work was done at conferences at the Isaac Newton Institute and at BIRS in Banff, and we gratefully acknowledge their support. Parts of the travel of the second author were supported by a Simons Travel Grant. We thank E. Gorsky, A. Negut, A. Oblomkov, O. Schiffmann, E. Vasserot, M. Vazirani, and K. Walker for their interest and discussions of this and/or their work over the years. We especially thank D. Jordan and A. Mellit for many discussions closely related to this paper. We would also like to thank the referees for thoughtful comments and suggestions that helped us improve the exposition and clarity of the paper. We are grateful to M. Scharlemann for discussions in connection with our revision of Sect. 3.3. The work of the second author has been partially funded by the ERC Grant 637618 and a Simons Foundation Collaboration Grant.

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Liverpool, Liverpool, UK

    H. R. Morton

  2. Department of Mathematics, University of California, Riverside, USA

    Peter Samuelson

Authors
  1. H. R. Morton
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Peter Samuelson
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Peter Samuelson.

Additional information

Communicated by H. Yau

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Morton, H.R., Samuelson, P. DAHAs and Skein Theory. Commun. Math. Phys. 385, 1655–1693 (2021). https://doi.org/10.1007/s00220-021-04052-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-021-04052-8

Search

Navigation