Skip to main content
SpringerLink
Log in

Quon Language: Surface Algebras and Fourier Duality

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

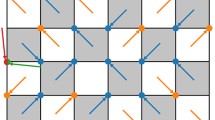
Abstract

Quon language is a 3D picture language that we can apply to simulate mathematical concepts. We introduce the surface algebras as an extension of the notion of planar algebras to higher genus surface. We prove that there is a unique one-parameter extension. The 2D defects on the surfaces are quons, and surface tangles are transformations. We use quon language to simulate graphic states that appear in quantum information, and to simulate interesting quantities in modular tensor categories. This simulation relates the pictorial Fourier duality of surface tangles and the algebraic Fourier duality induced by the S matrix of the modular tensor category. The pictorial Fourier duality also coincides with the graphic duality on the sphere. For each pair of dual graphs, we obtain an algebraic identity related to the S matrix. These identities include well-known ones, such as the Verlinde formula; partially known ones, such as the 6j-symbol self-duality; and completely new ones.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Atiyah M.F.: Topological quantum field theory. Publications Mathématiques de l’IHÉS 68, 175–186 (1988)

    Article  MATH  Google Scholar 

  2. Barrett J.: Geometrical measurements in three-dimensional quantum gravity. Int. J. Mod. Phys. A 18(2), 97–113 (2003)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Biamonte J.: Charged string tensor networks. Proc. Natl. Acad. Sci. 114(10), 2447–2449 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bisch D.: A note on intermediate subfactors. Pac. J. Math. 163, 201–216 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bisch D., Jones V.F.R.: Algebras associated to intermediate subfactors. Invent. Math. 128, 89–157 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  6. Barrett J.W., Westbury B.W.: Spherical categories. Adv. Math. 143(2), 357–375 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  7. Coecke B., Kissinger A.: Picturing Quantum Processes: A First Course in Quantum Theory and Diagrammatic Reasoning. Cambridge University Press, Cambridge (2017)

    Book  MATH  Google Scholar 

  8. Drinfeld V.G.: Quantum groups. Zapiski Nauchnykh Seminarov POMI 155, 18–49 (1986)

    Google Scholar 

  9. Evans D.E., Kawahigashi Y.: Quantum Symmetries on Operator Algebras, vol. 147. Clarendon Press, Oxford (1998)

    MATH  Google Scholar 

  10. Freidel L., Noui K., Roche Ph.: 6j symbols duality relations. J. Math. Phys. 48(11), 113512 (2007)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  11. Gannon T.: Modular data: the algebraic combinatorics of conformal field theory. J. Algebr. Comb. 22(2), 211–250 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  12. Greenberger, D.M., Horne, M.A., Zeilinger, A.: Going beyond Bell’s theorem. In: Kafatos, M. (ed.) Bell’s Theorem, Quantum Theory and Conceptions of the Universe. Fundamental Theories of Physics, vol. 37. Springer, Dordrecht (1989)

  13. Jaffe A., Liu Z.: Mathematical picture language program. Proc. Natl. Acad. Sci. 115(1), 81–86 (2018)

    Article  MathSciNet  Google Scholar 

  14. Jaffe A., Liu Z., Wozniakowski A.: Holographic software for quantum networks. Sci. China Math. 61(4), 593–626 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  15. Jiang C., Liu Z., Wu J.: Noncommutative uncertainty principles. J. Funct. Anal. 270(1), 264–311 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  16. Jones, V.F.R.: Planar algebras, I. arXiv:math/9909027

  17. Jones, V.F.R.: The planar algebra of a bipartite graph. In: Knots in Hellas ’98 (Delphi). Series in Knots Everything, vol. 24, pp. 94–117. World Sci. Publ., River Edge (2000)

  18. Jones V.F.R.: Quadratic tangles in planar algebras. Duke Math. J. 161(12), 2257–2295 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  19. Jones V.F.R., Sunder V.S.: Introduction to Subfactors, vol. 234. Cambridge University Press, Cambridge (1997)

    Book  MATH  Google Scholar 

  20. Kawahigashi Y., Longo R., Müger M.: Multi-interval subfactors and modularity of representations in conformal field theory. Commun. Math. Phys. 219(3), 631–669 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  21. Lafont Y.: Towards an algebraic theory of boolean circuits. J. Pure Appl. Algebra 184(2), 257–310 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  22. Liu Z.: Exchange relation planar algebras of small rank. Trans. AMS 368, 8303–8348 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  23. Liu, Z., Morrison, S., Penneys, D.: Lifting shadings on symmetrically self-dual subfactor planar algebras. To appear Contemporary Mathematics arXiv:1709.05023

  24. Longo R., Rehren K.-H.: Nets of subfactors. Rev. Math. Phys. 7(04), 567–597 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  25. Liu Z., Wozniakowski A., Jaffe A.: Quon 3D language for quantum information. Proc. Natl. Acad. Sci. 114(10), 2497–2502 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  26. Liu, Z., Xu, F.: Jones-Wassermann subfactors for modular tensor categories (2016). arXiv:1612.08573

  27. Moore G., Seiberg N.: Classical and quantum conformal field theory. Commun. Math. Phys. 123(2), 177–254 (1989)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  28. Müger M.: From subfactors to categories and topology I: Frobenius algebras in and Morita equivalence of tensor categories. Journal of Pure and Applied Algebra 180, 81–157 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  29. Müger M.: On the structure of modular categories. Proc. Lond. Math. Soc. 87(2), 291–308 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  30. Ocneanu, A.: Quantized groups, string algebras, and Galois theory for algebras. In: Evans, D., Takesaki, M. (eds.) Operator Algebras and Applications (London Mathematical Society Lecture Note Series), pp. 119–172. Cambridge University Press, Cambridge (1989). https://doi.org/10.1017/CBO9780511662287.008

  31. Robert J.: Skein theory and turaev-viro invariants. Topology 34(4), 771–788 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  32. Reshetikhin N., Turaev V.G.: Invariants of 3-manifolds via link polynomials and quantum groups. Invent. Math. 103(1), 547–597 (1991)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  33. Turaev V.G.: Quantum Invariants of Knots and 3-manifolds, vol.~18. Walter de Gruyter GmbH & Co KG, Cambridge (2016)

    Book  MATH  Google Scholar 

  34. Turaev V.G., Viro O.Y.: State sum invariants of 3-manifolds and quantum 6j-symbols. Topology 31(4), 865–902 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  35. Tambara D., Yamagami S.: Tensor categories with fusion rules of self-duality for finite abelian groups. J. Algebra 209(2), 692–707 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  36. Verlinde E.: Fusion rules and modular transformations in 2D conformal field theory. Nucl. Phys. B 300, 360–376 (1988)

    Article  ADS  MATH  Google Scholar 

  37. Wassermann A.: Operator algebras and conformal field theory III. Fusion of positive energy representations of LSU(N) using bounded operators. Invent. Math. 133(3), 467–538 (1998)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. Witten E.: Topological quantum field theory. Commun. Math. Phys. 117(3), 353–386 (1988)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  39. Xu F.: Jones-Wassermann subfactors for disconnected intervals. Commun. Contemp. Math. 2(03), 307–347 (2000)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author would like to thank Terry Gannon, Arthur Jaffe, Vaughan F. R. Jones, Shamil Shakirov, Cumrun Vafa, Erik Verlinde, Jinsong Wu, and Feng Xu for helpful discussions. The author was supported by Grants TRT0080 and TRT0159 from the Templeton Religion Trust and an AMS-Simons Travel Grant. The author would like to thank the Isaac Newton Institute for Mathematical Sciences, Cambridge, for support and hospitality during the programme “Operator algebras: subfactors and their applications”.

Author information

Authors and Affiliations

  1. Departments of Mathematics and of Physics, Harvard University, Cambridge, USA

    Zhengwei Liu

Authors
  1. Zhengwei Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhengwei Liu.

Additional information

Communicated by C. Schweigert

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, Z. Quon Language: Surface Algebras and Fourier Duality. Commun. Math. Phys. 366, 865–894 (2019). https://doi.org/10.1007/s00220-019-03361-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-019-03361-3

Search

Navigation