Skip to main content
SpringerLink
Log in

Diffusion Approximation for Self-Similarity of Stochastic Advection in Burgers’ Equation

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

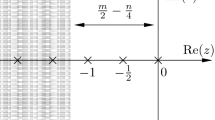
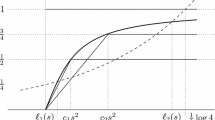
Abstract

Self-similarity of Burgers’ equation with stochastic advection is studied. In self-similar variables a stationary solution is constructed which establishes the existence of a stochastically self-similar solution for the stochastic Burgers’ equation. The analysis assumes that the stochastic coefficient of advection is transformed to a white noise in the self-similar variables. Furthermore, by a diffusion approximation, the long time convergence to the self-similar solution is proved in the sense of distribution.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Arnold L.: Random Dynamical Systems. Springer Monographs in Mathematics. Springer, Berlin (2003)

    Google Scholar 

  2. Becker M., Wayne C.E.: Using global invariant manifolds to understand metastability in the Burgers’ equation with small viscosity. SIAM J. Appl. Dyn. Syst. 8, 1043–1065 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  3. Bertini L., Cancrini N., Jona-Lasinio G.: The stochastic Burgers’ equation. Commun. Math. Phys. 165, 211–232 (1994)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  4. Blömker D., Duan J.: Predictability of the Burgers’ dynamics under model uncertainty. In: Baxendale, P., Lototsky, S. (eds.) Boris Rozovsky 60th Birthday Volume Stochastic Differential Equations: Theory and Applications, pp. 71–90. World Scientific, New Jersey (2007)

    Chapter  Google Scholar 

  5. Bricmont J., Kupiainen A., Xin J.: Global large time self-similarity of a thermal-diffusive combustion system with critical nonlinearity. J. Differ. Equ. 130, 9–35 (1996)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  6. Burgers, J.M.: The Nonlinear Diffusion Equation. D. Reidel, Dordrecht (1974)

  7. Chambers D.H., Adrian R.J., Moin P., Stewart D.S., Sung H.J.: Karhunen–Loéve expansion of Burgers’ model of turblulence. Phys. Fluids 31(9), 2573–2582 (1988)

    Article  ADS  Google Scholar 

  8. Choi H., Temam R., Moin P., Kim J.: Feedback control for unsteady flow and its application to stochastic Burgers’ equation. J. Fluid Mech. 253, 509–543 (1993)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  9. Chow P.L.: Stochastic Partial Differential Equations. Chapman & Hall/CRC, London (2007)

    MATH  Google Scholar 

  10. Chueshov I., Kuksin S.: Stochastic 3D Navier–Stokes equations in a thin domain and its α-approximation. Physica D 10–12, 1352–1367 (2008)

    Article  MathSciNet  Google Scholar 

  11. Davies, I.M., Truman, A., Zhao, H.: Stochastic heat and Burgers’ equations and the intermittence of turbulence. In: Seminar on Stochastic Analysis, Random Fields and Applications IV, pp. 94–110, Progr. Probab., 58. Birkhauser, Basel (2004)

  12. E W., Vanden-Eijnden E.: Asymptotic theory for the probability density functions in Burgers’ turbulence. Phys. Rev. Lett. 83(13), 2572–2575 (1999)

    Article  ADS  Google Scholar 

  13. Ethier S.N., Kurtz T.G.: Markov Processes: Characterization and Convergence. Wiley, New York (1986)

    Book  MATH  Google Scholar 

  14. Escobedo M., Zuazua E.: Large time behavior of for convection–diffusion equation in \({\mathbb{R}^N}\). J. Funct. Anal. 100, 119–161 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  15. Frederiksen, J.S., Zidikheri, M.J.: Stochastic subgrid modelling for atmospheric large eddy simulations. In: Geoffry, N.M., Roberts, A.J. (eds.) Proceedings of the 14th Biennial Computational Techniques and Applications Conference, CTAC-2008, vol. 50 of ANZIAM J., pp. C490–C504 (2008). http://journal.austms.org.au/ojs/index.php/ANZIAMJ/article/view/1365. Accessed 4 Dec 2008

  16. Foias C.: Statistical study of Navier–Stokes equations I. Rend. Sem. Mat. Univ. Padova 48, 219–348 (1972)

    MathSciNet  Google Scholar 

  17. Foias C.: Statistical study of Navier–Stokes equations II. Rend. Sem. Mat. Univ. Padova 49, 9–123 (1973)

    MATH  Google Scholar 

  18. Hayot F., Jayaprakash C.: Aspects of the stochastic Burgers’ equation and their connection with turbulence. Int. J. Mod. Phys. B 14, 1781 (2000)

    Article  ADS  Google Scholar 

  19. Holden H., Lindstrøm T., ØKsendal B., Ubøe J., Zhang T.S.: The Burgers’ equation with a noisy force and the stochastic heat equation. Commun. Partial Differ. Equ. 19(1&2), 119–141 (1994)

    Article  MATH  Google Scholar 

  20. Hosokawa I., Yamamoto K.: Turbulence in the randomly forced one dimensional Burgers’ flow. J. Stat. Phys. 13, 245–272 (1975)

    Article  ADS  Google Scholar 

  21. Imkeller P., Monahan A.H.: Conceptual stochastic climate models. Stochast. Dyn. 2, 311–326 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  22. Jeng D.-T.: Forced model equation for turbulence. Phys. Fluids 12(10), 2006–2010 (1969)

    Article  ADS  MATH  Google Scholar 

  23. Kavian O.: Remarks on the large time behavior of a nonlinear diffusion equation. Ann. Inst. Henri. Poincaré 4(5), 423–452 (1987)

    MATH  MathSciNet  Google Scholar 

  24. Kim Y.J., Tzavaras A.E.: Diffusive N-waves and metastability in the Burgers’ equations. SIAM J. Math. Anal. 33(3), 607–633 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  25. Kushner H.: Approximation and Weak Convergence Methods for Random Processes with Applications to Stochastic Systems Theory. MIT Press, Cambridge, Massachusetts (1984)

    MATH  Google Scholar 

  26. Liu T.P.: Nonlinear stability of shok waves for viscous conservation laws. Mem. Am. Math. Soc. 56(328), 1–108 (1985)

    ADS  Google Scholar 

  27. Maitre E.: On a nonlinear compactness lemma in L p(0, T; B). Int. J. Math. Math. Sci. 27, 1725–1730 (2003)

    Article  MathSciNet  Google Scholar 

  28. Miller J.C., Bernoff A.J.: Rate of convergence to self-similar solutions of Burgers’ equation. Stud. Appl. Math. 111, 29–40 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  29. Metivier M.: Stochastic Partial Differential Equations in Infinite Dimensional Spaces. Scuola Normale Superiore, Pisa (1988)

    MATH  Google Scholar 

  30. Neate, A.D., Truman, A.: On the stochastic Burgers’ equation with some applications to turbulence and astrophysics. In: Morters, P., et al. (eds.) Analysis and Stochastics of Growth Processes and Interface Models, pp. 281–306. OUP (2008)

  31. Da Prato G., Zabczyk J.: Stochastic Equations in Infinite Dimensions. Cambridge University Press, Cambridge (1992)

    Book  MATH  Google Scholar 

  32. Da Prato G., Debussche A., Temam R.: Stochastic Burgers’ equation. NoDEA 1, 389–402 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  33. Da Prato G., Gatarek D.: Stochastic Burgers’ equation with correlated noise. Stochast. Stochast. Rep. 52, 29–41 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  34. Suslov, S.A., Roberts, A.J.: Similarity, attraction and initial conditions in an example of nonlinear diffusion. J. Aust. Math. Soc. B 40(E), E1–E26 (1998). http://anziamj.austms.org.au/ojs/index.php/ANZIAMJ/article/view/453

  35. Sinai Ya.G.: Two results concerning asymptotic behavior of solutions of the Burgers’ equation with force. J. Stat. Phys. 64, 1–12 (1992)

    Article  ADS  MathSciNet  Google Scholar 

  36. Sinai Ya.G.: Statistics of shocks in solutions of inviscid Burgers’ equation. Commun. Math. Phys. 148, 601–621 (1992)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  37. Vishik M.I., Fursikov A.V.: Mathematical problems of statistical hydromechanics. Kluwer Academic Publishers, Dordrecht (1988)

    Book  MATH  Google Scholar 

  38. Walsh J.B.: An introduction to stochastic partial differential equations. Lect. Notes Math. 1180, 265–439 (1986)

    Article  Google Scholar 

  39. Wang, W., Roberts, A.J.: Self similarity and attraction in stochastic nonlinear reaction–diffusion systems. SIAM J. Appl. Dyn. Syst. 12(1), 450–486 (2013). http://arxiv.org/abs/1111.1371

  40. Yoshida K.: Functional Analysis. Springer, Berlin (1980)

    Book  Google Scholar 

  41. Zidikheri, M.J., Frederiksen, J.S.: Parameterization of subgrid scale energy injection in oceanic flows. In: Geoffry, N.M., Roberts, A.J. (eds.) Proceedings of the 14th Biennial Computational Techniques and Applications Conference, CTAC-2008, vol. 50 of ANZIAM J., pp. C459–C473 (2008). http://journal.austms.org.au/ojs/index.php/ANZIAMJ/article/view/1404. Accessed 1 Dec 2008

  42. Zuazua E.: A dynamical system approach to the self-similar large time behavior in scalar convection–diffusion equations. J. Differ. Equ. 108, 1–35 (1994)

    Article  ADS  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Nanjing University, Nanjing, China

    Wei Wang

  2. School of Mathematical Sciences, University of Adelaide, Adelaide, SA, Australia

    A. J. Roberts

Authors
  1. Wei Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. J. Roberts
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wei Wang.

Additional information

Communicated by L. Caffarelli

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, W., Roberts, A.J. Diffusion Approximation for Self-Similarity of Stochastic Advection in Burgers’ Equation. Commun. Math. Phys. 333, 1287–1316 (2015). https://doi.org/10.1007/s00220-014-2117-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-014-2117-7

Keywords

Search

Navigation