Force-based atomistic/continuum blending for multilattices

Abstract

We formulate the blended force-based quasicontinuum method for multilattices and develop rigorous error estimates in terms of the approximation parameters: choice of atomistic region, blending region, and continuum finite element mesh. Balancing the approximation parameters yields a convergent atomistic/continuum multiscale method for multilattices with point defects, including a rigorous convergence rate in terms of the computational cost. The analysis is illustrated with numerical results for a Stone–Wales defect in graphene.

A Notation

This appendix summarizes notation used in the manuscript.

• $\mathcal {L}$—a Bravais lattice

• $\mathcal {M}$—a multilattice

• $\mathcal {S} = \{0, \ldots , S-1\}$—the index set of atomic species

• $\xi$—an element of $\mathcal {L}$ or $\epsilon \mathcal {L}$ for $\epsilon > 0$.

• $\alpha ,\beta ,\gamma ,\delta , \iota , \chi$—indexes denoting atomic species

• $\rho , \tau , \sigma \in \mathcal {L}$—vectors between lattice sites

• $\mathcal {R}$—an interaction range whose elements are triples of the form $(\rho \alpha \beta )\in \mathcal {L} \times \mathcal {S} \times \mathcal {S}$

• $\mathcal {R}_1 := \{ \rho \in \mathcal {L} : \exists (\rho \alpha \beta )\in \mathcal {R}\}$—projection of $\mathcal {R}$ onto lattice direction

• $\mathrm{conv}$—notation for the convex hull of a set

• $r_{\mathrm{cut}}:=\max \{ |\rho |: \rho \in \mathcal {R}_1\}$—a finite cut-off distance for interaction range

• $\nu _x := B_{2r_{\mathrm{cut}}}(0)$—buff region up to twice interaction range

• $r_{\mathrm{cell}}$—the radius of the smallest ball inscribing the unit cell of $\mathcal {L}$

• $r_{\mathrm{buff}} := \max \{ r_{\mathrm{cut}}, r_{\mathrm{cell}}\}$

• $\varvec{u} = \big (u_\alpha \big )_{\alpha = 0}^{S-1}$—vector of displacements of all species of atoms

• $(U, \varvec{p})$—displacement/shift description defined by $U = u_0$ and $p_\alpha = u_\alpha - u_0$

• $\varvec{y}^\mathrm{ref}$ and $\varvec{p}^\mathrm{ref}$—the reference deformation and shifts

• $D_{(\rho \alpha \beta )} \varvec{u}(\xi ) = u_\beta (\xi + \rho ) - u_\alpha (\xi ), D_{(\rho \alpha \beta )}(U,\varvec{p}) = U(\xi +\rho ) - U(\xi ) + p_\beta (\xi + \rho ) - p_\alpha (\xi )$

• $D\varvec{u}(\xi ) = \big (D_{(\rho \alpha \beta )}\varvec{u}(\xi )\big )_{(\rho \alpha \beta )\in \mathcal {R}}, D(U,\varvec{p})(\xi ) = \big (D_{(\rho \alpha \beta )}(U,\varvec{p})(\xi )\big )_{(\rho \alpha \beta )\in \mathcal {R}}$

• $\hat{V}_\xi (D\varvec{y}(\xi ))$ and $V_\xi (D\varvec{u})$—site potentials defined on deformations and displacements, respectively

• $\mathcal {E}^\mathrm{a}(\varvec{u})$ and $\mathcal {E}^\mathrm{a}_{\mathrm{hom}}(\varvec{u})$—energy difference functionals for defective and defect free lattice.

• $\mathcal {T}_{\mathrm{a}}$—atomistic scale finite element mesh of triangles in 2D and tetrahedra in 3D

• $\bar{\zeta }(x), \bar{\zeta }_\xi (x) = \bar{\zeta }(x-\xi )$—nodal basis function of $\mathcal {T}_\mathrm{a}$ associated with the origin and $\xi$ respectively

• $\omega _{\rho }(x):= \int _{0}^1 \bar{\zeta }(x+t\rho )dt$—an auxiliary function

• $Iu_\alpha , IU, Ip_\alpha$ or $\bar{u}_\alpha , \bar{U}, \bar{p}_\alpha$—a piecewise linear interpolant with respect to $\mathcal {T}_\mathrm{a}$

• $\tilde{I}u_\alpha , \tilde{I}U, \tilde{I}p_\alpha$ or $\tilde{u}_\alpha , \tilde{U}, \tilde{p}_\alpha$—a $\mathrm{C}^{2,1}$ interpolant with respect to $\mathcal {T}_\mathrm{a}$

• $u^*(x) := (\bar{\zeta } * \bar{u})(x)$—quasi-interpolant of u defined through convolution

• $|\cdot |$—meaning depends on context: $|\cdot |$ is $\ell ^2$ norm of a vector, matrix, higher order tensor, or finite difference stencil. |T| is area or volume of element T in a finite element partition, $|\gamma |$ is the order of a multiindex.

• $\Vert \cdot \Vert _{\ell ^2(A)}$—$\ell ^2$ norm over a set A. If $f:A \rightarrow \mathbb {R}^d$ is a vector-valued function, $\Vert f\Vert _{\ell ^2(A)} = (\sum _{\alpha \in A}|f(\alpha )|^2)^{1/2}$.

• $\Vert \cdot \Vert _{\mathrm{a}}$—norm on admissible displacements defined by $\Vert \varvec{u}\Vert _\mathrm{a}^2 := \sum _{\alpha = 0}^{S-1}\Vert \nabla Iu_\alpha \Vert _{L^2(\mathbb {R}^d)}^2 + \sum _{\alpha \ne \beta }\Vert Iu_\alpha - Iu_\beta \Vert _{L^2(\mathbb {R}^d)}^2$.

• $\varvec{\mathcal {U}}$ —space of admissible displacements defined by

  $$\begin{aligned} \mathcal {U} :=~ \left\{ \varvec{u} = (u_\alpha )_{\alpha = 0}^{S-1} : u_\alpha :\mathcal {L} \rightarrow \mathbb {R}^n, \Vert \varvec{u}\Vert _\mathrm{a}< \infty \right\} /\mathbb {R}^n \end{aligned}$$

• $\varvec{\mathcal {U}}_0$ —space of test displacements defined by

  $$\begin{aligned} \left\{ (U, \varvec{p}) : \mathrm{supp}(\nabla IU), \, p_0 \equiv 0, \, \text{ and } \, \mathrm{supp}(Ip_\alpha ) \, \text{ are } \text{ compact }\right\} /\mathbb {R}^n \end{aligned}$$

• $\varOmega$—a finite polygonal domain

• $\varphi$ —the blending function

• $\varOmega _\mathrm{a}:=~ \mathrm{supp}(1-\varphi ) + B_{2r_{\mathrm{buff}}}$—the atomistic domain

• $\varOmega _{\mathrm{b}} :=~ \mathrm{supp}(\nabla \varphi ) + B_{2r_{\mathrm{buff}}}$—the blending region

• $\varOmega _\mathrm{c}:=~ {\mathrm{supp}}(\varphi ) \cap \varOmega + B_{2r_{\mathrm{buff}}}$—the continuum region

• $\varOmega _{\mathrm{core}} :=~ \varOmega \setminus \varOmega _\mathrm{c}$—the defect core region

• $\mathcal {T}_h$—the (coarse) finite element mesh on $\varOmega$

• $h(x) := \max _{T: x\in T} \mathrm{Diam}(T)$—the mesh size function

• $R_{\mathrm{t}} := \inf _{R} \{R > 0: \varOmega _{\mathrm{t}} \subset B_{R}(0)\}$—an exterior measure of a domain $\varOmega _{\mathrm{t}}$

• $r_{\mathrm{t}} := \sup _{r}\{r > 0: B_{r}(0) \subset \varOmega _{\mathrm{t}}\}$—an interior measure of a domain $\varOmega _{\mathrm{t}}$

• $R_{\mathrm{o}} := \inf _{R} \{R > 0: \varOmega \subset B_{R}(0)\}$ —an exterior measurement of $\varOmega$

• $r_{\mathrm{i}} := \sup _{r}\{r > 0: B_{r}(0) \subset \varOmega \}$—an interior measurement of $\varOmega$

• $\varOmega _{\mathrm{ext}} := \mathbb {R}^d\setminus B_{r_{\mathrm{i}}/2}(0)$—exterior of $\varOmega$

• $I_h$— the standard piecewise linear nodal interpolant on $\mathcal {T}_h$

• $S_{h}$—the Scott-Zhang quasi-interpolant on $\mathcal {T}_h$.

• $W_{\mathrm{CB}}(U,\varvec{p})$—Cauchy–Born strain energy density function

• $\mathcal {E}^\mathrm{c}(U,\varvec{p})$—Cauchy–Born energy functional

• $\mathcal {U}_h :=~ \left\{ u \in \mathrm{C}^0(\varOmega ) : u|_{T} \in \mathcal {P}_1(T), \quad \forall \, T \in \mathcal {T}_h\right\}$—a finite element space

• $\varvec{\mathcal {U}}_h :=~ \mathcal {U}_h / \mathbb {R}^n$ space of admissible finite element displacements

• $\mathcal {U}_{h,0} :=~ \left\{ u \in \mathrm{C}^0(\mathbb {R}^d): u|_{T} \in \mathcal {P}_1(T), \quad \forall \, T \in \mathcal {T}_h, u = 0 \text{ on } \mathbb {R}^d\setminus \varOmega \right\}$—finite element space satisfying homogeneous boundary conditions

• $\varvec{\mathcal {U}}_{h,0} :=~ \mathcal {U}_{h,0}/ \mathbb {R}^n$—finite element quotient space

• $\varvec{\mathcal {P}}_{h,0} :=~ \{0\} \times (\mathcal {U}_{h,0})^{S-1}$—finite element space for shifts

• $\Vert (U, \varvec{p})\Vert _{\mathrm{ml}}^2 := \Vert \nabla U \Vert _{L^2(\mathbb {R}^d)}^2 + \sum _{\alpha = 0}^{S-1}\Vert p_\alpha \Vert ^2_{L^2(\mathbb {R}^d)} = \Vert \nabla U \Vert _{L^2(\mathbb {R}^d)}^2 + \Vert \varvec{p}\Vert ^2_{L^2(\mathbb {R}^d)}$—norm on finite element spaces

• $\Vert \varvec{p}\Vert _{L^p} := \sum _{\alpha = 0}^{S-1}\Vert p_\alpha \Vert _{L^p}, \Vert \nabla \varvec{p}\Vert _{L^p} := \sum _{\alpha = 0}^{S-1}\Vert \nabla p_\alpha \Vert _{L^p}$

• $\eta (x)$—a smooth bump function or standard mollifying function depending on the context

• $T_{R}u_\alpha (x) = \eta (x/R)\bigg (Iu_\alpha - \frac{1}{|A_R|}\int \limits _{A_R} Iu_0\, dx\bigg )$—a truncation operator

• $\varPi _{h} u_\alpha := S_h (T_{r_{\mathrm{i}}}u_\alpha )$—an projection operator from discrete displacements to finite element displacements

• $\varPi _{h} p_\alpha := \varPi _{h} (u_\alpha - u_0)$ —- a projection operator on shifts

• $[\mathrm{{S}^\mathrm{c}_{\mathrm{d}}}(U,\varvec{q})(x)]_{\beta }$ and $[\mathrm{{S}^\mathrm{c}_{\mathrm{s}}}(U,\varvec{q})(x)]_{\alpha \beta }$— continuum stress function associated with displacements and shifts

• $[\,\mathrm{S}^\mathrm{a}_{\mathrm{d}}(U,\varvec{q})(x)]_\beta$ and $[\,\mathrm{S}^\mathrm{a}_{\mathrm{s}}(U,\varvec{q})(x)]_{\alpha \beta }$ —atomistic stress function associated with displacements and shifts

• $V_{,(\rho \alpha \beta )(\tau \gamma \delta )}\big ( \cdot \big ):v:w :=~ w^{{\top }}\big [V_{,(\rho \alpha \beta )(\tau \gamma \delta )}\big ( \cdot \big )\big ]v \quad \forall v,w \in \mathbb {R}^n$

• $\mathbb {C} : D(W,\varvec{q}): D(Z,\varvec{r}) :=~ \sum _{\begin{array}{c} (\rho \alpha \beta )\\ (\tau \gamma \delta ) \end{array}} V_{,(\rho \alpha \beta )(\tau \gamma \delta )}:D_{(\rho \alpha \beta )}(W,\varvec{q}):D_{(\tau \gamma \delta )}(Z,\varvec{r})$

• $\mathbb {C} : \nabla (W,\varvec{q}): \nabla (Z,\varvec{r}) :=~ \sum _{\begin{array}{c} (\rho \alpha \beta )\\ (\tau \gamma \delta ) \end{array}} V_{,(\rho \alpha \beta )(\tau \gamma \delta )}:(\nabla _\rho W + q_\beta - q_\alpha ):(\nabla _\tau Z + r_\beta - r_\alpha )$

• $\varphi _n$—a sequence of blending functions

• $\psi _n := 1-\varphi _n$

• $\theta _n := \sqrt{\psi _n}$

• $B_{r}, B_{r}(x)$—Ball of radius r about the origin or ball of radius r about x.

• $\mathrm{supp}(f)$—support of a function f.

• $\mathrm{Diam}(U)$—diameter of the set U measured with the Euclidean norm.

• $(\mathbb {R}^n)^{\mathcal {R}}$—direct product of vectors with $|\mathcal {R}|$ terms.

• —transpose of a matrix.

• $\otimes$—tensor product.

• $\nabla ^j$—jth derivative of a function defined on $\mathbb {R}^d$.

• $\partial _\gamma$—multiindex notation for derivatives.

• $L^p(U)$—Standard Lebesgue spaces.

• $W^{k,p}(U)$—Standard Sobolev spaces.

• $W^{k,p}_{\mathrm{loc}}(U) = \left\{ f:U \rightarrow \mathbb {R}^d : f \in W^{k,p}(V) \, \forall V \subset \subset U \right\}$.

• $H^k(U) = W^{k,2}(U)$, $H^1_0(U) = \left\{ f \in H^k(U) : \text{ Trace }(f) = 0~\, \text{ on } \,~ \partial U \right\}$.

• $\mathrm{C}^{k}$—space of k times continuously differentiable functions.

• $C^{k,1}$—space of functions having continuous derivatives up to order k, and whose k-th partial derivatives are Lipschitz continuous.

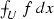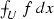• —average value of f over U.