Skip to main content
SpringerLink
Log in

Energy corrected FEM for optimal Dirichlet boundary control problems

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

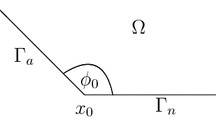
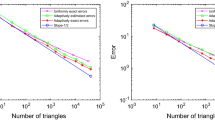
In the presence of re-entrant corners, the solution of PDE-constrained optimization problems, in general, has singular components, even when the given data are smooth. Consequently, the use of standard finite element discretization gives rise to the so-called ’pollution effect’. This means that only a reduced convergence rate, compared to the best approximation error, is obtained. We discuss how optimal convergence rates in weighted norms can be achieved using the idea of energy corrected finite element methods, applied to optimal Dirichlet boundary control problem in the energy space. We present optimal error estimates in weighted norms for the state variable and for the control. Several numerical examples illustrate the obtained theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Apel, T., Mateos, M., Pfefferer, J., Rösch, A.: On the regularity of the solutions of Dirichlet optimal control problems in polygonal domains. SIAM J. Control Optim. 53(6), 3620–3641 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  2. Apel, T., Nicaise, S., Schöberl, J.: A non-conforming finite element method with anisotropic mesh grading for the Stokes problem in domains with edges. IMA J. Numer. Anal. 21(4), 843–856 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  3. Apel, T., Pfefferer, J., Rösch, A.: Finite element error estimates on the boundary with application to optimal control. Math. Comput. 84(291), 33–70 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Apel, T., Sändig, A.-M., Whiteman, J.R.: Graded mesh refinement and error estimates for finite element solutions of elliptic boundary value problems in non-smooth domains. Math. Methods Appl. Sci. 19(1), 63–85 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  5. Babuška, I., Strouboulis, T.: The Finite Element Method and Its Reliability. Numerical Mathematics and Scientific Computation. The Clarendon Press, Oxford University Press, New York (2001)

    MATH  Google Scholar 

  6. Bergounioux, M., Ito, K., Kunisch, K.: Primal-dual strategy for constrained optimal control problems. SIAM J. Control Optim. 37(4), 1176–1194 (1999). (electronic)

    Article  MathSciNet  MATH  Google Scholar 

  7. Blum, H.: The influence of reentrant corners in the numerical approximation of viscous flow problems. In: Numerical treatment of the Navier–Stokes equations (Kiel, 1989), Volume 30 of Notes Numer. Fluid Mechanics, pp. 37–46. Vieweg, Braunschweig (1990)

  8. Brenner, S.C.: Multigrid methods for the computation of singular solutions and stress intensity factors. I. Corner singularities. Math. Comput. 68(226), 559–583 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  9. Brenner, S.C., Scott, L.R.: The Mathematical Theory of Finite Element Methods, Volume 15 of Texts in Applied Mathematics, 3rd edn. Springer, New York (2008)

    Book  Google Scholar 

  10. Cai, Z., Kim, S.: A finite element method using singular functions for the Poisson equation: corner singularities. SIAM J. Numer. Anal. 39, 286–299 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  11. Casas, E., Mateos, M., Tröltzsch, F.: Error estimates for the numerical approximation of boundary semilinear elliptic control problems. Comput. Optim. Appl. 31(2), 193–219 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  12. Ciarlet, P.G., Lions, J.L.: Handbook of Numerical Analysis, vol. II. Finite Element Methods (Part 1). North Holland, Amsterdam, New York, Oxford (1991)

    MATH  Google Scholar 

  13. Collis, S.S., Ghayour, K., Heinkenschloss, M., Ulbrich, M., Ulbrich, S.: Optimal control of unsteady compressible viscous flows. Int. J. Numer. Methods Fluids 40(11), 1401–1429 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  14. de los Reyes, J.C., Kunisch, K.: A semi-smooth Newton method for control constrained boundary optimal control of the Navier–Stokes equations. Nonlinear Anal. 62(7), 1289–1316 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  15. Egger, H., Rüde, U., Wohlmuth, B.: Energy-corrected finite element methods for corner singularities. SIAM J. Numer. Anal. 52(1), 171–193 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  16. Fix, G.: Higher-order Rayleigh–Ritz approximations. J. Math. Mech. 18, 645–657 (1968/1969)

  17. Glowinski, R., Lions, J.L., Trémoliéres, R.: Numerical Analysis of Variational Inequalities. Elsevier, Amsterdam (2011)

    MATH  Google Scholar 

  18. Grisvard, P.: Elliptic Problems in Nonsmooth Domains. Pitman, Boston (1985)

    MATH  Google Scholar 

  19. Haroske, D.D., Triebel, H.: Distributions, Sobolev Spaces, Elliptic Equations. European Mathematical Society, Warsaw (2008)

    MATH  Google Scholar 

  20. Hintermüller, M., Ito, K., Kunisch, K.: The primal-dual active set strategy as a semismooth newton method. SIAM J. Optim. 13(3), 865–888 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  21. Hintermüller, M., Kovtunenko, V.A., Kunisch, K.: The primal-dual active set method for a crack problem with non-penetration. IMA J. Appl. Math. 69(1), 1–26 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  22. Hinze, M., Pinnau, R., Ulbrich, M., Ulbrich, S.: Optimization with PDE Constraints, volume 23 of Mathematical Modelling: Theory and Applications. Springer, New York (2009)

    MATH  Google Scholar 

  23. Hömberg, D., Meyer, C., Rehberg, J., Ring, W.: Optimal control for the thermistor problem. SIAM J. Control Optim. 48(5), 3449–3481 (2009/2010)

  24. Hsiao, G.C., Wendland, W.L.: Boundary Integral Equations. Springer, Berlin (2008)

    Book  MATH  Google Scholar 

  25. Huber, M., John, L., Pustejovska, P., Rüde, U., Waluga, C., Wohlmuth, B.: Solution techniques for the Stokes system: a priori and a posteriori modifications, resilient algorithms. In: Proceedings of the 8th International Congress on Industrial and Applied Mathematics, pp. 109–134. Higher Ed. Press, Beijing (2015)

  26. John, L.: Optimal Boundary Control in Energy Spaces Preconditioning and Applications. Monographic Series TU Graz, Computation in Engineering and Science, vol. 24 (2014)

  27. John, L., Pustejovska, P., Wohlmuth, B., Rüde, U.: Energy-corrected finite element methods for the Stokes system. IMA J. Numer. Anal. (published online) (2016)

  28. Kikuchi, N., Oden, J. T.: Contact Problems in Elasticity: A Study of Variational Inequalities and Finite Element Methods. Studies in Applied and Numerical Mathematics. Society for Industrial and Applied Mathematics, Philadelphia (1988)

  29. Kondratiev, V.A.: Boundary value problems for elliptic equations in domains with conical or angular points. Trans. Mosc. Math. Soc. 16, 227–313 (1967)

    MathSciNet  Google Scholar 

  30. Kufner, A.: Weighted Sobolev spaces. A Wiley-Interscience Publication. Wiley, New York (1985). Translated from the Czech

  31. Kunisch, K., Vexler, B.: Optimal vortex reduction for instationary flows based on translation invariant cost functionals. SIAM J. Control Optim. 46(4), 1368–1397 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  32. Lions, J.-L.: Optimal Control of Systems Governed by Partial Differential Equations. Springer, Berlin, Heidelberg (1971)

    Book  MATH  Google Scholar 

  33. Lubuma, J.M.-S., Patidar, K.C.: Towards the implementation of the singular function method for singular perturbation problems. Appl. Math. Comput. 209, 68–74 (2009)

    MathSciNet  MATH  Google Scholar 

  34. Meyer, C., Rösch, A.: Superconvergence properties of optimal control problems. SIAM J. Control Optim. 43(3), 970–985 (2004). (electronic)

    Article  MathSciNet  MATH  Google Scholar 

  35. Of, G., Phan, T.X., Steinbach, O.: An energy space finite element approach for elliptic Dirichlet boundary control problems. Numer. Math. 129(4), 723–748 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  36. Rüde, U.: Local corrections for eliminating the pollution effect of reentrant corners. Technical Report TUM-INFO-02-89-I01, Institut für Informatik, Technische Universtät München (1989)

  37. Rüde, U., Waluga, C., Wohlmuth, B.: Nested Newton strategies for energy-corrected finite element methods. SIAM J. Sci. Comput. 36(4), A1359–A1383 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  38. Rüde, U., Zenger, C.: On the treatment of singularities in the multigrid method. In: Hackbusch, Wolfgang, Trottenberg, Ulrich (eds.) Multigrid Methods II, Volume 1228 of Lecture Notes in Mathematics, pp. 261–271. Springer, Berlin, Heidelberg (1986)

    Google Scholar 

  39. Scott, L.R., Zhang, S.: Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comput. 54(190), 483–493 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  40. Spann, W.: On the boundary element method for the Signorini problem of the Laplacian. Numer. Math. 65, 337–356 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  41. Steinbach, O.: Boundary element methods for variational inequalities. Numer. Math. 126(1), 173–197 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  42. Toselli, A., Widlund, O.: Domain Decomposition Methods—Algorithms and Theory, Volume 34 of Springer Series in Computational Mathematics. Springer, Berlin (2005)

    Book  MATH  Google Scholar 

  43. Tröltzsch, F.: Optimal Control of Partial Differential Equations. Theory, Methods and Applications. American Mathematical Society, Providence (2010)

    MATH  Google Scholar 

  44. Zenger, C., Gietl, H.: Improved difference schemes for the Dirichlet problem of Poisson’s equation in the neighbourhood of corners. Numer. Math. 30(3), 315–332 (1978)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The financial support by the German Research Foundation (DFG) trough grant WO 671/11-1 and through the International Research Training Group IGDK 1754 “Optimization and Numerical Analysis for Partial Differential Equations with Nonsmooth Structures” is gratefully acknowledged.

Author information

Authors and Affiliations

  1. Institute for Numerical Mathematics, Technische Universität München, 85748, Garching bei München, Germany

    Lorenz John, Piotr Swierczynski & Barbara Wohlmuth

Authors
  1. Lorenz John
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Piotr Swierczynski
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Barbara Wohlmuth
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Piotr Swierczynski.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

John, L., Swierczynski, P. & Wohlmuth, B. Energy corrected FEM for optimal Dirichlet boundary control problems. Numer. Math. 139, 913–938 (2018). https://doi.org/10.1007/s00211-018-0952-8

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-018-0952-8

Mathematics Subject Classification

Search

Navigation