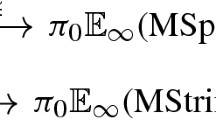Abstract
For a multiplicative cohomology theory E, complex orientations are in bijective correspondence with multiplicative natural transformations to E from complex bordism cohomology MU. If E is represented by a spectrum with a highly structured multiplication, we give an iterative process for lifting an orientation \(MU \rightarrow E\) to a map respecting this extra structure, based on work of Arone–Lesh. The space of strictly commutative orientations is the limit of an inverse tower of spaces parametrizing partial lifts; stage 1 corresponds to ordinary complex orientations, and lifting from stage \((m-1)\) to stage m is governed by the existence of an orientation for a family of E-modules over a fixed base space \(F_m\). When E is p-local, we can say more. We find that this tower only changes when m is a power of p, and if E is E(n)-local the tower is constant after stage \(p^n\). Moreover, if the coefficient ring \(E^*\) is p-torsion free, the ability to lift from stage 1 to stage p is equivalent to a condition on the associated formal group law that was shown necessary by Ando.
Similar content being viewed by others
References
Ando, M., Blumberg, A., Gepner, D. Hopkins, M., Rezk, C.: Units of ring spectra and thom spectra. arXiv:0810.4535
Ando, M., Blumberg, A., Gepner, D.J., Hopkins, M.J., Rezk, C.: Units of ring spectra, orientations and Thom spectra via rigid infinite loop space theory. J. Topol. 7(4), 1077–1117 (2014)
Ando, M., Hopkins, M.J., Rezk, C.: Multiplicative orientations of \(KO\)-theory and of the spectrum of topological modular forms. Preprint. http://www.math.uiuc.edu/~mando/papers/koandtmf.pdf
Ando, M., Hopkins, M.J., Strickland, N.P.: The sigma orientation is an \(H_\infty \) map. Am. J. Math. 126(2), 247–334 (2004)
Arone, G., Lesh, K.: Filtered spectra arising from permutative categories. J. Reine Angew. Math. 604, 73–136 (2007)
Arone, G.Z., Lesh, Kathryn: Augmented \(\Gamma \)-spaces, the stable rank filtration, and a \(bu\) analogue of the Whitehead conjecture. Fund. Math. 207(1), 29–70 (2010)
Ando, M.: Operations in complex-oriented cohomology theories related to subgroups of formal groups, Ph.D. thesis, M.I.T. (1992)
Ando, M.: Isogenies of formal group laws and power operations in the cohomology theories \(E_n\). Duke Math. J. 79(2), 423–485 (1995)
Ando, M.: Power operations in elliptic cohomology and representations of loop groups. Trans. Am. Math. Soc. 352(12), 5619–5666 (2000)
Bruner, R.R., May, J.P., McClure, J.E., Steinberger, M.: \(H_\infty \) ring spectra and their applications. In: Lecture Notes in Mathematics, vol. 1176. Springer, Berlin (1986)
Baker, A., Richter, B.: Some properties of the Thom spectrum over loop suspension of complex projective space. In: An Alpine Expedition Through Algebraic Topology, Contemp. Math., vol. 617, pp. 1–12. Amer. Math. Soc., Providence, RI (2014)
Hopkins, M.J., Kuhn, N.J., Ravenel, D.C.: Generalized group characters and complex oriented cohomology theories. J. Am. Math. Soc. 13(3), 553–594 (2000). (electronic)
Hopkins, M.J., Mahowald, M., Sadofsky, H.: Constructions of Elements in Picard Groups, Topology and Representation Theory (Evanston, IL, 1992), Contemp. Math., vol. 158, pp. 89–126. American Mathematical Society, Providence, RI (1994)
Johnson, N., Noel, J.: For complex orientations preserving power operations, \(p\)-typicality is atypical. Topol. Appl. 157(14), 2271–2288 (2010)
Lazarev, A.: Homotopy theory of \(A_{\infty }\) ring spectra and applications to MU-modules. \(K\)-theory 24(3), 243–281 (2001)
Lawson, T., Naumann, N.: Strictly commutative realizations of diagrams over the Steenrod algebra and topological modular forms at the prime 2. Int. Math. Res. Not. IMRN 10, 2773–2813 (2014)
May, J.P.: The Geometry of Iterated Loop Spaces, Vol. 271, Lecture Notes in Mathematics. Springer, Berlin (1972)
May, J.P.: \(E_{\infty }\) ring spaces and \(E_{\infty }\) ring spectra, Lecture Notes in Mathematics, Vol. 577. Springer, Berlin (1977) (With contributions by Frank Quinn, Nigel Ray, and Jørgen Tornehave)
Möllers, J.-D.: \(K(1)\)-local complex \(E_\infty \)-orientations, Ph.D. thesis. Ruhr-Universität Bochum (2010)
Mathew, A., Stojanoska, V.: The Picard group of topological modular forms via descent theory. Geom. Topol. 20(6), 3133–3217 (2016)
Quillen, D.: Elementary proofs of some results of cobordism theory using Steenrod operations. Adv. Math. 7(1971), 29–56 (1971)
Rezk, C.: Lectures on power operations. http://www.math.uiuc.edu/~rezk/papers.html
Rognes, J.: A spectrum level rank filtration in algebraic \(K\)-theory. Topology 31(4), 813–845 (1992)
Ravenel, D.C., Wilson, W.S.: The Morava \(K\)-theories of Eilenberg–Mac Lane spaces and the Conner–Floyd conjecture. Am. J. Math. 102(4), 691–748 (1980)
Snaith, V.P.: A stable decomposition of \(\Omega ^{n}S^{n}X\). J. Lond. Math. Soc. (2) 7, 577–583 (1974)
Walker, B.J.: Multiplicative orientations of K-Theory and p-adic analysis, ProQuest LLC, Ann Arbor, MI, Thesis (Ph.D.)–University of Illinois at Urbana-Champaign (2008)
Zhu, Y.: Norm coherence for descent of level structures on formal deformations. arXiv:1706.03445
Acknowledgements
The authors would like to thank Greg Arone and Kathryn Lesh for discussions related to this material, to Andrew Baker, Eric Peterson, and Nathaniel Stapleton for their comments, and to Jeremy Hahn for locating an error in the previous version of this paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Michael J. Hopkins partially supported by NSF Grant DMS-0906194.
Tyler Lawson partially supported by NSF Grant DMS-1206008.
Rights and permissions
About this article
Cite this article
Hopkins, M.J., Lawson, T. Strictly commutative complex orientation theory. Math. Z. 290, 83–101 (2018). https://doi.org/10.1007/s00209-017-2009-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00209-017-2009-6