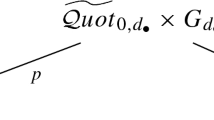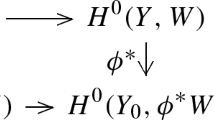Skip to main content
SpringerLink
Log in

On localizations of the characteristic classes of ℓ-adic sheaves and conductor formula in characteristic p > 0

  • Published:
Mathematische Zeitschrift Aims and scope Submit manuscript

Abstract

Abbes, Kato and Saito generalize the Grothendieck-Ogg-Shafarevich formula to an arbitrary dimension (Kato and Saito in Ann. Math. 168:33–96, 2008; Abbes and Saito in Invent. Math. 168:567–612, 2007). In this paper, assuming the strong resolution of singularities, we prove a localized version of a formula proved using the characteristic class of an ℓ-adic sheaf by Abbes and Saito (Invent Math 168:567–612, 2007). We prove a localized version of the Lefschetz-Verdier trace formula proved in Grothendieck (Formule de Lefschetz, exposé III, SGA 5, Lect. Notes Math., vol 589, pp 372–406, Exp. X, Springer, Berlin, 1977 [Théorème 4.4]). As an application, we prove a conductor formula in an arbitrary dimension in the equal characteristic case.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abbes A., Saito T.: The characteristic class and ramification of an ℓ-adic étale sheaf. Invent. Math. 168, 567–612 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  2. Bloch, S.: Cycles on arithmetic schemes and Euler characteristics of curves. In: Algebraic Geometry, Bowdoin, 1985. Proc. Symp. Pure Math. Part 2, vol. 46, pp. 421–450. Amer. Math. Soc., Providence (1987)

  3. Deligne, P.: La formule de dualité globale. In: exposé XVIII, SGA 4 tome 3. Lect. Notes Math., vol. 305, pp. 481–587. Springer, Berlin (1973)

  4. Deligne, P.: Cohomologie étale. In: Seminaire de Geometrie Algebrique du Bois-Marie SGA \({4\frac{1}{2}}\). Lect. Notes Math, vol. 569. Springer, Berlin (1987)

  5. Fulton, W.: Intersection theory, 2nd edn. In: Ergeb. der Math. und ihrer Grenz. 3. Folge. 2 Springer, Berlin (1998)

  6. Grothendieck, A.: rédigé par Illusie L. In: Formule de Lefschetz, exposé III, SGA 5. Lect. Notes Math. Exp. X, vol. 589, pp. 372–406. Springer, Berlin (1977)

  7. Illusie L.: Théorie de Brauer et caractéristique d’Euler-Poincaré. Astérisque 82–83, 161–172 (1981)

    MathSciNet  Google Scholar 

  8. Kato K.: Swan conductors for characters of degree one in the imperfect residue field case, Algebraic K-Theory and algebraic number theory. Contemp. Math. 83, 110–131 (1989)

    Google Scholar 

  9. Kato K.: Class field theory, \({\mathcal{D}}\)-modules and ramification of higher dimensional schemes, Part I. Am. J. Math. 116, 757–784 (1994)

    Article  MATH  Google Scholar 

  10. Kato, K.: Generalized class field theory. In: Proceedings of the International Congress of Mathematicians, Kyoto, 1990, pp. 419–428. Am. Math. Soc., Providence (1991)

  11. Kato K., Saito T.: Ramification theory of schemes over a perfect field. Ann. Math. 168, 33–96 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  12. Kato K., Saito T.: On the conductor formula of Bloch. Publ. Math. IHES 100, 5–151 (2004)

    MathSciNet  MATH  Google Scholar 

  13. Kato K., Saito S., Saito T.: Artin characters of algebraic surfaces. Am. J. Math. 110, 49–75 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  14. Laumon G.: Caractéristique d’Euler-Poincaré des faiceau constructibles sur une surface. Astérisques 101–102, 193–207 (1982)

    Google Scholar 

  15. Matsuda S.: On the Swan conductor in positive characteristic. Am. J. Math. 119(4), 705–739 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  16. Saito S.: General fixed point formula for an algebraic surface and the theory of Swan representations for two-dimensional local rings. Am. J. Math. 109, 1009–1042 (1987)

    Article  MATH  Google Scholar 

  17. Saito T.: Wild ramification and the characteristic cycle of an ℓ-adic sheaf. J. Inst. of Math. Jussieu 8, 769–829 (2008)

    Article  Google Scholar 

  18. Saito, T.: The Euler numbers of l-adic sheaves of rank 1 in positive characteristic. In: ICM90 Satellite Conference Proceedings, Arithmetic and Algebraic geometry, pp. 165–181. Springer (1991)

  19. Tsushima, T.: On localizations of the characteristic classes of ℓ-adic sheaves of rank 1. In: RIMS Kôkyûroku Bessatsu, vol. B12 (2009). Algebraic Number Theory and Related Topics, pp. 193–207 (2007)

  20. Vidal I.: Formule du conducteur pour un caractère ℓ-adique. Compositio Mathematica 145, 687–717 (2009)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Graduate School of Mathematical Sciences, The University of Tokyo, 3-8-1 Komaba, Meguro-ku, Tokyo, 153-8914, Japan

    Takahiro Tsushima

Authors
  1. Takahiro Tsushima
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Takahiro Tsushima.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Tsushima, T. On localizations of the characteristic classes of ℓ-adic sheaves and conductor formula in characteristic p > 0. Math. Z. 269, 411–447 (2011). https://doi.org/10.1007/s00209-010-0743-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00209-010-0743-0

Keywords

Search

Navigation