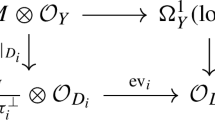Skip to main content
SpringerLink
Log in

Varieties with quadratic entry locus, I

  • Published:
Mathematische Annalen Aims and scope Submit manuscript

Abstract

We introduce and study (L)QEL-manifolds \({X \subset \mathbb P^N}\) of type δ, a class of projective varieties whose extrinsic and intrinsic geometry is very rich, especially when δ >  0. We prove a strong Divisibility Property for LQEL-manifolds of type δ ≥  3, allowing the classification of those of type \({\delta \geq \frac{dim(X)}{2}}\) . In particular we obtain a new and very short proof that Severi varieties have dimension 2,4, 8 or 16 and also an almost self-contained proof of their classification due to Zak. We also provide the classification of special Cremona transformations of type (2,3) and (2,5).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Barth W., Larsen M.E.: On the homotopy groups of complex projective algebraic manifolds. Math. Scand. 30, 88–94 (1972)

    MATH  MathSciNet  Google Scholar 

  2. Bertram A., Ein L., Lazarsfeld R.: Vanishing theorems, a theorem of Severi and the equations defining projective varieties. J. Am. Math. Soc. 4, 587–602 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  3. Chaput P.E.: Severi varieties. Math. Z. 240, 451–459 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  4. Ciliberto C., Mella M., Russo F.: Varieties with one apparent double point. J. Algebraic Geom. 13, 475–512 (2004)

    MATH  MathSciNet  Google Scholar 

  5. Crauder B., Katz S.: Cremona transformations with smooth irreducible fundamental locus. Am. J. Math. 111, 289–309 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  6. Curtis C.W.: Linear Algebra—An Introductory Approach, Undergraduate Texts in Mathematics, corrected 6th edn. Springer, Berlin (1997)

    Google Scholar 

  7. Debarre O.: Higher-Dimensional Algebraic Geometry. Universitext, Springer, USA (2001)

    MATH  Google Scholar 

  8. Ein L.: Varieties with small dual variety. I. Invent. Math. 86, 63–74 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  9. Ein, L.: Vanishing theorems for varieties of low codimension, L.N.M., vol. 1311, pp. 71–75. Springer, Berlin

  10. Ein L., Shepherd-Barron N.: Some special Cremona transformations. Am. J. Math. 111, 783–800 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  11. Fu B.: Inductive characterizations of hyperquadrics. Math. Ann. 340, 185–194 (2008)

    Article  MathSciNet  Google Scholar 

  12. Fujita T.: Projective threefolds with small secant varieties. Sci. Papers Coll. Gen. Ed. Univ. Tokyo 32, 33–46 (1982)

    MATH  Google Scholar 

  13. Fujita, T.: Classification theory of polarized varieties. Lond. Math. Soc. Lec. Notes Ser. 155 (1990)

  14. Fujita T., Roberts J.: Varieties with small secant varieties: the extremal case. Am. J. Math. 103, 953–976 (1981)

    Article  MATH  MathSciNet  Google Scholar 

  15. Griffiths P., Harris J.: Algebraic geometry and local differential geometry. Ann. Sci. Ecole Norm. Sup. 12, 355–432 (1979)

    MATH  MathSciNet  Google Scholar 

  16. Hartshorne R.: Varieties of small codimension in projective space. Bull. A. M. S. 80, 1017–1032 (1974)

    Article  MATH  MathSciNet  Google Scholar 

  17. Holme A., Roberts J.: Zak’s theorem on superadditivity. Ark. Mat. 32, 99–120 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  18. Hulek K., Katz S., Schreyer F.O.: Cremona transformations and syzygies. Math. Z. 209, 419–443 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  19. Hwang, J.M.: Geometry of Minimal Rational Curves on Fano Manifolds. In: Vanishing theorems and effective results in algebraic geometry. I.C.T.P. Lecture Notes, vol. 6, pp. 335–393

  20. Hwang, J.M.: Rigidity of rational homogeneous spaces, International Congress of Mathematicians, vol. II, pp. 613–626. European Mathematical Society, Zürich (2006)

  21. Hwang J.M., Kebekus S.: Geometry of chains of minimal rational curves. J. Reine Angew. Math. 584, 173–194 (2005)

    MATH  MathSciNet  Google Scholar 

  22. Hwang J.M., Mok N.: Rigidity of irreducible Hermitian symmetric spaces of the compact type under Kähler deformation. Invent. Math. 131, 393–418 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  23. Hwang J.M., Mok N.: Birationality of the tangent map for minimal rational curves. Asian J. Math. 8, 51–63 (2004)

    MATH  MathSciNet  Google Scholar 

  24. Hwang J.M., Mok N.: Prolongations of infinitesimal linear automorphisms of projective varieties and rigidity of rational homogeneous spaces of Picard number 1 under Kähler deformation. Invent. Math. 160, 591–645 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  25. Ionescu P.: Embedded projective varieties of small invariants. Springer L.N.M. 1056, 142–186 (1984)

    MathSciNet  Google Scholar 

  26. Ionescu, P., Russo, F.: Conic-connected manifolds, math.AG/0701885

  27. Ionescu P., Russo F.: Varieties with quadratic entry locus, II. Comp. Math. 144, 949–962 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  28. Ivey T.A., Landsberg J.M.: Cartan for Beginners: Differential Geometry via Moving Frames and Exterior Differential Systems, Graduate Studies in Mathematics, vol. 61. American Mathematical Society, USA (2003)

    Google Scholar 

  29. Kollár, J.: Rational Curves on Algebraic Varieties, Erg. der Math., vol. 32. Springer, Germany (1996)

  30. Kollár J., Miyaoka Y., Mori S.: Rationally connected varieties. J. Algebraic Geom. 1, 429–448 (1992)

    MATH  MathSciNet  Google Scholar 

  31. Kachi, Y., Sato, E.: Segre’s Reflexivity and an Inductive Characterization of Hyperquadrics, Mem, vol. 160, no. 763. American Mathematical Society, USA (2002)

  32. Landsberg J.M.: On degenerate secant and tangential varieties and local differential geometry. Duke Math. J. 85, 605–634 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  33. Landsberg J.M.: On the infinitesimal rigidity of homogeneous varieties. Compos. Math. 118, 189–201 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  34. Landsberg J.M.: Griffiths-Harris rigidity of compact hermitian symmetric spaces. J. Diff. Geom. 74, 395–405 (2006)

    MATH  MathSciNet  Google Scholar 

  35. Lam, T.Y.: Introduction to Quadratic Forms over Fields, Graduate Studies in Mathematics, vol. 67. American Mathematical Society, USA (2005)

  36. Lazarsfeld, R., van de Ven, A.: Topics in the geometry of projective space, Recent work by Zak, F.L., DMV Seminar 4, Birkhäuser, Germany, (1984)

  37. Mella, M., Russo, F.: Special Cremona transformations whose base locus has dimension at most three, preprint (2005)

  38. Mori S.: Projective manifolds with ample tangent bundle. Ann. Math. 110, 593–606 (1979)

    Article  Google Scholar 

  39. Mukai S.: Biregular classification of Fano threefolds and Fano manifolds of coindex 3. Proc. Nat. Acad. Sci. USA 86, 3000–3002 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  40. Ohno M.: On degenerate secant varieties whose Gauss map have the largest image. Pac. J. Math. 187, 151–175 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  41. Russo, F.: Notes for the Trento School “Geometry of Special Varieties”, preprint 2007 available at http://www.dmi.unict.it/~frusso/Note_di_Corso_files/GeometrySpecialVarieties.pdf

  42. Scorza, G.: Sulla determinazione delle varietá a tre dimensioni di S r (r  ≥  7) i cui S 3 tangenti si tagliano a due a due, Rend. Circ. Mat. Palermo 25 (1908), 193–204 (contained also in G. Scorza, Opere Scelte, vol. I, 167–182, Ed. Cremonese, Italy) (1960)

  43. Scorza, G.: Sulle varietá a quattro dimensioni di S r (r  ≥  9) i cui S 4 tangenti si tagliano a due a due, Rend. Circ. Mat. Palermo 27 (1909),148–178; contained also in G. Scorza, Opere Scelte, vol. I, 252–292, Ed. Cremonese, Italy (1960)

  44. Semple, J.G., Roth, L.: Introduction to Algebraic Geometry, Oxford Univ. Press, England, 1949, 1986

  45. Semple J.G., Tyrrell J.A.: The Cremona transformation of S 6 by quadrics through a normal elliptic septimic scroll 1 R 7. Mathematika 16, 88–97 (1969)

    Article  MathSciNet  Google Scholar 

  46. Semple J.G., Tyrrell J.A.: The T 2,4 of S 6 defined by a rational surface 3 F 8. Proc. Lond. Math. Soc. 20, 205–221 (1970)

    Article  MATH  MathSciNet  Google Scholar 

  47. Severi F.: Intorno ai punti doppi impropri di una superficie generale dello spazio a quattro dimensioni e ai suoi punti tripli apparenti. Rend. Circ. Mat. Palermo 15, 33–51 (1901)

    Article  MATH  Google Scholar 

  48. Terracini A.: Sulle V k per cui la varieta’ degli S h (h + 1)-secanti ha dimensione minore dell’ ordinario. Rend. Circ. Mat. Palermo 31, 392–396 (1911)

    Article  MATH  Google Scholar 

  49. Vermeire P.: Some results on secant varieties leading to a geometric flip construction. Comp. Math. 125, 263–282 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  50. Weyl H.: Emmy Noether. Scr. Math. 3, 201–220 (1935)

    Google Scholar 

  51. Zak F.L.: Severi varieties. Math. USSR Sb. 54, 113–127 (1986)

    Article  MATH  Google Scholar 

  52. Zak, F.L.: Tangents and secants of algebraic varieties, Transl. Math. Monogr. vol. 127. American Mathematical Society, USA (1993)

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica e Informatica, Università degli Studi di Catania, Viale A. Doria 6, 95125, Catania, Italy

    Francesco Russo

Authors
  1. Francesco Russo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Francesco Russo.

Additional information

Partially supported by CNPq (Centro Nacional de Pesquisa), grant 308745/2006-0, and by PRONEX/FAPERJ–Algebra Comutativa e Geometria Algebrica.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Russo, F. Varieties with quadratic entry locus, I . Math. Ann. 344, 597–617 (2009). https://doi.org/10.1007/s00208-008-0318-0

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00208-008-0318-0

Keywords

Search

Navigation