Skip to main content
SpringerLink
Log in

Dynamic Stability of Equilibrium Capillary Drops

  • Published:
Archive for Rational Mechanics and Analysis Aims and scope Submit manuscript

Abstract

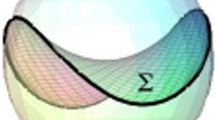
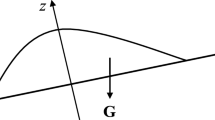
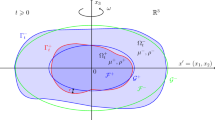
We investigate a model for contact angle motion of quasi-static capillary drops resting on a horizontal plane. We prove global in time existence and long time behavior (convergence to equilibrium) in a class of star-shaped initial data for which we show that topological changes of drops can be ruled out for all times. Our result applies to any drop which is initially star-shaped with respect to a small ball inside the drop, given that the volume of the drop is sufficiently large. For the analysis, we combine geometric arguments based on the moving-plane type method with energy dissipation methods based on the formal gradient flow structure of the problem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Aftalion A., Busca J., Reichel W.: Approximate radial symmetry for overdetermined boundary value problems. Adv. Differ. Equ. 4(6), 907–932 (1999)

    MATH  MathSciNet  Google Scholar 

  2. Alberti G., DeSimone A.: Quasistatic evolution of sessile drops and contact angle hysteresis. Archive Ration. Mech. Anal. 202(1), 295–348 (2011)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  3. Alexandrov A.D.: A characteristic property of spheres. Annali di Matematica Pura ed Applicata 58(1), 303–315 (1962)

    Article  MathSciNet  Google Scholar 

  4. Ambrosio, L., Gigli, N., Savaré G.: Gradient flows in metric spaces and in the space of probability measures. Lectures in Mathematics ETH Zürich, 2nd edn. Birkhäuser Verlag, Basel, 2008

  5. Bellettini, G., Caselles, V., Chambolle, A., Novaga, M.: The volume preserving crystalline mean curvature flow of convex sets in \({\mathbb{R}^N}\) . J. Math. Pures Appl. (9) 92(5), 499–527 (2009)

    Google Scholar 

  6. Berestycki H., Nirenberg L.: On the method of moving planes and the sliding method. Boletim da Sociedade Brasileira de Matemática-Bulletin/Brazilian Mathematical Society 22(1), 1–37 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  7. Blake, T.D., Ruschak, K.J.: Wetting: static and dynamic contact lines. Liquid film coating, 1 (1997)

  8. Brändle C., Vázquez J.L.: Viscosity solutions for quasilinear degenerate parabolic equations of porous medium type. Indiana Univ. Math. J. 54(3), 817–860 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  9. Brandolini B., Nitsch C., Salani P., Trombetti C.: On the stability of the Serrin problem. J. Differ. Equ. 245(6), 1566–1583 (2008)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  10. Brothers J.E., Ziemer W.P.: Minimal rearrangements of Sobolev functions. J. Reine Angew. Math. 384, 153–179 (1988)

    MATH  MathSciNet  Google Scholar 

  11. Caffarelli, L.A., Salsa, S.A.: Geometric Approach to Free Boundary Problems, vol. 68. American Mathematical Society, Providence, 2005

  12. Caffarelli, L.A., Vazquez, J.L.: Viscosity solutions for the porous medium equation. In: Differential Equations: La Pietra 1996, Proceeding of Symposia in Pure Mathematics, vol. 65. American Mathematical Society, Providence, 1999

  13. Chambolle A.: An algorithm for mean curvature motion. Interfaces Free Bound. 6(2), 195–218 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  14. Crandall M.G., Ishii H., Lions P.L.: Users guide to viscosity solutions of second order partial differential equations. Bull. Am. Math. Soc. 27(1), 1–67 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  15. De Silva, D.: Free boundary regularity for a problem with right hand side. ArXiv e-prints (0912.2057)

  16. Gidas B., Ni W.-M., Nirenberg L.: Symmetry and related properties via the maximum principle. Commun. Math. Phys. 68(3), 209–243 (1979)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  17. Glasner K., Kim I.C.: Viscosity solutions for a model of contact line motion. Interfaces Free Bound. 11(1), 37–60 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  18. Glasner K.B.: A boundary integral formulation of quasi-steady fluid wetting. J. Comput. Phys. 207(2), 529–541 (2005)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  19. Greenspan H.P.: On the motion of a small viscous droplet that wets a surface. J. Fluid Mech. 84(1), 125–143 (1978)

    Article  ADS  MATH  Google Scholar 

  20. Grunewald N., Kim I.: A variational approach to a quasi-static droplet model. Calculus Var. Partial Differ. Equ. 41, 1–19 (2011)

    Article  MATH  MathSciNet  Google Scholar 

  21. Hocking L.M., Miksis M.J.: Stability of a ridge of fluid. J. Fluid Mech. 247, 157–157 (1993)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  22. Jordan R., Kinderlehrer D., Otto F.: The variational formulation of the Fokker–Planck equation. SIAM J. Math. Anal. 29(1), 1–17 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  23. Kim I.C.: Uniqueness and existence results on the hele-shaw and the stefan problems. Arch. Ration. Mech. Anal. 168, 299–328 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  24. Kim, I.C., Pozár, N.: Nonlinear elliptic-parabolic problems. arXiv preprint arXiv:1203.2224, 2012

  25. Kinderlehrer D., Nirenberg L., Spruck J.: Regularity in elliptic free boundary problems i. J. d’Anal. Math. 34(1), 86–119 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  26. Luckhaus S., Sturzenhecker T.: Implicit time discretization for the mean curvature flow equation. Calc. Var. Partial Differential Equ. 3(2), 253–271 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  27. Mielke, A.: Modelling and analysis of rate-indepedent processes. Lipschitz Lectures (2007)

  28. Serrin J.: A symmetry problem in potential theory. Arch. Ration. Mech. Anal. 43, 304–318 (1971)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, UCLA, Los Angeles, USA

    William M. Feldman & Inwon C. Kim

Authors
  1. William M. Feldman
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Inwon C. Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to William M. Feldman.

Additional information

Communicated by D. Kinderlehrer

W.M. Feldman, and I.C. Kim have been partially supported by NSF DMS-0970072.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Feldman, W.M., Kim, I.C. Dynamic Stability of Equilibrium Capillary Drops. Arch Rational Mech Anal 211, 819–878 (2014). https://doi.org/10.1007/s00205-013-0698-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00205-013-0698-5

Keywords

Search

Navigation