Abstract
We define rationalizability for multicriteria games and examine its properties. In a multicriteria game, each agent can have multiple decision criteria and thus has a vector-valued utility function. An agent’s rationalizable action is defined as such an action that can survive iterated elimination of never-Pareto optimal actions. We first generalize some properties of standard rationalizability such as existence to the multicriteria case. We then show that a rationalizable action in some weighted game is also rationalizable in the original multicriteria game, whereas the converse may not hold. This implies the robustness of non-rationalizable actions under utility aggregations for any weight vectors. We also discuss interpretations of mixed actions and their implications to multicriteria games.
Similar content being viewed by others
Notes
Multicriteria games are also called multiobjective games or games with vector payoffs in the literature.
According to Zhao (2018), Most of the existing applications of multicriteria games are in industrial engineering and operations research.
Ok (2002) studied how an incomplete preference can be represented by a vector-valued utility function. In this context, Pareto equilibria of multicriteria games can be seen as Nash equilibria of games with incomplete preferences (Bade 2005). For a multicriteria game, if the agents can aggregate their own utilities by giving weights to the criteria, then the incompleteness is resolved and we obtain a standard game. (If they are uncertain about the weights, we would obtain a standard game with incomplete information.) However, as discussed by several authors (e.g.Aumann 1962; Bewley 2002; Ok 2002), there seems to be no a priori reason why an agent can always resolve the indecisiveness. Eliaz and Ok (2006) studied the choice-theoretic distinction between “indecisiveness” and “indifference”.
Both Bernheim (1984) and Pearce (1984) informally refer to common knowledge of rationality to explain intuitively the idea of rationalizability. They assume that the agents hold independent conjectures about the opponents’ choices. On the other hand, more recent studies on epistemic game theory consider that common knowledge of rationality allows the agents’ conjectures to be correlated. (An earlier formal analysis was done by Tan and Werlang (1988). See Perea (2012, Ch. 3) for a literature review of this topic.) This paper defines rationalizability per the original (Definition 3), but our results do not rely on the use of the independent version. Investigating potential implications led by the difference (independent or correlated) in multicriteria games is out of scope in this paper. However, it may be an interesting future work.
Of course these results depend on how to aggregate utilities. Our (as well as Shapley’s) analysis is based on a simple linear transformation used in many studies of multicriteria games, while there are other various ways of utility aggregation.
Studies on multicriteria games often deal with a stronger version of Pareto optimality, which is based on weak vector dominance, “p weakly dominates q if \(p_j \ge q_j\) for all \(j \in \{1, \ldots , m\}\) and \(p \ne q\).” This paper only discusses weak Pareto optimality. We would obtain similar definitions and results for the strong version.
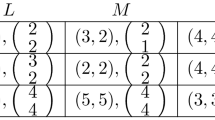
Consider the following example. For agent i, let \(A_i = \{a_1, a_2, a_3 \}\). For \(m_{-i} \in \varDelta A_{-i}\), let \(U_i (a_1, m_{-i}) = (3, 0), U_i (a_2, m_{-i}) = (2, 2)\) and \(U_i (a_3, m_{-i}) = (0, 3)\). In this case, every pure action is Pareto optimal for \(m_{-i}\). Consider i’s mixed action, say \(m_i\), that gives probability 1/2 to each of \(a_1\) and \(a_3\). Since \(U_i (m_i, m_{-i}) = (3/2, 3/2)\), it is not Pareto optimal for \(m_{-i}\).
Osborne and Rubinstein (1994, Ch. 3) point out that the notion of mixed-action equilibria cannot capture the agents’ motivation to introduce randomization, as a weak point of the view of mixed actions as objects of choice. The above discussion suggests that this critique does not hold generally for multicriteria games.
The observation here does not affect the idea that a multicriteria game can be seen as a game with incomplete preferences. Introducing mixed actions into a game with incomplete preferences essentially entails analyzing a multicriteria game with mixed extension (e.g. Bade 2005). Thus, even in a more general context, we face the same problem.
For the stronger version of Pareto optimality (see Footnote 7), the statement holds if we only consider positive weights, as shown by Shapley (1959).
References
Aumann RJ (1962) Utility theory without the completeness axiom. Econometrica 30(3):445–462
Bade S (2005) Nash equilibrium in games with incomplete preferences. Econ Theory 26(2):309–332
Battigalli P (1997) On rationalizability in extensive games. J Econ Theory 74(1):40–61
Bernheim D (1984) Rationalizable strategic behavior. Econometrica 52:1007–1028
Bewley TF (2002) Knightian decision theory. Part I. Decis Econ Financ 25(2):79–110
Blackwell D (1956) An analog of the minimax theorem for vector payoffs. Pac J Math 6(1):1–8
Borm P, van Megen F, Tijs S (1999) A perfectness concept for multicriteria games. Math Methods Oper Res 49(3):401–412
Borm P, Vermeulen D, Voorneveld M (2003) The structure of the set of equilibria for two person multicriteria games. Eur J Oper Res 148(3):480–493
De Marco G, Morgan J (2007) A refinement concept for equilibria in multicriteria games via stable scalarizations. Int Game Theory Rev 9(2):169–181
Eisenstadt E, Moshaiov A (2017) Novel solution approach for multi-objective attack-defense cyber games with unknown utilities of the opponent. IEEE Trans Emerg Topic Computat Intell 1(1):16–26
Eliaz K, Ok EA (2006) Indifference or indecisiveness? Choice-theoretic foundations of incomplete preferences. Game Econ Behav 56(1):61–86
Kada O (2004) Incomplete preference, iterated strict dominance and rationalizability. Unpublished manuscript
Kokkala J, Poropudas J, Virtanen K (2015) Rationalizable strategies in games with incomplete preferences. MRPA Paper No. 68331. https://mpra.ub.uni-muenchen.de/68331/
Ok EA (2002) Utility representation of an incomplete preference relation. J Econ Theory 104(2):429–449
Osborne MJ, Rubinstein A (1994) A course in game theory. MIT Press, Cambridge
Pearce DG (1984) Rationalizable strategic behavior and the problem of perfection. Econometrica 52:1029–1050
Perea A (2012) Epistemic game theory: reasoning and choice. Cambridge University Press, Cambridge
Rettieva A (2017) Equilibria in dynamic multicriteria games. Int Game Theory Rev 19:1750002
Shapley LS (1959) Equilibrium points in games with vector payoffs. Naval Res Log Q 6(1):57–61
Tan T, Werlang SRC (1988) The Bayesian foundations of solution concepts of games. J Econ Theory 45(2):370–391
Voorneveld M, Grahn S, Dufwenberg M (2000) Ideal equilibria in noncooperative multicriteria games. Math Methods Oper Res 52(1):65–77
Voorneveld M, Vermeulen D, Borm P (1999) Axiomatizations of Pareto equilibria in multicriteria games. Games Econ Behav 28(1):146–154
Zhao J (1991) The equilibria of a multiple objective game. Int J Game Theory 20(2):171–182
Zhao J (2018) Three little-known and yet still significant contributions of Lloyd Shapley. Games Econ Behav 108:592–599
Author information
Authors and Affiliations
Corresponding author
Additional information
The author greatly appreciates the reviewers’ helpful comments. This research was supported by JSPS KAKENHI Grant-in-Aid 15K16292.
Rights and permissions
About this article
Cite this article
Sasaki, Y. Rationalizability in multicriteria games. Int J Game Theory 48, 673–685 (2019). https://doi.org/10.1007/s00182-018-0655-5
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00182-018-0655-5