Abstract
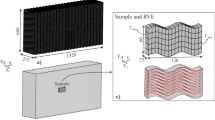
This work presents a variational homogenization approach based on representative volume elements (RVE) in order to investigate the macro- and microviscoelastic behavior of tendon fascicles. A three-dimensional hexagonal–helicoidal finite element RVE is proposed to properly account for the morphology of tendon fascicles observed in serial block-face scanning electron microscopy. Two material phases (collagen fibers and cells) comprising three finite strain variational viscoelastic models (fibrils, matrix of fibers and cells) compose the proposed multiscale model. The material parameters of the micromechanical models were identified with the aid of atomic force microscopy experiments extracted from the literature. A set of multiscale simulations of tensile tests under physiological strain amplitudes were performed, providing the following results. Firstly, numerical predictions corroborate experimental findings: collagen fibrils are the main load-bearing structures of tendons; the cellular matrix contributes neither to the stiffness nor to the energy dissipation of tendons. Secondly, the model brings insights about microscale mechanics of tendon fascicles not completely understood: prediction of uncoiling of fibers during axial loads which may explain the large apparent Poisson ratios and fluid loss, and significant strain localization in cells, which may lead to important mechanotransduction mechanisms. Moreover, the distribution of dissipated power became available, pointing out the fibrils as the main source of dissipation of fascicles under high macroscopic strain rates and during the unloading phase in cyclic regimes.
Similar content being viewed by others
References
Svensson, R.B., Hassenkam, T., Grant, C.A., Peter Magnusson, S.: Tensile properties of human collagen fibrils and fascicles are insensitive to environmental salts. Biophys. J. 99(12), 4020–4027 (2010). ISSN 00063495
Vergari, C., Pourcelot, P., Holden, L., Ravary-Plumioën, B., Gerard, G., Laugier, P., Mitton, D., Crevier-Denoix, N.: True stress and Poisson’s ratio of tendons during loading. J. Biomech. 44(4), 719–724 (2011). ISSN 00219290
Chernak, L.A., Thelen, D.G.: Tendon motion and strain patterns evaluated with two-dimensional ultrasound elastography. J. Biomech. 45(15), 2618–2623 (2012)
Reese, S.P., Weiss, J.: Tendon fascicles exhibit a linear correlation between poisson’s ratio and force during uniaxial stress relaxation. J. Biomech. Eng. 135(3), 34501 (2013). ISSN 1528-8951
Böl, M., Ehret, A.E., Leichsenring, K., Ernst, M.: Tissue-scale anisotropy and compressibility of tendon in semi-confined compression tests. J. Biomech. 48(6), 1092–1098 (2015)
Lynch, H.A., Johannessen, W., Wu, J.P., Jawa, A., Elliott, D.M.: Effect of fiber orientation and strain rate on nonlinear tendon tensile properties. J. Biomech. Eng. 125, 726–731 (2003)
Ahmadzadeh, H., Freedman, B.R., Connizzo, B.K., Soslowsky, L.J., Shenoy, V.B.: Micromechanical poroelastic finite element and shear-lag models of tendon predict large strain dependent Poisson’s ratios and fluid expulsion under tensile loading. Acta Biomaterialia 22, 83–91 (2014)
Kannus, P.: Structure of the tendon connective tissue. Scand. J. Med. Sci. Sports 10(6), 312–320 (2000)
Franchi, M., Trirè, A., Quaranta, M., Orsini, E., Ottani, V.: Collagen structure of tendon relates to function. TheScientificWorldJournal 7, 404–420 (2007)
Thorpe, C.T., Birch, H.L., Clegg, P.D., Screen, H.R.C.: Tendon physiology and mechanical behavior: structure-function relationships. In: Gomes, M.E., Reis, R.L., Rodrigues, M.T. (eds.) Tendon Regeneration: Understanding Tissue Physiology and Development to Engineer Functional Substitutes, Chap. 1, pp. 3–39. Elsevier Academic Press, Cambridge (2015)
Svensson, R.B., Hassenkam, T., Hansen, P., Peter Magnusson, S.: Viscoelastic behavior of discrete human collagen fibrils. J Mech Behav Biomed Mater 3(1), 112–115 (2010b)
Shen, Z.L., Kahn, H., Ballarini, R., Eppell, S.J.: Viscoelastic properties of isolated collagen fibrils. Biophys J 100(12), 3008–3015 (2011)
Yang, L., van der Werf, K.O., Dijkstra, P.J., Feijen, J., Bennink, M.L.: Micromechanical analysis of native and cross-linked collagen type I fibrils supports the existence of microfibrils. J Mech Behav Biomed Mater 6, 148–158 (2012)
Blanco, P.J., Sanchez, P.J., de Souza Neto, E.A., Feijo, R.A.: Variational Foundations and generalized unified theory of RVE-based multiscale models. Arch Comput Methods Eng 23(2), 191–253 (2014). ISSN 18861784
de Souza Neto, E., Blanco, P.J., Sánchez, P.J., Feijóo, R.: An RVE-based multiscale theory of solids with micro-scale inertia and body force effects. Mech Mater 80, 136–144 (2015)
Lanir, Y.: Multi-scale structural modeling of soft tissues mechanics and mechanobiology. J Elast 129(1–2), 7–48 (2017). https://doi.org/10.1007/s10659-016-9607-0. ISSN 15732681
Fang, F., Lake, S.P.: Modelling approaches for evaluating multiscale tendon mechanics. Interface Focus, 6 (2016)
Marino, M., Vairo, G.: Stress and strain localization in stretched collagenous tissues via a multiscale modelling approach. Comput. Methods Biomech. Biomed. Eng. 1–20 (2012)
Herchenhan, A., Kalson, N.S., Holmes, D.F., Hill, P., Kadler, K.E., Margetts, L.: Tenocyte contraction induces crimp formation in tendon-like tissue. Biomech. Model. Mechanobiol. 11(3–4), 449–459 (2012)
Fallah, A., Ahmadian, M.T., Firozbakhsh, K., Aghdam, M.M.: Micromechanics and constitutive modeling of connective soft tissues. J. Mech. Behav. Biomed. Mater. 60, 157–176 (2016). https://doi.org/10.1016/j.jmbbm.2015.12.029
Ganghoffer, J.F., Laurent, C., Maurice, G., Rahouadj, R., Wang, X.: Nonlinear viscous behavior of the tendon’s fascicles from the homogenization of viscoelastic collagen fibers. Eur. J. Mech. A/Solids 59, 265–279 (2016). https://doi.org/10.1016/j.euromechsol.2016.04.006
Ortiz, M., Stainier, L.: The variational formulation of viscoplastic constitutive updates. Comput. Methods Appl. Mech. Eng. 7825(98), 419–444 (1999)
Fancello, E., Ponthot, J.-P., Stainier, L.: A variational formulation of constitutive models and updates in non-linear finite viscoelasticity. Int. J. Numer. Methods Eng. 65(11), 1831–1864 (2006)
Mosler, J., Bruhns, O.T.: On the implementation of rate-independent standard dissipative solids at finite strain—Variational constitutive updates. Comput. Methods Appl. Mech. Eng. 199(9–12), 417–429 (2010)
Vassoler, J.M., Reips, L., Fancello, E.A.: A variational framework for fiber-reinforced viscoelastic soft tissues. Int. J. Numer. Methods Eng. 89(13), 1691–1706 (2012)
Kalson, N.S., Lu, Y., Taylor, S.H., Starborg, T., Holmes, D.F., Kadler, K.E.: A structure-based extracellular matrix expansion mechanism of fibrous tissue growth. eLife, 4 (2015). ISSN 2050-084X
Aifantis, K.E., Shrivastava, S., Odegard, G.M.: Transverse mechanical properties of collagen fibers from nanoindentation. J. Mater. Sci.: Mater. Med. 22(6), 1375–1381 (2011)
Haga, H., Sasaki, S., Kawabata, K., Ito, E., Ushiki, T., Sambongi, T.: Elasticity mapping of living fibroblasts by AFM and immunofluorescence observation of the cytoskeleton. Ultramicroscopy 82, 253–258 (2000). ISSN 03043991
Sirghi, L., Ponti, J., Broggi, F., Rossi, F.: Probing elasticity and adhesion of live cells by atomic force microscopy indentation. Eur. Biophys. J. 37(6), 935–945 (2008). ISSN 01757571
Raman, A., Trigueros, S., Cartagena, A., Stevenson, P.Z., Susilo, M., Nauman, E., Antoranz Contera, S.: Mapping nanomechanical properties of live cells using multi-harmonic atomic force microscopy. Nat. Nanotechnol. 6(12), 809–814 (2011)
Nawaz, S., Sánchez, P., Bodensiek, K., Li, S., Simons, M., Schaap, I.A.T.: Cell visco-elasticity measured with AFM and optical trapping at sub-micrometer deformations. PLoS ONE 7(9), (2012). ISSN 19326203
Hecht, F.M., Rheinlaender, J., Schierbaum, N., Goldmann, W.H., Fabry, B., Schäffer, T.E.: Imaging viscoelastic properties of live cells by AFM: power-law rheology on the nanoscale. Soft Matter 11(23), 4584–4591 (2015). ISSN 1744-6848
Thorpe, C.T., Udeze, C.P., Birch, H.L., Clegg, P.D., Screen, H.R.C.: Specialization of tendon mechanical properties results from interfascicular differences. J. R. Soc. Interface 9(July), 3108–3117 (2012)
de Aro, A.A., de Campos Vidal, B., Rosa Pimentel, E.: Biochemical and anisotropical properties of tendons. Micron 43(2–3), 205–214 (2012)
Starborg, T., Kalson, N.S., Lu, Y., Mironov, A., Cootes, T.F., Holmes, D.F., Kadler, K.E.: Using transmission electron microscopy and 3View to determine collagen fibril size and three-dimensional organization. Nat. Protoc. 8(7), 1433–48 (2013). ISSN 1750-2799
Provenzano, P.P., Vanderby, R.: Collagen fibril morphology and organization: implications for force transmission in ligament and tendon. Matrix Biol. 25(2), 71–84 (2006). ISSN 0945053X
Buehler, M.J.: Nature designs tough collagen: explaining the nanostructure of collagen fibrils. Proc. Nat. Acad. Sci. USA 103, 12285–12290 (2006)
Svensson, R.B., Mulder, H., Kovanen, V., Peter Magnusson, S.: Fracture mechanics of collagen fibrils: influence of natural cross-links. Biophys. J. 104(11), 2476–2484 (2013)
Gurtin, M.E., Anand, L.: The decomposition F = FeFp, material symmetry, and plastic irrotationality for solids that are isotropic-viscoplastic or amorphous. Int. J. Plast. 21(9), 1686–1719 (2005). ISSN 07496419
Nguyen, T.D., Jones, R.E., Boyce, B.L.: Modeling the anisotropic finite-deformation viscoelastic behavior of soft fiber-reinforced composites. Int. J. Solids Struct. 44, 8366–8389 (2007)
Schröder, J., Neff, P.: Invariant formulaiton of hyperelastic transverse isotropy based on polyconvex free energy functions. Int. J. Solids Struct. 40(2), 401–445 (2003). ISSN 00207683
Merodio, J., Ogden, R.W.: Mechanical response of fiber-reinforced incompressible non-linearly elastic solids. Int. J. Non-Linear Mech. 40(2–3), 213–227 (2005). ISSN 00207462
Ehret, A.E., Itskov, M.: A polyconvex hyperelastic model for fiber-reinforced materials in application to soft tissues. J. Mater. Sci. 42(21), 8853–8863 (2007). ISSN 00222461
Holzapfel, G., Ogden, R.W.: Constitutive modelling of passive myocardium: a structurally based framework for material characterization. Philos. Trans. Ser. A Math. Phys. Eng. Sci. 367(1902), 3445–75 (2009). ISSN 1364-503X
Balzani, D., Neff, P., Schröder, J., Holzapfel, G.: A polyconvex framework for soft biological tissues. Adjustment to experimental data. Int. J. Solids Struct. 43(20), 6052–6070 (2006). ISSN 00207683
de Souza Neto, E.A., Peric, D., Owen, D.R.J.: Computational methods for plasticity: Theory Appl. (2009)
Gurtin, M.E., Fried, E., Anand, L.: The Mechanics and Thermodynamics of Continua. Cambridge University Press, Cambridge (2010)
Carniel, T.A., Fancello, E.A.: Modeling the local viscoelastic behavior of living cells under nanoindentation tests. Latin Am. J. Solids Struct. 1–19 (2017)
Vaz Jr., M., Cardoso, E.L., Stahlschmidt, J.: Particle swarm optimization and identification of inelastic material parameters. Eng. Comput. 30(7), 936–960 (2013). ISSN 0264-4401
Screen, H.R.: Hierarchical approaches to understanding tendon mechanics. J. Biomech. Sci. Eng. 4(4), 481–499 (2009)
Svensson, R.B., Herchenhan, A., Starborg, T., Larsen, M., Kadler, K.E., Qvortrup, K., Peter M.S.: Evidence of structurally continuous collagen fibrils in tendon. Acta Biomaterialia, 1–9 (2017)
Nguyen, V.D., Béchet, E., Geuzaine, C., Noels, L.: Imposing periodic boundary condition on arbitrary meshes by polynomial interpolation. Comput. Mater. Sci. 55, 390–406 (2012). ISSN 09270256
Hansen, K., Weiss, J., Barton, J.K.: Imposing periodic boundary condition on arbitrary meshes by polynomial interpolation. J. Biomech. Eng. 124(1), 72–77 (2002). ISSN 01480731
Connizzo, B.K., Yannascoli, S.M., Soslowsky, L.J.: Structure–function relationships of postnatal tendon development: a parallel to healing. Matrix Biol. 32(2), 106–116 (2013)
Cheng, V.W.T., Screen, H.R.C.: The micro-structural strain response of tendon. J. Mater. Sci. 42(21), 8957–8965 (2007). ISSN 00222461
Goh, K.L., Holmes, D.F., Lu, H.-Y., Richardson, S., Kadler, K.E., Purslow, P.P., Wess, T.J.: Ageing changes in the tensile properties of tendons: influence of collagen fibril volume fraction. J. Biomech. Eng. 130(2), 021011 (2008). ISSN 01480731
Screen, H.R.C., Seto, J., Krauss, S., Boesecke, P., Gupta, H.S.: Extrafibrillar diffusion and intrafibrillar swelling at the nanoscale are associated with stress relaxation in the soft collagenous matrix tissue of tendons. Soft Matter 7(23), 11243–11251 (2011). ISSN 1744-683X
Szczesny, S.E., Elliott, D.M.: Incorporating plasticity of the interfibrillar matrix in shear lag models is necessary to replicate the multiscale mechanics of tendon fascicles. J. Mech. Behav. Biomed. Mater. 40, 325–338 (2014)
Legerlotz, K., Riley, G.P., Screen, H.R.C.: Specimen dimensions influence the measurement of material properties in tendon fascicles. J. Biomech. 43(12), 2274–2280 (2010)
Haraldsson, B.T., Aagaard, P., Krogsgaard, M., Alkjaer, M., Magnusson, S.P.: Regoin-specific mechanical properties of the human patella tendon. J. Appl. Physiol. 98, 1006–1007 (2005)
Akhtar, R., Schwarzer, N., Sherratt, M.J., Watson, R.E.B., Graham, H.K., Trafford, W., Mummery, P.M., Derby, B.: Nanoindentation of histological specimens: mapping the elastic properties of soft tissues. J. Mater. Res. 24(3), 638–646 (2009). ISSN 0884-2914
Hammer, N., Huster, D., Fritsch, S., Hädrich, C., Koch, H., Schmidt, P., Sichting, F., Franz Xaver Wagner, M., Boldt, A.: Do cells contribute to tendon and ligament biomechanics? PLoS ONE (2014). ISSN 19326203. https://doi.org/10.1371/journal.pone.0105037
Herbert, A., Brown, C., Rooney, P., Kearney, J., Ingham, E., Fisher, J.: Bi-linear mechanical property determination of acellular human patellar tendon grafts for use in anterior cruciate ligament replacement. J. Biomech. 49(9), 1607–1612 (2016). https://doi.org/10.1016/j.jbiomech.2016.03.041. ISSN 18732380
Depalle, B., Qin, Z., Shefelbine, S.J., Buehler, M.J.: Influence of cross-link structure, density and mechanical properties in the mesoscale deformation mechanisms of collagen fibrils. J. Mech. Behav. Biomed. Mater. 1–13 (2014)
Liu, Y., Ballarini, R., Eppell, S.J.: Tension tests on mammalian collagen fibrils. Interface Focus 6(1), 20150080 (2016). ISSN 2042-8898
Simo, J.C., Taylor, R.L.: Consistent tangent operators for rate-independent elastoplasticity. Comput. Methods Appl. Mech. Eng. 48(1), 101–118 (1985)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Andreas Öchsner.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Carniel, T.A., Fancello, E.A. A variational homogenization approach applied to the multiscale analysis of the viscoelastic behavior of tendon fascicles. Continuum Mech. Thermodyn. 31, 607–626 (2019). https://doi.org/10.1007/s00161-018-0714-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00161-018-0714-y