Skip to main content
SpringerLink
Log in

The flow of magnetohydrodynamic Maxwell nanofluid over a cylinder with Cattaneo–Christov heat flux model

  • Original Article
  • Published:
Continuum Mechanics and Thermodynamics Aims and scope Submit manuscript

Abstract

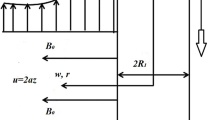
A theoretical analysis is performed for studying the flow and heat and mass transfer characteristics of Maxwell fluid over a cylinder with Cattaneo–Christov and non-uniform heat source/sink. The Brownian motion and thermophoresis parameters also considered into account. Numerical solutions are carried out by using Runge–Kutta-based shooting technique. The effects of various governing parameters on the flow and temperature profiles are demonstrated graphically. We also computed the friction factor coefficient, local Nusselt and Sherwood numbers for the permeable and impermeable flow over a cylinder cases. It is found that the rising values of Biot number, non-uniform heat source/sink and thermophoresis parameters reduce the rate of heat transfer. It is also found that the friction factor coefficient is high in impermeable flow over a cylinder case when compared with the permeable flow over a cylinder case.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Dhahir, S.A.: On non-Newtonian flow past a cylinder in a confined flow. J. Rheol. 33, 781 (1999). doi:10.1122/1.550074

    Article  ADS  Google Scholar 

  2. Martin, M.J., Boyd, I.D.: Momentum and heat transfer in a laminar boundary layer with slip flow. J. Thermophys. Heat Transf. 20, 710–719 (2006). doi:10.2514/1.22968

    Article  Google Scholar 

  3. Hayat, T., Abbas, Z., Sajid, M.: Series solution for the upper-convected Maxwell fluid over a porous stretching plate. Phys. Lett. Sect. A Gen. Atomic Solid State Phys. 358, 396–403 (2006). doi:10.1016/j.physleta.2006.04.117

    MATH  Google Scholar 

  4. Rashidi, M.M., Beg, O.A., Mehr, N.F., Hosseini, A., Gorla, R.S.R.: Homotopy simulation of axisymmetric laminar mixed convection nanofluid boundary layer flow. Theor. Appl. Mech. 39, 365–390 (2012)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Noor, N.F.M.: Analysis for MHD flow of a Maxwell fluid past a vertical stretching sheet in the presence of thermophoresis and chemical reaction. World Acad. Sci. Eng. Technol. 64, 1019–1023 (2012)

    Google Scholar 

  6. Hayat, T., Abbas, Z., Sajid, M.: MHD stagnation-point flow of an upper-convected Maxwell fluid over a stretching surface. Chaos Solitons Fractals 39, 840–848 (2009). doi:10.1016/j.chaos.2007.01.067

    Article  ADS  MATH  Google Scholar 

  7. Shateyi, S.: A new numerical approach to MHD flow of a Maxwell fluid past a vertical stretching sheet in the presence of thermophoresis and chemical reaction. Bound. Value Probl. 196, 1–14 (2013). doi:10.1186/1687-2770-2013-196

    MathSciNet  MATH  Google Scholar 

  8. Anika, N.N., Hoque, M.M., Islam, N.: Hall current effects on magnetohydrodynamic fluid over an infinite rotating vertical porous plate embedded in unsteady laminar flow. Ann. Pure Appl. Math. 3, 189–200 (2013)

    Google Scholar 

  9. Sajid, M., Abbas, Z., Ali, N., Javed, T., Ahmad, I.: Slip flow of a Maxwell fluid past a stretching sheet. Walailak J. Sci. Technol. 11, 1093–1103 (2014)

    Google Scholar 

  10. Halim, N.A., Noor, N.F.M.: Analytical solution for Maxwell nanofluid boundary layer flow over a stretching surface. In: The 22nd National Symposium on Mathematical Sciences (SKSM22): Strengthening Research and Collaboration of Mathematical Sciences in Malaysia. Vol. 1682. AIP Publishing (2015)

  11. Sheikholeslami, M., Mustafa, M.T., Ganji, D.D.: Nanofluid flow and heat transfer over a stretching porous cylinder considering thermal radiation. Iran. J. Sci. Technol. 39A3(Special issue), 433–440 (2015)

  12. Raju, C.S.K., Sandeep, N., JayachandraBabu, M.: Stagnation point flow towards horizontal and exponentially stretching/shrinking cylinders. J. Adv. Phys. 5(3), 207–213 (2016)

    Article  Google Scholar 

  13. Cattaneo, C.: Sullaconduzionedelcalore. AttidelSeminario Matematicoe Fisico dell Universitadi Modenae Reggio Emilia 3, 83–101 (1948)

    Google Scholar 

  14. Christov, C.I.: On frame in different formulation of the Maxwell–Cattaneo model of finite-speed heat conduction. Mech. Res. Commun. 36, 481–486 (2009)

    Article  MATH  Google Scholar 

  15. Straughan, B.: Thermal convection with the Cattaneo–Christov model. Int. J. Heat Mass Transf. 53, 95–98 (2010)

    Article  MATH  Google Scholar 

  16. Hayat, T., Imtiaz, M., Alsaedi, A., Almezal, S.: On Cattaneo–Christov heat flux in MHD flow of Oldroyd-B fluid with homogeneous-heterogeneous reactions. J. Mag. Mat. 401, 296–303 (2016)

    Article  ADS  Google Scholar 

  17. Mahapatra, T.R., Gupta, A.: Heat transfer in stagnation-point flow towards a stretching sheet. Heat Mass Transf. 38, 517–521 (2002)

    Article  ADS  Google Scholar 

  18. Pop, S., Grosan, T., Pop, I.: Radiation effects on the flow near the stagnation point of a stretching sheet. Tech. Mech. 25, 100–106 (2004)

    Google Scholar 

  19. Sharma, P., Singh, G.: Effects of variable thermal conductivity and heat source/sink on MHD flow near a stagnation point on a linearly stretching sheet. J. Appl. Fluid Mech. 2, 13–21 (2009)

    Google Scholar 

  20. Dinarvand, S., Abbassi, A., Hosseini, R., Pop, I.: Homotopy analysis method for mixed convective boundary layer flow of a nanofluid over a vertical circular cylinder. Therm. Sci. 9, 549–561 (2015). doi:10.2298/TSCI120225165D

    Article  Google Scholar 

  21. Ibrahim, S.M., Gangadhar, K., Bhaskar Reddy, N.: Radiation and mass transfer effects on MHD oscillatory flow in a channel filled with porous medium in the presence of chemical reaction. J. Appl. Fluid Mech. 8(3), 529–537 (2015)

    Article  Google Scholar 

  22. Raju, C.S.K., Sandeep, N.: Heat and mass transfer in 3D non-Newtonian nano and Ferro fluids over a bidirectional stretching surface. Int. J. Eng. Res. Afr. 21, 33–51 (2016)

    Article  Google Scholar 

  23. Malik, M.Y., Hussain, A., Salahuddin, T., Awais, M., Bilal, S.: Magnetohydrodynamic flow of Sisko fluid over a stretching cylinder with variable thermal conductivity: a numerical study. AIP Adv. 6, 025316 (2016). doi:10.1063/1.4942476

    Article  ADS  Google Scholar 

  24. Rashad, A.M., Mallikarjuna, B., Chamkha, A.J., Hariprasad Raju, S.: Thermophoresis effect on heat and mass transfer from a rotating cone in a porous medium with thermal radiation. Afrika Matematika 27(7), 1409–1424 (2016)

  25. Raju, C.S.K., Sandeep, N., Malvandi, A.: Free convective heat and mass transfer of MHD non-Newtonian nanofluids over a cone in the presence of non-uniform heat source/sink. J. Mol. Liquids 221, 108–115 (2016)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Garden City University, Bangalore, Karnataka, 560049, India

    C. S. K. Raju

  2. School of Computer Science and Engineering, VIT University, Vellore, 632014, India

    P. Sanjeevi

  3. Department of Mathematics, Annamacharya Institute of Science and Technology, Rajampet, 516126, Andhra Pradesh, India

    M. C. Raju

  4. Dept. of Mathematics, GITAM University, Visakhapatnam, 530045, India

    S. M. Ibrahim

  5. Department of Engineering and Architecture, University of Parma, Parco Area delle Scienze 181/A, 43124, Parma, Italy

    G. Lorenzini

  6. Department of Industrial Engineering, Alma Mater Studiorum-University of Bologna, viale Risorgimento no. 2, 40136, Bologna, Italy

    E. Lorenzini

Authors
  1. C. S. K. Raju
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. Sanjeevi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. C. Raju
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. S. M. Ibrahim
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. G. Lorenzini
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. E. Lorenzini
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. Lorenzini.

Additional information

Communicated by Andreas Öchsner.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Raju, C.S.K., Sanjeevi, P., Raju, M.C. et al. The flow of magnetohydrodynamic Maxwell nanofluid over a cylinder with Cattaneo–Christov heat flux model. Continuum Mech. Thermodyn. 29, 1347–1363 (2017). https://doi.org/10.1007/s00161-017-0580-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00161-017-0580-z

Keywords

Search

Navigation