Skip to main content
SpringerLink
Log in

Fundamental equations of certain electromagnetic-acoustic discontinuous fields in variational form

  • Original article
  • Published:
Continuum Mechanics and Thermodynamics Aims and scope Submit manuscript

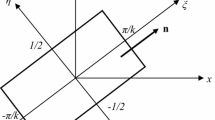
Abstract.

We present, in the first part of the paper, the well-known fundamental electromagnetic-acoustic equations, that is, the coupled Maxwell’s and Newton’s equations for an elastic dielectric continuum in differential form, and we also discuss the uniqueness of their linear solutions. In the second part, from a general principle of physics, we deduce a three-field variational principle that operates on the mechanical displacements, the electric potential, and the electromagnetic vector potential of the dielectric continuum. Then, we extend it through an involutory (or Friedrichs’s) transformation in deriving a nine-field unified variational principle that operates on the mechanical, electrical, and magnetic continuous linear fields under the infinitesimal strains. This variational principle generates Maxwell’s and Newton’s equations, the coupled linear constitutive relations, and the associated natural boundary conditions for the regular region of the dielectric continuum as its Euler-Lagrange equations. In the third part, we further generalise the unified variational principle so as to incorporate the jump conditions across a surface of discontinuity within the dielectric region. We also show that the integral and differential types of variational principles that apply to the linear motions of the elastic dielectric region with a fixed internal surface of discontinuity are in agreement with and recover, as special cases, some of the earlier variational principles. Further, the variational principles may be directly used in linear electromagnetic and/or acoustic field computations and in consistently establishing the lower order one- or two-dimensional equations of the elastic dielectric continuum.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Maugin, G.A.: Continuum Mechanics of Electromagnetic Solids, North Holland New York (1988)

  2. Rumsey, V.H.: Reaction concept in electromagnetic theory. Phys. Rev. 94, 483-491 (1954)

    Article  Google Scholar 

  3. Tonti, E.: Variational principles in electromagnetism. Rend. Ist. Lombardo A-102, 845-861 (1968)

    Google Scholar 

  4. Gurtin, M.E.: Variational principles in the linear theory of viscoelasticity. Arch. Rational Mech. Anal. 13, 179-191 (1963)

    MATH  Google Scholar 

  5. Tonti, E.: On the variational formulation for linear initial value problems. Annali di Matem. Pura Appl. 95, 331-359 (1973)

    MATH  Google Scholar 

  6. Harrington, R.F.: Field Computation by Moment Methods, Krieger New York (1968)

  7. Anderson, N., Arthurs, A.M.: A variational principle for Maxwell’s equations. Int. J. Electron. 45, 333-334 (1978)

    MathSciNet  Google Scholar 

  8. Herrera, I.: Variational principles for problems with linear constraints: prescribed jumps and continuation type restrictions. J. Inst. Math. Appl. 25, 67-96 (1980)

    MathSciNet  MATH  Google Scholar 

  9. Toupin, R.A.: The elastic dielectric. J. Rational Mech. Anal. 5, 849-915 (1956)

    MathSciNet  MATH  Google Scholar 

  10. Daher, N., Maugin, G.A.: Virtual power and thermodynamics for electromagnetic continua with interfaces. J. Math. Phys. 27, 3022-3035 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  11. Berk, A.D.: Variational principle for electromagnetic resonators and waveguides. IRE Trans. Antennas Propag. AP-4, 104-110 (1956)

    Google Scholar 

  12. Panofsky, W.K.H., Phillips, M.: Classical Electricity and Magnetism, 2nd edn., Addison Wesley London (1962)

  13. Nelson, D.F., Chen, B.: Lagrangian treatment of magnetic dielectrics. Phys. Rev. B 50, 1023-1038 (1994)

    Article  Google Scholar 

  14. Silvester, P.P., Pelosi, G. (Eds.): Finite Elements for Wave Electromagnetics: Methods and Techniques, IEEE Press New York (1994)

  15. Papastavridis, J.G.: A panoramic view of the principles and equations of motion of advanced engineering dynamics. Appl. Mech. Rev. 51, 239-265 (1998)

    Google Scholar 

  16. Oden, J.T., Reddy, J.N.: Variational Methods in Theoretical Mechanics, Springer Berlin (1976)

  17. English, W.J.: A computer-implemented vector variational solution of loaded rectangular waveguides. SIAM J. Appl. Math. 21, 461-468 (1971)

    Google Scholar 

  18. Jeng, S.K., Chen, C.H.: Variational finite element solution of electromagnetic wave propagation in a one-dimensional inhomogeneous anisotropic medium. J. Appl. Phys. 55, 630-636 (1984)

    Article  Google Scholar 

  19. Tao, L.N.: On variational principles for electromagnetic theory. J. Math. Phys. 7, 526 (1966)

    Google Scholar 

  20. Morosi, C.: Action principles for electromagnetism. Rend. Accad. Naz. Lincei, Serie VIII, LI, 502-510 (1971)

  21. Anderson, N., Arthurs, A.M.: Complementary variational principles for Maxwell’s equations. Int. J. Electron. 47, 229-236 (1979)

    MathSciNet  Google Scholar 

  22. Morishita, K., Kumagai, N.: Unified approach to derivation of variational expression for electromagnetic fields. IEEE Trans. MTT-25, 34-40 (1977)

    Google Scholar 

  23. Cendra, H., Holm, D.D., Hoyle, M.J.W., Marsden, J.E.: The Maxwell-Vlasov equations in Euler-Poincaré form. J. Math. Phys. 39, 3138-3157 (1988)

    Article  MATH  Google Scholar 

  24. Biehl, F.J.: Variational principles for general electromagnetic fields in nonmoving media. Arch. Electrotech. 70, 441-449 (1987)

    Google Scholar 

  25. Schuler, J., Felippa, C.A.: Electromagnetic finite elements based on a four potential variational principle. Finite Elem. Anal. Des. 6, 321-339 (1990)

    Article  MATH  Google Scholar 

  26. Mindlin, R.D.: Lecture Notes at Columbia University, New York (1968)

  27. Aşkar, G.A., Dökmeci, M.C.: Certain variational principles of mechanics of continua. BU&ITU R#07 (2000)

  28. Morishita, K., Kumagai, N.: Systematic derivation of variational expressions for electromagnetic and/or acoustic waves. IEEE Trans. MTT-26, 684-689 (1978)

    Google Scholar 

  29. Lee, P.C.Y.: A variational principle for the equations of piezoelectromagnetism in elastic dielectric crystals. J. Appl. Phys. 69, 7470-7473 (1991)

    Article  Google Scholar 

  30. Hadjigeorgiou, E.P., Kalpakides, V.K., Massalas, C.V.: A general theory for elastic dielectrics. II. the variational approach. Int. J. Non-linear Mech. 34, 967-980 (1999)

    Article  MATH  Google Scholar 

  31. Duvaut, G., Lions, P.L.: Inequations in Physics and Mechanics, Springer Berlin (1979)

  32. Auld, B.A.: Acoustic Fields and Waves in Solids, 2nd edn., Krieger New York (1990)

  33. Mason, W.P.: Crystal Physics of Interaction Processes, Academic Press New York (1966)

  34. Fichera, G.: Existence theorems in elasticity. In: Encyclopedia of Physics, vol. VIa/2, pp. 347-424, Springer Berlin Heidelberg New York (1972)

  35. Gurtin, M.E.: The linear theory of elasticity. In: Encyclopedia of Physics, vol. VIa/2, pp. 1-295, Springer Berlin Heidelberg New York (1972)

  36. Jones, D.S.: Methods in Electromagnetic Wave Propagation, Oxford University Press Oxford (1995)

  37. Santos, J.E., Sheen, D.: On the existence and uniqueness of solutions to Maxwell’s equations in bounded domains with application to magnetotellurics. Math. Models Methods Appl. Sci. 10, 615-628 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  38. Kirchhoff, G.: Über das Gleichgewicht und die Bewegung eines unendlich dünnen elastischen Stabes. J. Reine Angew. Math. 56, 285-313 (1859)

    Google Scholar 

  39. Neumann, F.: Vorlesungen über die Theorie der elastischen, der festen Körper und das Lichtathers, Teubner Leipzig (1885)

  40. Weiner, J.H.: A uniqueness theorem for the coupled thermoelastic problem. Q. Appl. Math. 15, 102-105 (1957)

    MathSciNet  MATH  Google Scholar 

  41. Aşkar Altay, G., Dökmeci, M.C.: A uniqueness theorem in Biot’s poroelasticity theory. Z. Angew Math. Phys. 49, 838-846 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  42. Knops, R.J., Payne, L.E.: Uniqueness theorems in linear elasticity. Springer Berlin (1972)

  43. Aşkar Altay, G., Dökmeci, M.C.: Coupled thermoelastic shell equations with second sound for high-frequency vibrations of temperature-dependent materials. Int. J. Solids Struct. 38 2737-2768 (2001)

  44. Morse, M., Feshbach, H.: Methods of Theoretical Physics, McGraw-Hill New York (1953)

  45. Biot, M.A.: New variational-Lagrangian irreversible thermodynamics with application to viscous flow, reaction-diffusion, and solid mechanics. In: Advances in Applied Mechanics, vol. 24, pp. 1-91, Academic Press New York (1984)

  46. Rosen, P.: Use of restricted variational principles for solution of differential equations. J. Appl. Phys. 25, 336-338 (1954)

    MathSciNet  MATH  Google Scholar 

  47. Friedrichs, K.O.: Ein Verfahren der Variationsrechung das Minimum eines Integrals als das Maximum eines anderen Ausdruckes darzustellen. Math. Phys. (Ges. Wiss. Göttingen, Nachrichten) 1, 13-20 (1929)

    Google Scholar 

  48. Fraeijs de Veubeke, B.M.: Dual principles of elastodynamics-finite element applications. In: Lecture on Finite Element Methods in Continuum Mechanics, pp. 357-377, University of Alabama Press Huntsville (1973)

  49. Dökmeci, M.C.: Certain integral and differential types of variational principles in nonlinear piezoelectricity. IEEE Trans. UFFC-35, 775-787 (1988)

    Google Scholar 

  50. Lanczos, C.: The Variational Principles of Mechanics, 4th edn., Dover New York (1986)

  51. Tiersten, H.F.: Natural boundary and initial conditions from a modification of Hamilton’s principle. J. Math. Phys. 9, 1445-1451 (1968)

    MATH  Google Scholar 

  52. Kellogg, O.D.: Foundations of Potential Theory, F. Ungar New York (1946)

  53. Christoffel, E.B.: Untersuchungen über die mit dem Fortbestehen linearer partieller. Differentialgleichungen vertraglichen Unstetigkeiten. Ann. Math. 8, 81-113 (1877)

    Google Scholar 

  54. Harrington, R.F.: Time-Harmonic Electromagnetic Fields, McGraw Hill New York (1961)

  55. Tiersten, H.F.: Linear Piezoelectric Plate Vibrations, Plenum Press New York (1969)

  56. He, J.-H.: Coupled variational principles of piezoelectricity. Int. J. Eng. Sci. 39, 323-341 (2001)

    Article  MathSciNet  Google Scholar 

  57. Cannarozzi, A.A., Ubertini, F.: Some hybrid variational methods for linear electroelasticity problems. Int. J. Solids Struct. 38, 2573-2596 (2001)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Engineering, Boğaziçi University, 34342, Istanbul, Turkey

    G. A. Altay

  2. Istanbul Technical University, P.K.9, Gümüşuyu, 34430, Istanbul, Turkey

    M. C. Dökmeci

Authors
  1. G. A. Altay
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. C. Dökmeci
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. A. Altay.

Additional information

Communicated by K. Hutter

Received: 9 January 2002, Accepted: 26 May 2003, Published online: 5 December 2003

PACS:

03.40, 41.10, 77.60

Correspondence to: G.A. Altay

Rights and permissions

Reprints and permissions

About this article

Cite this article

Altay, G.A., Dökmeci, M.C. Fundamental equations of certain electromagnetic-acoustic discontinuous fields in variational form. Continuum Mech. Thermodyn. 16, 53–71 (2004). https://doi.org/10.1007/s00161-003-0141-5

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00161-003-0141-5

Keywords:

Search

Navigation