Life expectancy, money, and growth

Abstract

We examine the effect of an increase in life expectancy on portfolio choices of individuals and, thereby, on economic growth in a simple endogenous growth model populated by overlapping generations, in which money is introduced based on the money-in-the-utility-function approach. It is shown that an increase in longevity raises the balanced growth rate and lowers the inflation rate, offsetting the Tobin effect, if spillovers from accumulated capital to labor productivity sufficiently raise wage income and real savings, and, if not, it may retard economic growth and aggravate inflation. Under plausible conditions, the former will be the case.

Appendices

Uniqueness of balanced growth equilibrium

From Eq. (18),

$$\phi {\left( \pi \right)} \equiv {\left( {1 + \pi } \right)}\frac{\omega }{a}{\left[ {{\left( {1 + r} \right)}{\left( {1 + \pi } \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)} - {\left( {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}} \right]}$$

and

$$\varepsilon {\left( \pi \right)} \equiv {\left( {1 + \mu } \right)}{\left\{ {{\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right]}{\left( {1 + r} \right)}{\left( {1 + \pi } \right)} - 1} \right\}}$$

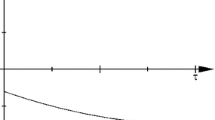
as depicted in Fig. 1. ϕ(π) is a quadratic function of π and has two intersections with the horizontal axis at −1 and ${\left\{ {{{\left[ {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}} \mathord{\left/ {\vphantom {{{\left[ {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}} {{\left[ {{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}} \right]}}}} \right. \kern-\nulldelimiterspace} {{\left[ {{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}} \right]}}} \right\}} - 1$, while ɛ(π) is a line and intersects the abscissa at ${\left\{ {{\left( {1 + r} \right)}{\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right]}} \right\}}^{{ - 1}} - 1$. Since we can easily see that ${\left\{ {{{\left[ {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}} \mathord{\left/ {\vphantom {{{\left[ {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}} {{\left[ {{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}} \right]}}}} \right. \kern-\nulldelimiterspace} {{\left[ {{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}} \right]}}} \right\}} - 1 > {\left\{ {{\left( {1 + r} \right)}{\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right]}} \right\}}^{{ - 1}} - 1$ for λ, η ∈ (0,1), Eq. (18) has two real roots, $\pi _{{\ell }}$ and π _h , such that $- 1 < \pi _{{\ell }} < {\left\{ {{\left( {1 + r} \right)}{\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right]}} \right\}}^{{ - 1}} - 1 < \pi _{h}$ (see Fig. 1). With condition (19), however, the smaller root, $\pi _{{\ell }}$, is not a solution to our model. Therefore, solving Eq. (18) for π, we obtain

$$\pi = \frac{{\frac{\omega }{a}{\left( {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)} + {\left( {1 + \mu } \right)}{\left( {1 + r} \right)}{\left\{ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right\}} + D^{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}} }}{{2\frac{\omega }{a}{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}}} - 1$$

where

$$D = {\left[ {\frac{\omega }{a}{\left( {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)} + {\left( {1 + \mu } \right)}{\left( {1 + r} \right)}{\left\{ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right\}}} \right]}^{2} - 4\frac{\omega }{a}{\left( {1 + \mu } \right)}{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}$$

from which it follows that

$${\left( {1 + \pi } \right)}{\left( {1 + r} \right)}\frac{{2\omega }}{a}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)} - {\left[ {\frac{\omega }{a}{\left( {1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)} + {\left( {1 + \mu } \right)}{\left( {1 + r} \right)}{\left\{ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}\frac{\mu }{{1 + \mu }}} \right\}}} \right]} > 0$$

This implies that $\frac{{\partial \varepsilon }}{{\partial \pi }} - \frac{{\partial \phi }}{{\partial \pi }} > 0$. Thus, we have $sign{\left( {\frac{{d\pi }}{{d\lambda }}} \right)} = sign{\left( {\frac{{\partial \phi }}{{\partial \lambda }} - \frac{{\partial \varepsilon }}{{\partial \lambda }}} \right)}.$

Increase in the growth rate of money supply

Differentiating ɛ(π; μ), we have

$$\frac{{\partial \varepsilon }}{{\partial \mu }} = {\left( {1 + r} \right)}{\left( {1 + \pi } \right)}{\left\{ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right\}} - 1$$

from which it follows that

$$sign{\left( {\frac{{\partial \varepsilon }}{{\partial \mu }}} \right)} = sign{\left[ {\pi - {\left\{ {{\left( {1 + r} \right)}^{{ - 1}} {\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}^{{ - 1}} - 1} \right\}}} \right]}$$

Since we can easily see that

$$\frac{{1 - \eta \frac{{1 + \rho }}{{2 + \rho - \lambda }}}}{{{\left( {1 + r} \right)}{\left( {1 - \frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right)}}} - 1 > \frac{1}{{{\left( {1 + r} \right)}{\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}}} - 1$$

while taking Eq. (19) into account, it follows that

$$\pi - {\left\{ {{\left( {1 + r} \right)}^{{ - 1}} {\left[ {1 - {\left( {1 - \eta } \right)}\frac{{1 + \rho }}{{2 + \rho - \lambda }}} \right]}^{{ - 1}} - 1} \right\}} > 0$$

The curve ϕ(π) does not depend on μ, so the upward shift of ɛ(π, μ) increases the inflation rate.