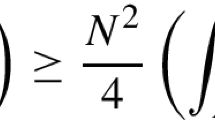Abstract
Breitenberger’s uncertainty principle on the torus \(\mathbb {T}\) and its higher-dimensional analogue on \(\mathbb {S}^{d-1}\) are well understood. We describe an entire family of uncertainty principles on compact manifolds \((M,g)\), which includes the classical Heisenberg–Weyl uncertainty principle (for \(M=B(0,1) \subset \mathbb {R}^d\) the unit ball with the flat metric) and the Goh–Goodman uncertainty principle (for \(M=\mathbb {S}^{d-1}\) with the canonical metric) as special cases. This raises a new geometric problem related to small-curvature low-distortion embeddings: given a function \(f:M \rightarrow \mathbb {R}\), which uncertainty principle in our family yields the best result? We give a (far from optimal) answer for the torus, discuss disconnected manifolds and state a variety of other open problems.







Similar content being viewed by others
References
Breitenberger, E.: Uncertainty measures and uncertainty relations for angle observables. Found. Phys. 15(3), 353–364 (1985)
Clarkson, J.: Uniformly convex spaces. Trans. Am. Math. Soc. 40(3), 396–414 (1936)
Dai, F., Xu, Y.: Hardy-Rellich inequality and uncertainty principle on the unit sphere. Constr. Approx. 40, 141–171 (2014)
Erb, W.: Uncertainty principles on compact Riemannian manifolds. Appl. Comput. Harmon. Anal. 29(2), 182–197 (2010)
Folland, G., Sitaram, A.: The uncertainty principle: a mathematical survey. J. Fourier Anal. Appl. 3(3), 207–238 (1997)
Freeden, W., Windheuser, U.: Combined spherical harmonic and wavelet expansion—a future concept in Earth’s gravitational determination. Appl. Comput. Harmon. Anal. 4(1), 1–37 (1997)
Goh, S., Goodman, T.: Uncertainty principles and asymptotic behavior. Appl. Comput. Harmon. Anal. 16(1), 19–43 (2004)
Kombe, I., Özaydin, M.: Improved Hardy and Rellich inequalities on Riemannian manifolds. Trans. Am. Math. Soc. 361(12), 6191–6203 (2009)
Kombe, I., Özaydin, M.: Hardy-Poincaré, Rellich and uncertainty principle inequalities on Riemannian manifolds. Trans. Am. Math. Soc. 365(10), 5035–5050 (2013)
Lieb, E., Loss, M.: Analysis. Second edition. Graduate Studies in Mathematics, vol. 14. American Mathematical Society, Providence, RI (2001)
Narcowich, F., Ward, J.: Nonstationary wavelets on the m-sphere for scattered data. Appl. Comput. Harmon. Anal. 3(4), 324–336 (1996)
Prestin, J., Quak, W.: Optimal functions for a periodic uncertainty principle and multiresolution analysis. Proc. Edinburgh Math. Soc. 42(2), 225–242 (1999)
Prestin, J., Quak, E., Rauhut, H., Selig, K.: On the connection of uncertainty principles for functions on the circle and on the real line. J. Fourier Anal. Appl. 9(4), 387–409 (2003)
Rösler, M., Voit, M.: An uncertainty principle for ultraspherical expansions. J. Math. Anal. Appl. 209(2), 624–634 (1997)
Acknowledgments
The author was supported by SFB 1060 of the DFG.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Gerald B. Folland.
Rights and permissions
About this article
Cite this article
Steinerberger, S. An Uncertainty Principle on Compact Manifolds. J Fourier Anal Appl 21, 575–599 (2015). https://doi.org/10.1007/s00041-014-9382-x
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00041-014-9382-x