Skip to main content
SpringerLink
Log in

Finite-Time Passivity for Atangana–Baleanu–Caputo Fractional-Order Systems with Nonlinear Perturbations

  • Published:
Circuits, Systems, and Signal Processing Aims and scope Submit manuscript

Abstract

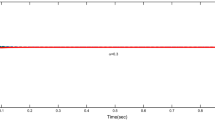
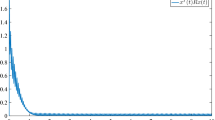
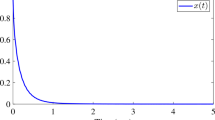
This paper investigates the problem of finite-time passivity for a class of nonlinear Atangana–Baleanu–Caputo (ABC) fractional-order systems (FOs). Firstly, a new definition of finite-time passivity for the FO nonlinear systems in the frame of the Atangana–Baleanu derivative of Caputo type is introduced. Then several sufficient conditions in the form of linear matrix inequalities (LMIs) are presented to guarantee that such the system is robustly finite-time passive. Finally, two numerical examples are given to verify the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Data Availability

All data generated or analyzed during this study are included in this article.

References

  1. K.A. Abro, A. Atangana, A comparative analysis of electromechanical model of piezoelectric actuator through Caputo Fabrizio and Atangana–Baleanu fractional derivatives. Math. Methods Appl. Sci. 43(17), 9681–9691 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  2. B.S.T. Alkahtani, Chua’s circuit model with Atangana Baleanu derivative with fractional order. Chaos Solitons Fractals 89, 547–551 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  3. A. Atangana, D. Baleanu, New fractional derivatives with nonlocal and non-singular kernel: theory and application to heat transfer model. Therm. Sci. 20(2), 763–769 (2016)

    Article  Google Scholar 

  4. A. Atangana, I. Koca, Chaos in a simple nonlinear system with Atangana–Baleanu derivatives with fractional order. Chaos Solitons Fractals 89, 447–454 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  5. D. Baleanu, A. Fernandez, On some new properties of fractional derivatives with Mittag–Leffler kernel. Commun. Nonlinear Sci. Numer. Simul. 59, 444–462 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  6. A. Ben Makhlouf, O. Naifar, M.A. Hammami, B.W. Wu, FTS and FTB of conformable fractional order linear systems. Math. Probl. Eng. 2018, Article ID 2572986 (2018)

  7. A. Ben Makhlouf, A.M. Nagy, Finite-time stability of linear Caputo–Katugampola fractional-order time delay systems. Asian J. Control 22(1), 297–306 (2020)

    Article  MathSciNet  Google Scholar 

  8. M. Caputo, M. Fabrizio, A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. 2, 73–85 (2015)

    Google Scholar 

  9. L. Chen, C. Liu, R. Wu, Y. He, Y. Chai, Finite-time stability criteria for a class of fractional-order neural networks with delay. Neural Comput. Appl. 27(3), 549–556 (2016)

    Article  Google Scholar 

  10. Y. Chen, L. Yang, A. Xue, Finite-time passivity of stochastic Markov jump neural networks with random distributed delays and sensor nonlinearities. Circuits Syst. Signal Process. 38(6), 2422–2444 (2019)

    Article  Google Scholar 

  11. I.K. Dassios, D.I. Baleanu, Caputo and related fractional derivatives in singular systems. Appl. Math. Comput. 337, 591–606 (2018)

    MathSciNet  MATH  Google Scholar 

  12. I.K. Dassios, G. Tzounas, F. Milano, Generalized fractional controller for singular systems of differential equations. J. Comput. Appl. Math. 378, 112919 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  13. F. Du, J.G. Lu, New approach to finite-time stability for fractional-order BAM neural networks with discrete and distributed delays. Chaos Solitons Fractals 151, 111225 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  14. F. Du, J.G. Lu, New results on finite-time stability of fractional-order Cohen–Grossberg neural networks with time delays. Asian J. Control (2021). https://doi.org/10.1002/asjc.2641

    Article  Google Scholar 

  15. W. Gao, B. Ghanbari, H.M. Baskonus, New numerical simulations for some real world problems with Atangana–Baleanu fractional derivative. Chaos Solitons Fractals 128, 34–43 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  16. C. Ge, J.H. Park, C. Hua, C. Shi, Robust passivity analysis for uncertain neural networks with discrete and distributed time-varying delays. Neurocomputing 364, 330–337 (2019)

    Article  Google Scholar 

  17. B. Ghanbari, A. Atangana, A new application of fractional Atangana–Baleanu derivatives: designing ABC-fractional masks in image processing. Physica A Stat. Mech. Appl. 542, 123516 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  18. A. Giusti, A comment on some new definitions of fractional derivative. Nonlinear Dyn. 93(3), 1757–1763 (2018)

    Article  MATH  Google Scholar 

  19. A. Gupta, S. Kumar, Design of Mittag–Leffler kernel-based fractional-order digital filter using fractional delay interpolation. Circuits Syst. Signal Process. 41(6), 3415–3445 (2022)

    Article  Google Scholar 

  20. D.T. Hong, N.H. Sau, M.V. Thuan, Output feedback finite-time dissipative control for uncertain nonlinear fractional-order systems. Asian J. Control (2021). https://doi.org/10.1002/asjc.2643

    Article  Google Scholar 

  21. A. Jmal, A.B. Makhlouf, A.M. Nagy, O. Naifar, Finite-time stability for Caputo–Katugampola fractional-order time-delayed neural networks. Neural Process. Lett. 50(1), 607–621 (2019)

    Article  Google Scholar 

  22. Y. Kao, Y. Li, J.H. Park, X. Chen, Mittag–Leffler synchronization of delayed fractional memristor neural networks via adaptive control. IEEE Trans. Neural Netw. Learn. Syst. 32(5), 2279–2284 (2020)

    Article  MathSciNet  Google Scholar 

  23. F. Kheyrinataj, A. Nazemi, Fractional Chebyshev functional link neural network-optimization method for solving delay fractional optimal control problems with Atangana-Baleanu derivative. Optim. Control Appl. Methods 41(3), 808–832 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  24. S. Kumar, D. Baleanu, Numerical solution of two-dimensional time fractional cable equation with Mittag–Leffler kernel. Math. Methods Appl. Sci. 43(15), 8348–8362 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  25. K.D. Kucche, S.T. Sutar, Analysis of nonlinear fractional differential equations involving Atangana–Baleanu–Caputo derivative. Chaos Solitons Fractals 143, 110556 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  26. H. Liu, X. Chen, J. Qiu, F. Zhao, Finite-time synchronization of complex networks with hybrid-coupled time-varying delay via event-triggered aperiodically intermittent pinning control. Math. Methods Appl. Sci. (2021). https://doi.org/10.1002/mma.7907

    Article  Google Scholar 

  27. U.N. Katugampola, A new approach to generalized fractional derivatives. Bull. Math. Anal. Appl. 6(4), 1–15 (2014)

    MathSciNet  MATH  Google Scholar 

  28. R. Khalil, M. Al Horani, A. Yousef, M. Sababheh, A new definition of fractional derivative. J. Comput. Appl. Math. 264, 65–70 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  29. Y.J. Ma, B.W. Wu, Y.E. Wang, Finite-time stability and finite-time boundedness of fractional order linear systems. Neurocomputing 173, 2076–2082 (2016)

    Article  Google Scholar 

  30. O. Martínez-Fuentes, G. Fernández-Anaya, A.J. Vázquez, Lyapunov functions for fractional-order nonlinear systems with Atangana–Baleanu derivative of Riemann–Liouville type. Math. Methods Appl. Sci. 44(18), 14206–14216 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  31. V.F. Morales-Delgado, J.F. Gómez-Aguilar, K. Saad, R.F. Escobar Jiménez, Application of the Caputo–Fabrizio and Atangana–Baleanu fractional derivatives to mathematical model of cancer chemotherapy effect. Math. Methods Appl. Sci. 42(2), 1167–1193 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  32. S. Rajavel, R. Samidurai, J. Cao, A. Alsaedi, B. Ahmad, Finite-time non-fragile passivity control for neural networks with time-varying delay. Appl. Math. Comput. 297, 145–158 (2017)

    MathSciNet  MATH  Google Scholar 

  33. N.H. Sau, M.V. Thuan, N.T.T. Huyen, Passivity analysis of fractional-order neural networks with time-varying delay based on LMI approach. Circuits Syst. Signal Process. 39(12), 5906–5925 (2020)

    Article  Google Scholar 

  34. N. Sene, K. Abdelmalek, Analysis of the fractional diffusion equations described by Atangana–Baleanu–Caputo fractional derivative. Chaos Solitons Fractals 127, 158–164 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  35. H. Shen, Y. Wang, J. Cao, X. Chen, Non-fragile mixed passive and $H_{\infty }$ state estimation for singularly perturbed neural networks with semi-Markov jumping parameters. J. Franklin Inst. 357(10), 6352–6369 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  36. J. Sheng, W. Jiang, D. Pang, Finite-time stability of Atangana–Baleanu fractional-order linear systems. Complexity 2020, Artical ID 1727358), 8 (2020)

  37. N.H. Sweilam, S.M. Al-Mekhlafi, T. Assiri, A. Atangana, Optimal control for cancer treatment mathematical model using Atangana–Baleanu–Caputo fractional derivative. Adv. Differ. Equ. 2020(1), 1–21 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  38. H. Tajadodi, A. Khan, J. Francisco Gómez-Aguilar, H. Khan, Optimal control problems with Atangana–Baleanu fractional derivative. Optim. Control Appl. Methods 42(1), 96–109 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  39. M.A. Taneco-Hernández, C. Vargas-De-Leon, Stability and Lyapunov functions for systems with Atangana–Baleanu Caputo derivative: an HIV/AIDS epidemic model. Chaos Solitons Fractals 132, 109586 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  40. G.S. Teodoro, J.T. Machado, E.C. De Oliveira, A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 388, 195–208 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  41. M.V. Thuan, D.C. Huong, D.T. Hong, New results on robust finite-time passivity for fractional-order neural networks with uncertainties. Neural Process. Lett. 50(2), 1065–1078 (2019)

    Article  Google Scholar 

  42. M.V. Thuan, T.N. Binh, D.C. Huong, Finite-time guaranteed cost control of Caputo fractional-order neural networks. Asian J. Control 22(2), 696–705 (2020)

    Article  MathSciNet  Google Scholar 

  43. G. Tzounas, I. Dassios, M.A.A. Murad, F. Milano, Theory and implementation of fractional order controllers for power system applications. IEEE Trans. Power Syst. 35(6), 4622–4631 (2020)

    Article  Google Scholar 

  44. S. Ullah, M.A. Khan, M. Farooq, Modeling and analysis of the fractional HBV model with Atangana–Baleanu derivative. The Eur. Phys. J. Plus 133(8), 1–18 (2018)

    Article  Google Scholar 

  45. H. Wang, J. Zhao, Finite-time passivity of switched non-linear systems. IET Control Theory Appl. 12(3), 338–345 (2018)

    Article  MathSciNet  Google Scholar 

  46. J.L. Wang, X.X. Zhang, H.N. Wu, T. Huang, Q. Wang, Finite-time passivity and synchronization of coupled reaction–diffusion neural networks with multiple weights. IEEE Trans. Cybern. 49(9), 3385–3397 (2018)

    Article  Google Scholar 

  47. C. Wang, X. Chen, J. Cao, J. Qiu, Y. Liu, Y. Luo, Neural network-based distributed adaptive pre-assigned finite-time consensus of multiple TCP/AQM networks. IEEE Trans. Circuits Syst. I Regul. Pap. 68(1), 387–395 (2020)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The author would like to thank the editor(s) and anonymous reviewers for their constructive comments which helped to improve the present paper. The research of Mai Viet Thuan is funded by Ministry of Education and Training of Vietnam (B2023-TNA). The research of Nguyen Truong Thanh is supported by the Hanoi University of Mining and Geology, Vietnam [T21-03].

Author information

Authors and Affiliations

  1. Faculty of Fundamental Science, Hanoi University of Industry, 298 Cau Dien street, Bac Tu Liem District, Hanoi, Vietnam

    Nguyen Huu Sau

  2. Department of Mathematics, University of Mining and Geology, Hanoi, Vietnam

    Nguyen Truong Thanh

  3. Department of Mathematics and Informatics, TNU–University of Sciences, Thainguyen, Vietnam

    Nguyen Thi Thanh Huyen & Mai Viet Thuan

Authors
  1. Nguyen Huu Sau
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nguyen Truong Thanh
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Nguyen Thi Thanh Huyen
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mai Viet Thuan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mai Viet Thuan.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sau, N.H., Thanh, N.T., Huyen, N.T.T. et al. Finite-Time Passivity for Atangana–Baleanu–Caputo Fractional-Order Systems with Nonlinear Perturbations. Circuits Syst Signal Process 41, 6774–6787 (2022). https://doi.org/10.1007/s00034-022-02135-y

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00034-022-02135-y

Keywords

Mathematics Subject Classification

Search

Navigation