Skip to main content
SpringerLink
Log in

Steady states of a diffusive Lotka–Volterra system with fear effects

  • Published:
Zeitschrift für angewandte Mathematik und Physik Aims and scope Submit manuscript

Abstract

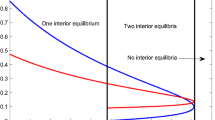
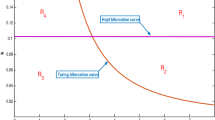
In this paper, we focus on the dynamical properties of a diffusive Lotka–Volterra system with fear effects subjected to the Neuman boundary condition in a bounded domain. The nonexistence of nonconstant solutions is obtained when diffusion rate is sufficiently large. We also establish the existence and multiplicity of the nonhomogeneous steady-state solutions bifurcating from the constant solution of the system by means of the Lyapunov–Schmidt reduction method. In addition, we consider the influence of the intrinsic growth rate on the population dynamics of the model and show that not only can the population density of both predator and prey change by changing the intrinsic growth rate, but also the coexistence equilibrium can possibly be destabilized. At the same time, we also consider the fear effect on the stability, we find that there is no obvious impact on the stability when the fear effect k is small. However, the results on stability will obviously change when the fear effect is large, which are very different from the ODE system (Wang et al. in J Math Biol 73:1179-1204, 2016) without the fear effect.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Cantrell, R.S., Cosner, C.: Spatial Ecology via Reaction–diffusion Equations. Wiley, New York (2003)

    MATH  Google Scholar 

  2. Cantrell, R.S., Cosner, C., Hutson, V.: Permanence in ecological systems with spatial heterogeneity. Proc. R. Soc. Edinb. Sect. A 123(3), 533–559 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chen, X., Hambrock, R., Lou, Y.: Evolution of conditional dispersal: a reaction–diffusion-advection model. J. Math. Biol. 57(3), 361–386 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  4. Chen, X.Y., Polavcik, P.: Gradient-like structure and Morse decompositions for time-periodic one-dimensional parabolic equations. J. Dyn. Differ. Equ. 7(1), 73–107 (1995)

    Article  MathSciNet  Google Scholar 

  5. Du, Y., Lou, Y.: Qualitative behaviour of positive solutions of a predator-prey model: effects of saturation. Proc. R. Soc. Edinb. Sect. A 131, 321–349 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  6. Gourley, S.A., Britton, N.F.: A predator–prey reaction–diffusion system with nonlocal effects. J. Math. Biol. 34, 297–333 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  7. Hale, J.K.: Asymptotic Behavior of Dissipative Systems. Math. Surveys Monogr., vol. 25, Amer. Math. Soc., Providence, RI (1988)

  8. Henry, D.: Geometric Theory of Semilinear Parabolic Equations. Lecture Notes in Math, vol. 840. Springer, Berlin (1981)

    Book  Google Scholar 

  9. Guo, S.J., Ma, L.: Stability and bifurcation in a delayed reaction–diffusion equation with Dirichlet boundary condition. J. Nonlinear Sci. 26(2), 545–580 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  10. Jiang, J., Shi, J.: Dynamics of a reaction–diffusion system of autocatalytic chemical reaction. Discrete Contin. Dyn. Syst. 21(1), 245–258 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  11. Lam, K.Y., Ni, W.M.: Uniqueness and complete dynamics in heterogeneous competition–diffusion systems. SIAM J. Appl. Math. 72(6), 1695–1712 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  12. Leung, A.: Limiting behaviour for a prey–predator model with diffusion and crowding effects. J. Math. Biol. 6, 87–93 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  13. Li, D., Guo, S.: Stability and Hopf bifurcation in a reaction–diffusion model with chemotaxis and nonlocal delay effect. Int. J. Bifurc. Chaos. 4(28), 1850046 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  14. Li, D., Guo, S.: Traveling wavefronts in a reaction–diffusion model with chemotaxis and nonlocal delay effect. Nonlinear Anal. Real World Appl. 45, 736–754 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  15. Li, D., He, X., Li, X., Guo, S.: Traveling wavefronts in a two-species chemotaxis model with Lotka–Volterra competitive kinetics. Appl. Math. Lett. 114, 106905 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  16. Ma, L., Gao, J.P., Li, D., Lian, W.Y.: Dynamics of a delayed Lotka–Volterra competition model with directed dispersal. Nonlinear Anal. Real World Appl. 71, 103830 (2023)

    Article  MathSciNet  Google Scholar 

  17. Ma, L., Guo, S.J.: Positive solutions in the competitive Lotka–Volterra reaction–diffusion model with advection terms. Proc. Am. Math. Soc. 149(7), 3013–3019 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  18. Ma, L., Guo, S.J.: Bifurcation and stability of a two-species reaction–diffusion–advection competition model. Nonlinear Anal. Real World Appl. 59, 103241 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  19. Ma, L., Feng, Z.S.: Stability and bifurcation in a two-species reaction–diffusion–advection competition model with time delay. Nonlinear Anal. Real World Appl. 61, 103327 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  20. Merchant, S.M., Nagata, W.: Instabilities and spatiotemporal patterns behind predator invasions with nonlocal prey competition. Theor. Popul. Biol. 80, 289–297 (2011)

    Article  MATH  Google Scholar 

  21. Mischaikow, K., Smith, H., Thieme, H.R.: Asymptotically autonomous semiflows: chain recurrence and Lyapunov functions. Trans. Am. Math. Soc. 347(5), 1669–1685 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  22. Pao, C.V.: Nonlinear Parabolic and Elliptic Equations. Plenum Press, New York (1992)

    MATH  Google Scholar 

  23. Peng, R., Shi, J., Wang, M.: On stationary patterns of a reaction–diffusion model with autocatalysis and saturation law. Nonlinearity 21(7), 1471–1488 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  24. Peng, R., Shi, J.: Non-existence of non-constant positive steady states of two Holling type-II predator-prey systems: Strong interaction case. J. Differ. Equ. 247, 866–886 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  25. Pijush, P., Nikhil, P., Sudip, S., Joydev, C.: A three species food chain model with fear induced trophic cascade. Int. J. Appl. Comput. Math. 5(100), 1–26 (2019)

    MathSciNet  MATH  Google Scholar 

  26. Suraci, J.P., Clinchy, M., Dill, L.M., Roberts, D., Zanette, L.Y.: Fear of large carnivores causes a trophic cascade. Nat. Commun. 7, 10698 (2016)

    Article  Google Scholar 

  27. Tang, D., Zhou, P.: On a Lotka–Volterra competition-diffusion–advection system: homogeneity vs heterogeneity. J. Differ. Equ. 268(4), 1570–1599 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  28. Tang, D., Chen, Y.M.: Global Dynamics of a Lotka–Volterra competition-diffusion system in advective heterogeneous environments. SIAM J. Appl. Dyn. Syst. 20(3), 1232–1252 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  29. Ge, Q., Tang, D.: Global dynamics of two-species Lotka–Volterra competition–diffusion–advection system with general carrying capacities and intrinsic growth rates. J. Dyn. Differ. Equ. (accepted)

  30. Wang, X., Zanette, L., Zou, X.: Modeling the fear effect in predator–prey interactions. J. Math. Biol. 73, 1179–1204 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  31. Wu, J.H.: Theory and Applications of Partial Functional Differential Equations. Springer, New York (1996)

    Book  MATH  Google Scholar 

  32. Ye, Q., Li, Z., Wang, M., Wu, Y.: Introduction to Reaction–Diffusion Equations. Science Press, Beijing (2011)

    Google Scholar 

  33. Yi, F., Wei, J., Shi, J.: Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system. J. Differ. Equ. 246, 1944–1977 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  34. Zanette, L.Y., White, A.F., Allen, M.C., Michael, C.: Perceived predation risk reduces the number of offspring songbirds produce per year. Science 334, 1398–1401 (2011)

    Article  Google Scholar 

  35. Zhou, J., Mu, C.: Coexistence states of a Holling type-II predator–prey system. J. Math. Anal. Appl. 369, 555–563 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

We sincerely thank the very detailed and helpful referee reports by the anonymous reviewers and helpful suggestions by the editors.

Author information

Authors and Affiliations

  1. College of Computer Engineering Technical (Artificial Intelligence College), Guangdong Polytechnic of Science and Technology, Zhuhai, Guangdong, 519090, People’s Republic of China

    Li Ma

  2. College of Mathematics and Computers, Gannan Normal University, Ganzhou, Jiangxi, 341000, People’s Republic of China

    Huatao Wang

  3. College of Sciences, Chongqing University of Posts and Telecommunications, Chongqing, 400065, People’s Republic of China

    Dong Li

Authors
  1. Li Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Huatao Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dong Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dong Li.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

L. Ma is supported by the NSFC (Grant Nos. 12161003, 12071446, 11801089) and Jiangxi Provincial Natural Science Foundation (Grant Nos. 20202BAB211003, 20224BAB211004). D. Li is supported by Scientific and Technological Research Program of Chongqing Municipal Education Commission (Grant NO. KJQN201900610), Natural Science Foundation of Chongqing, China (Grant No. CSTB2022NSCQ-MSX1204) and NSFC (Grant No. 12001076).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ma, L., Wang, H. & Li, D. Steady states of a diffusive Lotka–Volterra system with fear effects. Z. Angew. Math. Phys. 74, 106 (2023). https://doi.org/10.1007/s00033-023-01998-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00033-023-01998-8

Keywords

Mathematics Subject Classification

Search

Navigation