The stability and Hopf bifurcation of the diffusive Nicholson’s blowflies model in spatially heterogeneous environment

Abstract

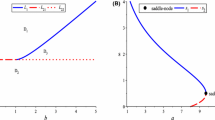
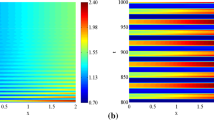
In this paper, we consider the diffusive Nicholson’s blowflies model in spatially heterogeneous environment when the diffusion rate is large. We show that the ratio of the average of the maximum per capita egg production rate to that of the death rate affects the dynamics of the model. The unique positive steady state is locally asymptotically stable if the ratio is less than a critical value. However, when the ratio is greater than the critical value, large time delay can make the unique positive steady state unstable through a Hopf bifurcation. In particular, the first Hopf bifurcation value tends to that of the “average” DDE model when the diffusion rate tends to infinity. Moreover, we show that the direction of the Hopf bifurcation is forward, and the bifurcating periodic solution from the first Hopf bifurcation value is orbitally asymptotically stable, which improves the earlier result by Wei and Li (Nonlinear Anal 60(7):1351–1367, 2005).

A Appendix

1.1 The proof of Proposition 3.3

Proof

It follows from Lemmas 2.4, 3.2 and Theorem 2.6 that

$$\begin{aligned} \begin{aligned} \cos 2\theta _0&=\frac{1-c_0^2+2c_0}{(1-c_0)^2},\quad \sin 2\theta _0=-\frac{2{\sqrt{c_0^2 - 2{c_0}} }}{(1 - {c_0})^2},\quad h_0= {\bar{\delta }} \sqrt{c_0^2 - 2{c_0}},\\&\lim _{r \rightarrow 0}r \tau _n=\frac{\theta _0+2n\pi }{h_0},\quad \lim _{r \rightarrow 0} \nu _r \tau _n=\theta _0+2n\pi ,\\ \lim _{r\rightarrow 0 } {E_r}&= \frac{-e^{-2\mathrm{i}\theta _0 }{\overline{p}} f''(c_0)c_0^2}{e^{-2\mathrm{i}\theta _0 }{\overline{p}} f'(c_0)-{\overline{\delta }}-2\mathrm{i} h_0 },\quad \lim _{r\rightarrow 0 } {F_r} = \frac{-{\overline{p}} f''(c_0)c_0^2}{{\overline{p}} f'(c_0)-{\overline{\delta }}}. \end{aligned} \end{aligned}$$

(A1)

Since $\lim _{r \rightarrow 0 } \psi (x)=\lim _{r \rightarrow 0 } {\overline{\psi }}(x)=c_0$, we see from Eq. (3.8) that

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0 } g_{20}&=\lim _{r \rightarrow 0 }\frac{ r \tau _{n}}{S_{n}(r)} e^{-2 \mathrm{i} \nu _r \tau _n} {\overline{p}} |\Omega | f''(c_0) c_0^3=\lim _{r \rightarrow 0 } g_{11} e^{-2 \mathrm{i} \nu _r \tau _n},\\ \lim _{r \rightarrow 0 } g_{11}&=\lim _{r \rightarrow 0 }\frac{ r \tau _{n}}{S_{n}(r)}{\overline{p}} |\Omega | f''(c_0) c_0^3, \\ \lim _{r \rightarrow 0 } g_{02}&=\lim _{r \rightarrow 0 } \frac{ r \tau _{n}}{S_{n}(r)} e^{2 \mathrm{i} \nu _r \tau _n}{\overline{p}} |\Omega | f''(c_0) c_0^3,\\ \end{aligned} \end{aligned}$$

(A2)

and

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0 } g_{21}&=\lim _{r \rightarrow 0 } \frac{2 r \tau _{n}}{S_{n}(r)} e^{- \mathrm{i} \nu _r \tau _n} {\overline{p}} |\Omega | f''(c_0) c_0^3 (-\frac{\mathrm{i} g_{11}}{\nu _{r} \tau _{n}} e^{- \mathrm{i} \nu _r \tau _n}+\frac{\mathrm{i} \overline{g}_{11}}{\nu _{r} \tau _{n}} e^{ \mathrm{i} \nu _r \tau _n}+\frac{{F_r}}{c_0})\\&\quad +\lim _{r \rightarrow 0 }\frac{r \tau _{n}}{S_{n}(r)} e^{\mathrm{i}\nu _r \tau _n} {\overline{p}} |\Omega | f''(c_0) c_0^3 (\frac{\mathrm{i} g_{20}}{\nu _{r} \tau _{n}} e^{- \mathrm{i} \nu _r \tau _n}+\frac{\mathrm{i} \overline{g}_{02}}{3 \nu _{r} \tau _{n}} e^{ \mathrm{i} \nu _r \tau _n}+\frac{{E_r}e^{-2 \mathrm{i} \nu _r \tau _n}}{c_0})\\&\quad +\lim _{r \rightarrow 0 }\frac{r \tau _{n}}{S_{n}(r)} e^{-\mathrm{i}\nu _r \tau _n} {\overline{p}} |\Omega | f''(c_0) c_0^3 \\&=\lim _{r \rightarrow 0 }\left\{ \frac{2}{\nu _{r} \tau _{n}}\left[ -\mathrm{i}g_{11}g_{20}+\mathrm{i}|g_{11}|^2\right] +g_{11}e^{-\mathrm{i}\nu _r \tau _n}\frac{2{F_r}}{c_0}\right\} \\&\quad +\lim _{r \rightarrow 0 }\left[ \frac{\mathrm{i}g_{11}g_{20}}{\nu _{r} \tau _{n}}+\frac{\mathrm{i}|g_{02}|^2}{3\nu _{r} \tau _{n}}+g_{11}e^{-\mathrm{i} \nu _r \tau _n}\frac{{E_r}}{c_0}\right] \\&\quad +\lim _{r \rightarrow 0 }\left[ -g_{11}e^{-\mathrm{i} \nu _r \tau _n}c_0+g_{11}e^{-\mathrm{i} \nu _r \tau _n}\frac{c_0}{c_0-2}\right] . \end{aligned} \end{aligned}$$

Therefore,

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0 } {\mathcal {R}}e g_{21}&=\lim _{r \rightarrow 0 } {\mathcal {R}}e \left[ \frac{-\mathrm{i}g_{11}g_{20}}{\nu _{r} \tau _{n}} +g_{11}e^{-\mathrm{i}\nu _r \tau _n}\left( \frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0\right) \right] . \end{aligned} \end{aligned}$$

This, combined with Eq. (3.24), implies that

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0}{\mathcal {R}}e C_{1}(0)&=\lim _{r \rightarrow 0}{\mathcal {R}}e \left[ \frac{\mathrm{i}}{2 \nu _r \tau _{n}}\left( g_{11} g_{20}-2\left| g_{11}\right| ^{2}-\frac{\left| g_{02}\right| ^{2}}{3}\right) +\frac{g_{21}}{2}\right] \\&=\lim _{r \rightarrow 0}{\mathcal {R}}e \left( \frac{\mathrm{i} g_{11} g_{20}}{2 \nu _r \tau _{n}}+\frac{g_{21}}{2} \right) \\&=\lim _{r \rightarrow 0}{\mathcal {R}}e \left[ \frac{\mathrm{i} g_{11} g_{20}}{2 \nu _r \tau _{n}}+ \frac{-\mathrm{i}g_{11}g_{20}}{2\nu _{r} \tau _{n}} +\frac{1}{2}g_{11}e^{-\mathrm{i}\nu _r \tau _n}\left( \frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0\right) \right] \\&=\frac{1}{2}\lim _{r \rightarrow 0} {\mathcal {R}}e\left[ g_{11}e^{-\mathrm{i}\nu _r \tau _n}\left( \frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0\right) \right] \\&=\frac{1}{2}\lim _{r \rightarrow 0}\left[ {\mathcal {R}}e (g_{11}e^{-\mathrm{i} \nu _r \tau _n})({\mathcal {R}}e\frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0)\right. \\&\quad \left. - {\mathcal {I}} m \left( g_{11}e^{-\mathrm{i} \nu _r \tau _n}\right) {\mathcal {I}}m\frac{{E_r}}{c_0} \right] . \end{aligned} \end{aligned}$$

(A3)

In order to analyze the sign of $\lim _{r \rightarrow 0}{\mathcal {R}}e C_{1}(0)$, we only need to calculate the signs of $\lim _{r \rightarrow 0}{\mathcal {R}}e (g_{11}e^{-\mathrm{i} \nu _r \tau _n})$, $\lim _{r \rightarrow 0}({\mathcal {R}}e\frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0)$, $\lim _{r \rightarrow 0} {\mathcal {I}} m \left( g_{11}e^{-\mathrm{i} \nu _r \tau _n}\right)$ and $\lim _{r \rightarrow 0}{\mathcal {I}}m\frac{{E_r}}{c_0}$, respectively. From (2.18), we have

$$\begin{aligned} \lim _{r \rightarrow 0}S_n(r)=\lim _{r \rightarrow 0} (1+r \tau _n f'(c_0)e^{-\mathrm{i} \theta _0}{\overline{p}} )c_0^2 |\Omega |, \end{aligned}$$

then

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0} \frac{1}{S_n(r)}&=\lim _{r \rightarrow 0}\frac{1+r \tau _n f'(c_0)e^{\mathrm{i} \theta _0}{\overline{p}} }{(1+r \tau _n f'(c_0)e^{-\mathrm{i} \theta _0}{\overline{p}} )(1+r \tau _n f'(c_0)e^{\mathrm{i} \theta _0}{\overline{p}} )c_0^2 |\Omega |}\\&=\lim _{r \rightarrow 0} \frac{1+r \tau _n f'(c_0){\overline{p}} \cos \theta _0+\mathrm{i} r \tau _n f'(c_0){\overline{p}}\sin \theta _0}{\left[ 1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2\right] c_0^2 |\Omega |}. \end{aligned} \end{aligned}$$

(A4)

It follows from (A2) and (A4) that

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0} g_{11}&=\lim _{r \rightarrow 0 }\frac{ r \tau _{n}}{S_{n}(r)}{\overline{p}} |\Omega | f''(c_0) c_0^3 \\&=\lim _{r \rightarrow 0} \frac{ r \tau _{n}{\overline{p}} f''(c_0) c_0\left[ 1+r \tau _n f'(c_0){\overline{p}} \cos \theta _0+\mathrm{i} r \tau _n f'(c_0){\overline{p}}\sin \theta _0\right] }{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}. \end{aligned} \end{aligned}$$

Then, together with Eq. (A1), yields

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0} {\mathcal {R}}e \left( g_{11}e^{-\mathrm{i} \theta _0}\right)&=\lim _{r \rightarrow 0} \left\{ {\mathcal {R}}e g_{11} \cos \theta _0+ {\mathcal {I}} m g_{11} \sin \theta _0\right\} \\&=\lim _{r \rightarrow 0} \frac{ r \tau _{n}{\overline{p}} f''(c_0) c_0\left( 1+r \tau _n f'(c_0){\overline{p}} \cos \theta _0\right) \cos \theta _0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&\quad +\lim _{r \rightarrow 0} \frac{ r \tau _{n}{\overline{p}} f''(c_0) c_0\left( r \tau _n f'(c_0){\overline{p}}\sin \theta _0\right) \sin \theta _0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&=\lim _{r \rightarrow 0} \frac{r \tau _{n}{\overline{p}} f''(c_0) c_0 \cos \theta _0+ \left( r \tau _{n}{\overline{p}}\right) ^2 f'(c_0) f''(c_0) c_0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&=\frac{(\theta _0+2n\pi ) \sqrt{c_0^2 - 2{c_0}}\frac{1}{1-c_0}+(\theta _0+2n\pi )^2(1-c_0)}{1+2\frac{(\theta _0+2n\pi )}{\sqrt{c_0^2 - 2{c_0}}}+\left[ \frac{(\theta _0+2n\pi )(1-c_0)}{\sqrt{c_0^2 - 2{c_0}}}\right] ^2}, \end{aligned} \end{aligned}$$

(A5)

and

$$\begin{aligned} \begin{aligned} \lim _{r \rightarrow 0} {\mathcal {I}}m \left( g_{11}e^{-\mathrm{i} \theta _0}\right)&=\lim _{r \rightarrow 0}\left\{ -{\mathcal {R}}e g_{11} \sin \theta _0+ {\mathcal {I}} m g_{11} \cos \theta _0\right\} \\&=-\lim _{r \rightarrow 0} \frac{ r \tau _{n}{\overline{p}} f''(c_0) c_0\left( 1+r \tau _n f'(c_0){\overline{p}} \cos \theta _0\right) \sin \theta _0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&\quad +\lim _{r \rightarrow 0} \frac{ r \tau _{n}{\overline{p}} f''(c_0) c_0\left( r \tau _n f'(c_0){\overline{p}}\sin \theta _0\right) \cos \theta _0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&=\lim _{r \rightarrow 0} \frac{- r \tau _{n}{\overline{p}} f''(c_0) c_0\sin \theta _0}{[1+2r \tau _n f'(c_0){\overline{p}} \cos \theta _0+(r \tau _n f'(c_0){\overline{p}})^2]}\\&=\frac{(\theta _0+2n\pi )(c_0 - 2)c_0\frac{1}{1-c_0}}{1+2\frac{(\theta _0+2n\pi )}{\sqrt{c_0^2 - 2{c_0}}}+\left[ \frac{(\theta _0+2n\pi )(1-c_0)}{\sqrt{c_0^2 - 2{c_0}}}\right] ^2}. \end{aligned} \end{aligned}$$

(A6)

Note that

$$\begin{aligned} \begin{aligned} \lim _{r\rightarrow 0 } \frac{{E_r}}{c_0}&= \frac{-e^{-2\mathrm{i}\theta _0 }(c_0-2)c_0}{e^{-2\mathrm{i}\theta _0 }(1-c_0)-1-2\mathrm{i} \sqrt{c_0^2 - 2{c_0}}}\\&=(-\cos 2\theta _0+\mathrm{i}\sin 2\theta _0) (c_0-2)c_0\\&\quad \times \frac{[(1-c_0)\cos 2\theta _0-1]+ \mathrm{i}[(1-c_0)\sin 2\theta _0+2\sqrt{c_0^2 - 2{c_0}}]}{((1-c_0)\cos 2\theta _0-1)^2+ ((1-c_0)\sin 2\theta _0+2\sqrt{c_0^2 - 2{c_0}})^2}. \end{aligned} \end{aligned}$$

Therefore, we obtain

(A7)

and

$$\begin{aligned} \begin{aligned} \lim _{r\rightarrow 0 } {\mathcal {I}}m \frac{{E_r}}{c_0}&=\frac{\left[ -\sin 2\theta _0-2\sqrt{c_0^2 - 2{c_0}}\cos 2\theta _0\right] (c_0-2)c_0}{((1-c_0)\cos 2\theta _0-1)^2+ ((1-c_0)\sin 2\theta _0+2\sqrt{c_0^2 - 2{c_0}})^2}\\&=\frac{\frac{2\sqrt{c_0^2 - 2{c_0}}}{(1-c_0)^2}(c_0^2-2c_0)^2}{((1-c_0)\cos 2\theta _0-1)^2+ ((1-c_0)\sin 2\theta _0+2\sqrt{c_0^2 - 2{c_0}})^2}\\&=\frac{2(c_0^2-2c_0)^{\frac{5}{2}}}{5c_0^4-14c_0^3+9c_0^2}. \end{aligned} \end{aligned}$$

(A8)

From (A1), one also have

$$\begin{aligned} \begin{aligned} \lim _{r\rightarrow 0 }\frac{2{F_r}}{c_0}&=\frac{-2{\overline{p}} f''(c_0)c_0}{{\overline{p}} f'(c_0)-{\overline{\delta }}}=\frac{-2(c_0-2)c_0}{-c_0}=2(c_0-2)>0. \end{aligned} \end{aligned}$$

(A9)

Then we see from (A7) and (A9) that

$$\begin{aligned} \begin{aligned}&\lim _{r \rightarrow 0}({\mathcal {R}}e\frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+ \frac{c_0}{c_0-2}-c_0)\\&\quad =\frac{\left[ (c_0-1)+\frac{1+3c_0^2-6c_0}{(1-c_0)^2}\right] (c_0-2)c_0}{((1-c_0)\cos 2\theta _0-1)^2+ ((1-c_0)\sin 2\theta _0+2\sqrt{c_0^2 - 2{c_0}})^2}+(c_0-4)+\frac{c_0}{c_0-2}\\&\quad =\frac{(c_0^3-3c_0)(c_0-2)^2c_0+(c_0-4)(5c_0^4-14c_0^3+9c_0^2)(c_0-2)+c_0(5c_0^4-14c_0^3+9c_0^2)}{(5c_0^4-14c_0^3+9c_0^2)(c_0-2)}\\&\quad =\frac{(c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)}{(5c_0^4-14c_0^3+9c_0^2)(c_0-2)}>0. \end{aligned} \end{aligned}$$

(A10)

It follows from (A5), (A6), (A8) and (A10) that

$$\begin{aligned} \begin{aligned}&\lim _{r \rightarrow 0}{\mathcal {R}}e C_{1}(0)=\lim _{r \rightarrow 0} {\mathcal {R}}e\left[ g_{11}e^{-\mathrm{i}\nu _r \tau _n}\left( \frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0\right) \right] \\&=\lim _{r \rightarrow 0}\left[ {\mathcal {R}}e (g_{11}e^{-\mathrm{i} \nu _r \tau _n})({\mathcal {R}}e\frac{{E_r}}{c_0}+\frac{2{F_r}}{c_0}+\frac{c_0}{c_0-2}-c_0)- {\mathcal {I}} m \left( g_{11}e^{-\mathrm{i} \nu _r \tau _n}\right) {\mathcal {I}}m\frac{{E_r}}{c_0} \right] \\&=\frac{(\theta _0+2n\pi ) \sqrt{c_0^2 - 2{c_0}}\frac{1}{1-c_0}+(\theta _0+2n\pi )^2(1-c_0)}{1+2\frac{(\theta _0+2n\pi )}{\sqrt{c_0^2 - 2{c_0}}}+\left[ \frac{(\theta _0+2n\pi )(1-c_0)}{\sqrt{c_0^2 - 2{c_0}}}\right] ^2}\\&\quad \times \frac{(c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)}{(5c_0^4-14c_0^3+9c_0^2)(c_0-2)}\\&\quad -\frac{(\theta _0+2n\pi )(c_0 - 2)c_0\frac{1}{1-c_0}}{1+2\frac{(\theta _0+2n\pi )}{\sqrt{c_0^2 - 2{c_0}}}+\left[ \frac{(\theta _0+2n\pi )(1-c_0)}{\sqrt{c_0^2 - 2{c_0}}}\right] ^2} \times \frac{2(c_0^2-2c_0)^{\frac{5}{2}}(c_0-2)}{(5c_0^4-14c_0^3+9c_0^2)(c_0-2)}. \end{aligned} \end{aligned}$$

(A11)

For simplicity, we only calculate the numerator of (A11):

$$\begin{aligned} \begin{aligned}&\left[ (\theta _0+2n\pi ) \sqrt{c_0^2 - 2{c_0}}\frac{1}{1-c_0}+(\theta _0+2n\pi )^2(1-c_0)\right] \\&\quad \times \left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] \\&\quad -(\theta _0+2n\pi )(c_0 - 2)c_0\frac{1}{1-c_0}\times 2(c_0^2-2c_0)^{\frac{5}{2}}(c_0-2)\\&=\frac{2(c_0^2-2c_0)^{\frac{7}{2}}(c_0-2)(\theta _0+2n\pi )}{c_0-1}\\&\quad -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] \\&\quad \times \left[ (c_0-1)(\theta _0+2n\pi )^2+\frac{\sqrt{c_0^2 - 2{c_0}}(\theta _0+2n\pi )}{c_0-1}\right] \\&<\frac{2(c_0^2-2c_0)^{\frac{7}{2}}(c_0-2)(\theta _0+2n\pi )}{c_0-1}\\&\quad -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] (c_0-1)(\theta _0+2n\pi )\\&\quad -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] \frac{\sqrt{c_0^2 - 2{c_0}}(\theta _0+2n\pi )}{c_0-1}\\&=(\theta _0+2n\pi )\left\{ \frac{2(c_0^2-2c_0)^{\frac{7}{2}}(c_0-2)}{c_0-1}\right. \\&\quad \left. -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] (c_0-1)\right\} \\&\quad -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] \frac{\sqrt{c_0^2 - 2{c_0}}(\theta _0+2n\pi )}{c_0-1}. \end{aligned} \end{aligned}$$

(A12)

Let

$$\begin{aligned} \begin{aligned} A&=\frac{2(c_0^2-2c_0)^{\frac{7}{2}}(c_0-2)}{c_0-1}\\&\quad -\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] (c_0-1),\\ B&=-\left[ (c_0^2-3)(c_0-2)^2c_0^2+(5c_0^4-14c_0^3+9c_0^2)(c_0^2-5c_0+8)\right] . \end{aligned} \end{aligned}$$

Then, when $c_0>2$, we have

$$\begin{aligned} \begin{aligned} A&\le \left\{ 2c_0^2(c_0-2)(c_0-1)^3(c_0-2)^2\right. \\&\quad \left. -c_0^2(c_0-1)^2[(c_0^2-3)(c_0-2)^2+(c_0-1)(2c_0-4)(c_0^2-5c_0+8)]\right\} \frac{1}{c_0-1}\\&=\frac{2c_0^2(c_0-2)(c_0-1)^2(c_0-1)(c_0-2)^2-c_0^2(c_0-1)^2(c_0-2)[3c_0^3-14c_0^2+23c_0-10]}{c_0-1}\\&=\frac{c_0^2(c_0-2)(c_0-1)^2[-c_0^3+4c_0^2-7c_0+2]}{c_0-1}\\&=\frac{c_0^2(c_0-2)(c_0-1)^2[-c_0(c_0-2)^2-3c_0+2]}{c_0-1}\\&<0, \end{aligned} \end{aligned}$$

(A13)

and

$$\begin{aligned} \begin{aligned} B=-\left\{ (c_0^2-3)(c_0-2)^2c_0^2+(c_0-1)(5c_0-9)c_0^2\left[ (c_0-\frac{5}{2})^2+\frac{7}{4}\right] \right\} <0. \end{aligned} \end{aligned}$$

(A14)

Summarizing the (A11), (A12), (A13) and (A14), we have $\lim _{r \rightarrow 0}{\mathcal {R}}e C_{1}(0)<0$. $\square$