Abstract
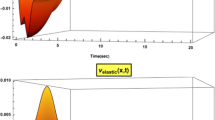
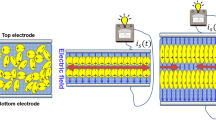
Fully dynamic system of equations for a single piezoelectric beam strongly couples the mechanical (longitudinal) vibrations with the total charge distribution across the beam. Unlike the electrostatic (or quasi-static) assumption of Maxwell’s equations, the hyperbolic-type charge equations have been recently shown to affect the stabilizability of the high-frequency vibrational modes if one considers only a single boundary controller; voltage at the electrodes of the beam. In this paper, we consider viscously damped beam equations and a single distributed state feedback controller with a delay. The effect of the delay in the feedback is investigated for the overall exponential stabilizability dynamics of the piezoelectric beam equations. First, the equations of motion in the state-space formulation are shown to be well-posed by the semigroup theory. Next, an energy approach by the Lyapunov theory is utilized to prove that the exponential stability is retained only if the coefficient of the delayed feedback is strictly less than the coefficient of the state feedback. Finally, the results are compared to the ones of the electrostatic case.

Similar content being viewed by others
References
Dagdeviren, C., Yang, B.D., Su, Y., Tran, P.L., Joe, P., Anderson, E., Xia, J., Doraiswamy, V., Dehdashti, B., Feng, X., Lu, B., Poston, R., Khalpey, Z., Ghaffari, R., Huang, Y., Slepian, M.J., Rogers, J.A.: Conformal piezoelectric energy harvesting and storage from motions of the heart, lung, and diaphragm. Proc. Natl. Acad. Sci. U. S. A. 111, 1927–1932 (2014)
Dagdeviren, C., Joe, P., Tuzman, O.L., Park, K., Lee, K.J., Shi, Y., Huang, Y., Rogers, J.A.: Recent progress in flexible and stretchable piezoelectric devices for mechanical energy harvesting, sensing and actuation. Extrem. Mech. Letter. 9(1), 269–281 (2016)
Datko, R.: Not all feedback stabilized hyperbolic systems are robust with respect to small time delays in their feedbacks. SIAM J. Control Optim. 26(3), 697–713 (1988)
Datko, R.: Two examples of ill-posedness with respect to small delays in stabilized elastic systems. IEEE Trans. Autom. Control 38, 163–166 (1993)
Devasia, S., Eleftheriou, E., Moheimani, S.O.R.: A survey of control issues in nanopositioning. IEEE Trans. Control Syst. Technol. 15(5), 802–823 (2007)
Dong, W., Xiao, L., Hu, W., Zhu, C., Huang, Y., Yin, Z.: Wearable human-machine interface based on PVDF piezoelectric sensor. Trans. Inst. Meas. Control. 39(4), 398–403 (2017)
Ebrahimi, F., Barati, M.R.: Vibration analysis of smart piezoelectrically actuated nano-beams subjected to magneto-electrical field in thermal environment. J. Vib. Control 24(3), 549–564 (2016)
Feng, B., Yang, X.-G.: Long-time dynamics for a nonlinear Timoshenko system with delay. Appl. Anal. 96, 606–625 (2017)
Gu, G.Y., Zhu, L.M., Su, C.Y., Ding, H., Fatikow, S.: Modeling and control of piezo-actuated nanopositioning stages: a survey. IEEE Trans. Autom. Sci. Eng. 13(1), 313–332 (2016)
Kirane, M., Said-Houari, B., Anwar, M.N.: Stability result for the Timoshenko system with a time-varying delay term in the internal feedbacks. Comm. Pure Appl. Anal. 10(2), 667–686 (2011)
Kayacik, O., Bruch, J.C., Sloss, J.M., Adali, S., Sadek, I.S.: Piezo control of free vibrations of damped beams with time delay in the sensor feedback. J. Mech. Adv. Mater. Struct. 16(5), 345–355 (2010)
Morris, K. A., Özer, A. Ö.: Comparison of stabilization of current-actuated and voltage-actuated piezoelectric beams. In: IEEE Proceedings on decision & control, Los Angeles, California, USA, 571–576 (2014)
Morris, K., Özer, A.Ö.: Modeling and stabilizability of voltage-actuated piezo-electric beams with magnetic effects. SIAM J. Control Optim. 52(4), 2371–2398 (2014)
Nicaise, S., Pignotti, C.: Stability and instability results of the wave equation with a delay term in the boundary or internal feedbacks. SIAM J. Control Optim. 45, 1561–1585 (2006)
Nicaise, S., Pignotti, C.: Stabilization of the wave equation with boundary or internal distributed delay. Differ. Integral Equ. 21, 935–958 (2008)
Özer, A.Ö.: Further stabilization and exact observability results for voltage-actuated piezoelectric beams with magnetic effects. Math. Control Signals Syst. 27(2), 219–244 (2015)
Özer, A.Ö.: Modeling and control results for an active constrained layered (ACL) beam actuated by two voltage sources with/without magnetic effects. IEEE Trans. Autom. Control. 62(12), 6445–6450 (2017)
Özer, A.Ö.: Dynamic and non-dynamic modeling for a piezoelectric smart beam and related preliminary stabilization results. Evol. Equ. Control Theory. 7(4), 639–668 (2018)
Pazy, A.: Semigroups of Linear Operators and Applications to Partial Differential Equations. Springer, New York (1983)
Ramos, A.J.A., Gonçalves, C.S., Corrêa Neto, S.S.: Exponential stability and numerical treatment for piezoelectric beams with magnetic effect. Math. Model. Numer. Anal. 52(1), 255–274 (2018)
Ramos, A.J.A., Freitas, M.M., Almeida Júnior, D.S., Jesus, S.S., Moura, T.R.S.: Equivalence between exponential stabilization and boundary observability for piezoelectric beams with magnetic effect. Z. Angew. Math. Phys. 70, 60 (2019)
Roos, J., Bruch, J. C., Jr., Sloss, J. M., Adali, S., Sadek, I. S.: Velocity feedback control with time delay using piezoelectrics. In: Proceedings of the SPIE conference, smart structures and materials 2003: modeling, signal processing, and control, Smith, R. C., Editor, San Diego, CA, 5049, 233–240 (2003)
Said-Houari, B., Laskri, Y.: A stability result of a Timoshenko system with a delay term in the internal feedback. Appl. Math. Comput. 217(6), 2857–2869 (2010)
Shi, Q.: MEMS Based broadband piezoelectric ultrasonic energy harvester (PUEH) for enabling self-powered implantable biomedical devices. Sci. Rep. 6, 24946 (2016)
Sloss, J. M., Adali, S., Sadek, L. S., Bruch, Jr. J. C.: Piezoelectric displacement feedback control with time delay. In: SPIE Conference on mathematics and control in smart structures. SPIE. 3667, 649–656 (1999)
Smith, R. C.: Smart material systems. Society for Industrial and Applied Mathematics. (2005)
Ultrasound imaging of the brain and liver, Science Daily Magazine, Source: Acoustical Society of America, 26 June 2017. Accessed: 25 February 2020
Xu, G.Q., Yung, S.P., Li, L.K.: Stabilization of wave systems with input delay in the boundary control. ESAIM: Control Optim. Calc. Var. 12(4), 770–785 (2006)
Funding
A. J. A. Ramos thanks the CNPq for financial support through the projects “Asymptotic stabilization and numerical treatment for carbon nanotubes” (CNPq Grant 310729/2019-0).
A. Ö. Özer gratefully acknowledges the financial support of the KY NSF grant (#1514712-1) and the RCAP (#20-8038) grant of Western Kentucky University for this research.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Ramos, A.J.A., Özer, A.Ö., Freitas, M.M. et al. Exponential stabilization of fully dynamic and electrostatic piezoelectric beams with delayed distributed damping feedback. Z. Angew. Math. Phys. 72, 26 (2021). https://doi.org/10.1007/s00033-020-01457-8
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00033-020-01457-8
Keywords
- Fully dynamic
- Electrostatic
- Maxwell’s equations
- Piezoelectric beam
- Exponential stability
- Time delayed control
- Distributed feedback control