Skip to main content
SpringerLink
Log in

Finite volume approximation and well-posedness of conservation laws with moving interfaces under abstract coupling conditions

  • Published:
Nonlinear Differential Equations and Applications NoDEA Aims and scope Submit manuscript

Abstract

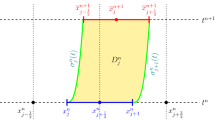
Scalar conservation law \(\displaystyle {\partial _t \rho (t, x) + \partial _x({\textbf{f}}(t, x, \rho )) = 0}\) with a flux \({\textbf{C}}^{1}\) in the state variable \(\rho \), piecewise \({\textbf{C}}^{1}\) in the (t, x)-plane admits infinitely many consistent notions of solution which differ by the choice of interface coupling. Only the case of the so-called vanishing viscosity solutions received full attention, while different choice of coupling is relevant in modeling situations that appear, e.g., in road traffic and in porous medium applications. In this paper, existence of solutions for a wide set of coupling conditions is established under some restrictions on \({\textbf{f}}\), via a finite volume approximation strategy adapted to slanted interfaces and to the presence of interface crossings. The notion of solution, restated under the form of an adapted entropy formulation which is consistently approximated by the numerical scheme, implies uniqueness and stability of solutions. Numerical simulations are presented to illustrate the reliability of the scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Colombo, R.M., Perrollaz, V., Sylla, A.: Conservation Laws and Hamilton-Jacobi Equations with Space Inhomogeneity. Preprint hal.science/hal-03873174, submitted (2022)

  2. Kruzhkov, S.N.: First order quasilinear equations with several independent variables. Math. USSR-Sbornik 81(123), 228–255 (1970)

    MathSciNet  MATH  Google Scholar 

  3. Bressan, A., Guerra, G., Shen, W.: Vanishing viscosity solutions for conservation laws with regulated flux. J. Differ. Equ. 266(1), 312–351 (2019)

    MathSciNet  MATH  Google Scholar 

  4. Karlsen, K.H., Towers, J.D.: Convergence of the Lax-Friedrichs scheme and stability for conservation laws with a discontinous space-time dependent flux. Chin. Ann. Math. Ser. B 25(3), 287–318 (2004)

    MathSciNet  MATH  Google Scholar 

  5. Panov, E.Y.: Existence and strong pre-compactness properties for entropy solutions of a first-order quasilinear equation with discontinuous flux. Arch. Ration. Mech. Anal. 195(2), 643–673 (2010)

    MathSciNet  MATH  Google Scholar 

  6. Gimse, T., Risebro, N.H.: Solution of the Cauchy problem for a conservation law with a discontinuous flux function. SIAM J. Math. Anal. 23(3), 635–648 (1992)

    MathSciNet  MATH  Google Scholar 

  7. Diehl, S.: On scalar conservation laws with point source and discontinuous flux function. SIAM J. Math. Anal. 26(6), 1425–1451 (1995)

    MathSciNet  MATH  Google Scholar 

  8. Diehl, S.: A uniqueness condition for nonlinear convection-diffusion equations with discontinuous coefficients. J. Hyperbolic Differ. Equ. 6(1), 127–159 (2009)

    MathSciNet  MATH  Google Scholar 

  9. Andreianov, B., Karlsen, K.H., Risebro, N.H.: A theory of \(\text{ L}^{1}\)-dissipative solvers for scalar conservation laws with discontinuous flux. Arch. Ration. Mech. Anal. 201(1), 27–86 (2011)

    MathSciNet  MATH  Google Scholar 

  10. Kaasschieter, E.F.: Solving the Buckley- Leverett equation with gravity in a heterogeneous porous medium. Comput. Geosci. 3(1), 23–48 (1999)

    MathSciNet  MATH  Google Scholar 

  11. Adimurthi, Mishra, S., Gowda, G.D.V.: Optimal entropy solutions for conservation laws with discontinuous flux-functions. J. Hyperbolic Differ. Equ. 2(4), 783–837 (2005)

    MathSciNet  MATH  Google Scholar 

  12. Cancès, C.: Asymptotic behavior of two-phase flows in heterogeneous porous media for capillarity depending only on space. I. Convergence to the optimal entropy solution. SIAM J. Math. Anal. 42(2), 946–971 (2010)

    MathSciNet  MATH  Google Scholar 

  13. Andreianov, B., Rosini, M.D.: Microscopic selection of solutions to scalar conservation laws with discontinuous flux in the context of vehicular traffic. In: Semigroups of operators–theory and applications, in J. Banasiak et al.(eds) Semigroups of Operators – Theory and Applications. SOTA 2018. Springer Proceedings in Mathematics & Statistics, vol 325. Springer, Cham, 2020, pp. 113-135 (2020)

  14. Cancès, C.: On the effects of discontinuous capillarities for immiscible two-phase flows in porous media made of several rock-types. Netw. Heterog. Media 5(3), 635–647 (2010)

    MathSciNet  MATH  Google Scholar 

  15. Andreianov, B., Cancès, C.: Vanishing capillarity solutions of Buckley- Leverett equation with gravity in two-rocks’ medium. Comput. Geosci. 17(3), 551–572 (2013)

    MathSciNet  MATH  Google Scholar 

  16. Colombo, R.M., Goatin, P.: A well posed conservation law with a variable unilateral constraint. J. Differ. Equ. 234(2), 654–675 (2007)

    MathSciNet  MATH  Google Scholar 

  17. Andreianov, B., Goatin, P., Seguin, N.: Finite volume schemes for locally constrained conservation laws. Numer. Math. 115(4), 609–645 (2010)

    MathSciNet  MATH  Google Scholar 

  18. Andreianov, B.: New approaches to describing admissibility of solutions of scalar conservation laws with discontinuous flux. ESAIM Proc. Surv. 50, 40–65 (2015)

    MathSciNet  MATH  Google Scholar 

  19. Andreianov, B., Sbihi, K.: Well-posedness of general boundary-value problems for scalar conservation laws. Trans. Amer. Math. Soc. 367(6), 3763–3806 (2015)

    MathSciNet  MATH  Google Scholar 

  20. Crasta, G., De Cicco, V., De Philippis, G., Ghiraldin, F.: Structure of solutions of multidimensional conservation laws with discontinuous flux and applications to uniqueness. Arch. Ration. Mech. Anal. 221(2), 961–985 (2016)

    MathSciNet  MATH  Google Scholar 

  21. Andreianov, B., Mitrović, D.: Entropy conditions for scalar conservation laws with discontinuous flux revisited. Ann. Inst. H. Poincaré Anal. Non Linéaire 32(6), 1307–1335 (2015)

    MathSciNet  MATH  Google Scholar 

  22. Audusse, E., Perthame, B.: Uniqueness for scalar conservation laws with discontinuous flux via adapted entropies. Proc. Roy. Soc. Edinburgh Sect. A 135(2), 253–265 (2005)

    MathSciNet  MATH  Google Scholar 

  23. Panov, E.Y.: On existence and uniqueness of entropy solutions to the Cauchy problem for a conservation law with discontinuous flux. J. Hyperbolic Differ. Equ. 6(3), 525–548 (2009)

    MathSciNet  MATH  Google Scholar 

  24. Towers, J.D.: Convergence of a difference scheme for conservation laws with a discontinuous flux. SIAM J. Numer. Anal. 38(2), 681–698 (2000)

    MathSciNet  MATH  Google Scholar 

  25. Adimurthi, A., Gowda, G.D.V.: Conservation law with discontinuous flux. J. Math. Kyoto Univ. 43(1), 27–70 (2003)

    MathSciNet  MATH  Google Scholar 

  26. Bürger, R., Karlsen, K.H., Towers, J.D.: An Engquist- Osher-type scheme for conservation laws with discontinuous flux adapted to flux connections. SIAM J. Numer. Anal. 47(3), 1684–1712 (2009)

    MathSciNet  MATH  Google Scholar 

  27. Andreianov, B., Lagoutière, F., Seguin, N., Takahashi, T.: Well-posedness for a one-dimensional fluid-particle interaction model. SIAM J. Math. Anal. 46(2), 1030–1052 (2014)

    MathSciNet  MATH  Google Scholar 

  28. Karlsen, K.H., Risebro, N.H., Towers, J.D.: \(L^1\) stability for entropy solutions of nonlinear degenerate parabolic convection-diffusion equations with discontinuous coefficients. Skr. K. Nor. Vidensk. Selsk. 1(3), 1–49 (2003)

    MATH  Google Scholar 

  29. Coclite, G.M., Risebro, N.H.: Conservation laws with time dependent discontinuous coefficients. SIAM J. Math. Anal. 36(4), 1293–1309 (2005)

    MathSciNet  MATH  Google Scholar 

  30. Andreianov, B., Karlsen, K.H., Risebro, N.H.: On vanishing viscosity approximation of conservation laws with discontinuous flux. Netw. Heterog. Media 5(3), 617–633 (2010)

    MathSciNet  MATH  Google Scholar 

  31. Karlsen, K.H., Towers, J.D.: Convergence of a Godunov scheme for conservation laws with a discontinuous flux lacking the crossing condition. J. Hyperbolic Differ. Equ. 14(4), 671–701 (2017)

    MathSciNet  MATH  Google Scholar 

  32. Towers, J.D.: Convergence via OSLC of the godunov scheme for a scalar conservation law with time and space flux discontinuities. Numer. Math. 139(4), 939–969 (2018)

    MathSciNet  MATH  Google Scholar 

  33. Andreianov, B., Cancès, C.: On interface transmission conditions for conservation laws with discontinuous flux of general shape. J. Hyperbolic Differ. Equ. 12(2), 343–384 (2015)

    MathSciNet  MATH  Google Scholar 

  34. Bachmann, F., Vovelle, J.: Existence and uniqueness of entropy solution of scalar conservation laws with a flux function involving discontinuous coefficients. Comm. Partial Differ. Equ. 31(1–3), 371–395 (2006)

    MathSciNet  MATH  Google Scholar 

  35. Shen, W.: On the uniqueness of vanishing viscosity solutions for Riemann problems for polymer flooding. NoDEA Nonlinear Differ. Equ. Appl. 24(4), 1–25 (2017)

    MathSciNet  Google Scholar 

  36. Bürger, R., Karlsen, K.H., Klingenberg, C., Risebro, N.H.: A front tracking approach to a model of continuous sedimentation in ideal clarifier-thickener units. Nonlin. Anal. Real World Appl. 4(3), 457–481 (2003)

    MathSciNet  MATH  Google Scholar 

  37. Garavello, M., Natalini, R., Piccoli, B., Terracina, A.: Conservation laws with discontinuous flux. Netw. Heterog. Media 2(1), 159–179 (2007)

    MathSciNet  MATH  Google Scholar 

  38. Sylla, A.: A LWR model with constraints at moving interfaces. ESAIM Math. Model. Numer. Anal. 56(3), 1081–1114 (2022)

    MathSciNet  MATH  Google Scholar 

  39. Sylla, A.: Influence of a slow moving vehicle on traffic: Well-posedness and approximation for a mildly nonlocal model. Netw. Heterog. Media 16(2), 221–256 (2021)

    MathSciNet  MATH  Google Scholar 

  40. Andreianov, B., Girard, T.: Existence of solutions to a class of one-dimensional models for pedestrian evacuation. Preprint hal-03937464 (2023)

  41. Vasseur, A.: Strong traces for solutions of multidimensional scalar conservation laws. Arch. Ration. Mech. Anal. 160(3), 181–193 (2001)

    MathSciNet  MATH  Google Scholar 

  42. Panov, E.Y.: Existence of strong traces for quasi-solutions of multidimensional conservation laws. J. Hyperbolic Differ. Equ. 4(4), 729–770 (2007)

    MathSciNet  MATH  Google Scholar 

  43. Cancès, C., Gallouët, T.: On the time continuity of entropy solutions. J. Evol. Equ. 11(1), 43–55 (2011)

    MathSciNet  MATH  Google Scholar 

  44. Colombo, R.M., Mercier, M., Rosini, M.D.: Stability and total variation estimates on general scalar balance laws. Comm. Math. Sci. 7(1), 37–65 (2009)

    MathSciNet  MATH  Google Scholar 

  45. Aleksić, J., Mitrović, D.: Strong traces for averaged solutions of heterogeneous ultra-parabolic transport equations. J. Hyperbolic Differ. Equ. 10(4), 659–676 (2013)

    MathSciNet  MATH  Google Scholar 

  46. Neves, W., Panov, E.Y., Silva, J.: Strong traces for conservation laws with general nonautonomous flux. SIAM J. Math. Anal. 50(6), 6049–6081 (2018)

    MathSciNet  MATH  Google Scholar 

  47. Crasta, G., De Cicco, V., De Philippis, G.: Kinetic formulation and uniqueness for scalar conservation laws with discontinuous flux. Comm. Partial Differ. Equ. 40(4), 694–726 (2015)

    MathSciNet  MATH  Google Scholar 

  48. Amadori, D., Goatin, P., Rosini, M.D.: Existence results for Hughes’ model for pedestrian flows. J. Math. Anal. Appl. 420(1), 387–406 (2014)

    MathSciNet  MATH  Google Scholar 

  49. Cancès, C., Seguin, N.: Error estimate for Godunov approximation of locally constrained conservation laws. SIAM J. Numer. Anal. 50(6), 3036–3060 (2012)

    MathSciNet  MATH  Google Scholar 

  50. Panov, E.Y.: On strong precompactness of bounded sets of measure-valued solutions of a first order quasilinear equation. Sbornik Math. 186(5), 729 (1995)

    MathSciNet  MATH  Google Scholar 

  51. Eymard, R., Gallouët, T., Herbin, R.: Finite Volume Methods. Handbook of Numerical Analysis, vol. 7, pp. 713–1020. Elsevier (2000)

  52. Andreianov, B., Coclite, G.M., Donadello, C.: Well-posedness for vanishing viscosity solutions of scalar conservation laws on a network. Discret. Contin. Dyn. Syst. 37(11), 5913–5942 (2017)

    MathSciNet  MATH  Google Scholar 

  53. Hughes, R.L.: A continuum theory for the flow of pedestrians. Trans. Res. Part B Methodol. 36(6), 507–535 (2002)

    Google Scholar 

  54. El-Khatib, N., Goatin, P., Rosini, M.D.: On entropy weak solutions of Hughes’ model for pedestrian motion. Z. Angew. Math. Phys. 64(2), 223–251 (2013)

    MathSciNet  MATH  Google Scholar 

  55. Andreianov, B., Rosini, M.D., Stivaletta, G.: On existence, stability and many-particle approximation of solutions of 1D Hughes model with linear costs. Preprint hal.science.fr/hal-03289551, submitted (2021)

  56. Lagoutière, F., Seguin, N., Takahashi, T.: A simple 1D model of inviscid fluid-solid interaction. J. Differ. Equ. 245(11), 3503–3544 (2008)

    MathSciNet  MATH  Google Scholar 

  57. Andreianov, B., Lagoutière, F., Seguin, N., Takahashi, T.: Small solids in an inviscid fluid. Netw. Heterog. Media 5(3), 385 (2010)

    MathSciNet  MATH  Google Scholar 

  58. Towers, J.D.: The Lax-Friedrichs scheme for interaction between the inviscid Burgers equation and multiple particles. Netw. Heterog. Media 15(1), 143–169 (2020)

    MathSciNet  MATH  Google Scholar 

  59. Gyamfi, K.A.: Analysis of entropy solutions to conservation laws with discontinuous flux in space and time. PhD thesis, Univ. Degli Studi dell’Aquila (2021)

  60. Aguillon, N., Lagoutière, F., Seguin, N.: Convergence of finite volume schemes for the coupling between the inviscid Burgers equation and a particle. Math. Comp. 86(303), 157–196 (2017)

    MathSciNet  MATH  Google Scholar 

  61. Towers, J.D.: A fixed grid, shifted stencil scheme for inviscid fluid-particle interaction. Appl. Numer. Math. 110, 26–40 (2016)

    MathSciNet  MATH  Google Scholar 

  62. Delle Monache, M.L., Goatin, P.: Scalar conservation laws with moving constraints arising in traffic flow modeling: an existence result. J. Differ. Equ. 257(11), 4015–4029 (2014)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This paper has been supported by the RUDN University Strategic Academic Leadership Program. The work on this paper was supported by l’Agence Nationale de la Recherche (ANR), project ANR-22-CE40-0010 (ANR CoSS).

Funding

No funding was received for conducting this study.

Author information

Authors and Affiliations

  1. Institut Denis Poisson CNRS UMR 7013, Université de Tours, Université d’Orléans, Parc Grandmont, 37200, Tours, France

    Boris Andreianov

  2. Peoples’ Friendship University of Russia (RUDN University), 6 Miklukho-Maklaya St, Moscow, Russian Federation, 117198

    Boris Andreianov

  3. Dipartimento di Matematica e Applicazioni, Università di Milano-Bicocca, Via R. Cozzi 53, 20125, Milan, Italy

    Abraham Sylla

Authors
  1. Boris Andreianov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Abraham Sylla
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors equally contributed to elaboration of statements and proofs, to writing and re-reading of the manuscript. AS implemented the scheme and carried out the numerical tests.

Corresponding authors

Correspondence to Boris Andreianov or Abraham Sylla.

Ethics declarations

Conflict of interest

The authors have no conflict of interest to declare that are relevant to the content of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Andreianov, B., Sylla, A. Finite volume approximation and well-posedness of conservation laws with moving interfaces under abstract coupling conditions. Nonlinear Differ. Equ. Appl. 30, 53 (2023). https://doi.org/10.1007/s00030-023-00857-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00030-023-00857-9

Keywords

Mathematics Subject Classification

Search

Navigation