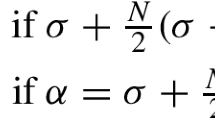Abstract
In this paper, we study the following reaction-diffusion-advection system
in a smoothly bounded domain \(\Omega \subset {\mathbb {R}}^{n}\), which describes a directed movement of immune cells toward chemokines during the immune process, where \(D_{u},D_{v},D_{w},s_{v},s_{w},\lambda _{w}\), \(\mu _{v},\mu _{w},\chi \) are positive parameters, and \(f\in C^{1}([0,\infty ))\) is a kinetic function. When \(n\ge 1\), if there exist positive constants \(\alpha \) and \(\theta _{0}\) such that \(\sup _{s\ge 0}\{f(s)\!+\!\alpha s\}<\infty \) and \(\lim _{s\rightarrow \infty }\inf \left\{ -\!\frac{f(s)}{s^{2}}\right\} =:\mu \in (\theta _{0},\infty ]\), then the solution of the system is global and uniformly bounded. In particular, when \(n=2\) and \(f(0)\ge 0\), the condition of f(u) could be improved as follows: if there exists \(\alpha >0\) such that \(\sup _{s\ge 0}\{f(s)+\alpha s\}<\infty \) and one of the conditions that \(\lim _{s\rightarrow \infty }\inf \left\{ -\frac{f(s)\ln s}{s^{2}}\right\} =:\mu \in (\sqrt{2}\frac{\chi s_{v}C_{w}}{D_{v}},\infty ]\) or \(\frac{2\sqrt{2}\chi s_{v}C_{GN}^{4}m_{1}C_{w}}{D_{v}}\le D_{u}\) holds, then the solution of the system is still global and uniformly bounded, where \(m_{1}\) is a positive constant given by below, \(C_{GN}>0\) is the Gagliardo-Nirenberg inequality’s constant and \(C_{w}\) represents the uniform upper bound of w. Moreover, when \(f\equiv 0\) and \(\frac{2\sqrt{2}\chi s_{v}C_{GN}^{4}m_{1}C_{w}}{D_{v}}\le D_{u}\), the global and uniform boundedness of solutions can also be established.
Similar content being viewed by others
References
Amann, H.: Dynamic theory of quasilinear parabolic equations II. Reaction-diffusion systems. Differ. Integr. Equ. 3(1), 13–75 (1990)
Bellomo, N., Bellouquid, A., Tao, Y., Winkler, M.: Towards a mathematical theory of Keller–Segel models of pattern formation in biological tissues. Math. Mod. Meth. Appl. Sci. 25, 1663–1763 (2015)
Bellomo, N., Painter, K.J., Tao, Y., Winkler, M.: Occurrence vs. absence of taxis-driven instabilities in a May–Nowak model for virus infection. SIAM J. Appl. Math. 79(5), 1990–2010 (2019)
Bellomo, N., Tao, Y.: Stabilization in a chemotaxis model for virus infection. Discr. Contin. Dyn. Syst. Ser. S. 13, 105–117 (2020)
Biler, P., Hebisch, W., Nadzieja, T.: The Debye system: existence and large time behavior of solutions. Nonlin. Anal. 23, 1189–1209 (1994)
Chaplain, M.A.J., Tello, J.I.: On the stability of homogeneous steady states of a chemotaxis system with logistic growth term. Appl. Math. Lett. 57, 1–6 (2016)
Devreotes, P., Janetopoulos, C.: Eukaryotic chemotaxis: distinctions between directional sensing and polarization. J. Biol. Chem. 278, 20445–20448 (2003)
Eftimie, R., Gillard, J., Cantrell, D.A.: Mathematical models for immunology: current state of the art and future research directions. Bull. Math. Biol. 78, 2091–2134 (2016)
Fishman, M.A., Perelson, A.S.: Modeling T cell-antigen presenting cell interactions. J. Theor. Biol. 160, 311–342 (1993)
Fuest, M.: Boundedness enforced by mildly saturated conversion in a chemotaxis–May–Nowak model for virus infection. J. Math. Anal. Appl. 472, 1729–1740 (2019)
Gajewski, H., Zacharias, K.: Global behavior of a reaction-diffusion system modelling chemotaxis. Math. Nachr. 195, 77–144 (1998)
Gereda, J.E., Leung, D.Y.M., Thatayatikom, A., Streib, J.E., Price, M.R.: Relation between house-dust endotoxin exposure, type 1 T-cell development, and allergen sensitisation in infants at high risk of asthma. The Lancet. 355, 1680–1683 (2000)
Groß, F., Fridolin, M., Behn, U.: Mathematical modeling of allergy and specific immunotherapy: Th1-Th2-Treg interactions. J. Theor. Biol. 269, 70–78 (2011)
Horstmann, D.: The nonsymmetric case of the Keller–Segel model in chemotaxis: some recent results. Nonlin. Differ. Equ. Appl. 8, 399–423 (2001)
Horstmann, D., Wang, G.: Blow-up in a chemotaxis model without symmetry assumptions. Eur. J. Appl. Math. 12, 159–177 (2001)
Horstmann, D., Winkler, M.: Boundedness vs. blow-up in a chemotaxis system. J. Differ. Equ. 215(1), 52–107 (2005)
Ishida, S., Seki, K., Yokota, T.: Boundedness in quasilinear Keller–Segel systems of parabolic-parabolic type on non-convex bounded domains. J. Differ. Equ. 256, 2993–3010 (2014)
Keller, E.F., Segel, L.A.: Initiation of slime mold aggregation viewed as an instability. J. Theor. Biol. 26, 399–415 (1970)
Kowalczyk, R.: On the global existence of solutions to an aggregation model. J. Math. Anal. Appl. 343, 379–398 (2008)
Lai, X., Zou, X.: Repulsion effect on superinfecting virions by infected cells. Bull. Math. Biol. 76, 2806–2833 (2014)
Lee, S., Kim, S., Oh, Y., Hwang, H.J.: Mathematical modeling and its analysis for instability of the immune system induced by chemotaxis. J. Math. Biol. 75, 1101–1131 (2017)
Li, X.: Global existence and boundedness of a chemotaxis model with indirect production and general kinetic function. Z. Angew. Math. Phys. 71, 1–22 (2020)
Mizoguchi, N., Souplet, P.: Nondegeneracy of blow-up points for the parabolic Keller-Segel system. Ann. Inst. H. Poincaré Anal. Non Linaire. 31, 851–875 (2014)
Nagai, T., Senba, T., Yoshida, K.: Application of the Trudinger–Moser inequality to a parabolic system of chemotaxis. Funk. Ekva. 40, 411–433 (1997)
Nirenberg, L.: An extended interpolation inequality. Ann. Scuola Norm-Sci. 20, 733–737 (1966)
Osaki, K., Tsujikawa, T., Yagi, A., Mimura, M.: Exponential attracktor for a chemotaxis-growth system of equations. Nonlin. Anal. 51, 119–144 (2002)
Osaki, K., Yagi, A.: Finite dimensional attractors for one-dimensional Keller-Segel equations. Funkcial. Ekvac. 44, 441–469 (2001)
Osaki, K., Yagi, A.: Global existence for a chemotaxis-growth system in \(R^2\). Adv. Math. Sci. Appl. 12, 587–606 (2002)
Pan, X., Wang, L., Hu, X.: Boundedness and stabilization of solutions to a chemotaxis May-Nowak model. Z. Angew. Math. Phys. 72, 1–16 (2021)
Pigozzo, A.B., Macedo, G.C., Santos, R.W.D., Lobosco, M.: On the computational modeling of the innate immune system. Bio. Med. Central. 14, 1–20 (2013)
Stancevic, O., Angstmann, C.N., Murray, J.M., Henry, B.I.: Turing patterns from dynamics of early HIV infection. Bull. Math. Biol. 75, 774–795 (2013)
Su, B., Zhou, W., Dorman, K.S., Jones, D.E.: Mathematical modelling of immune response in tissues. Comput. Math. Methods Med. 10, 9–38 (2009)
Tao, Y., Winkler, M.: Boundedness in a quasilinear parabolic-parabolic Keller–Segel system with subcritical sensitivity. J. Differ. Equ. 252, 692–715 (2012)
Wang, W., Ma, W., Lai, X.: Repulsion effect on superinfecting virions by infected cells for virus infection dynamic model with absorption effect and chemotaxis. Nonlin. Anal. RWA. 33, 253–283 (2017)
Wang, W., Zhang, M., Zheng, S.: Positive effects of repulsion on boundedness in a fully parabolic attraction-repulsion chemotaxis system with logistic source. J. Differ. Equ. 264, 2011–2027 (2018)
Winkler, M.: Aggregation vs. global diffusive behavior in the higher-dimensional Keller–Segel model. J. Differ. Equ. 248(12), 2889–2905 (2010)
Winkler, M.: Boundedness in the higher-dimensional parabolic-parabolic chemotaxis system with logistic source. Commun. Partial Differ. Equ. 35, 1516–1537 (2010)
Winkler, M.: Blow-up in a higher-dimensional chemotaxis system despite logistic growth restriction. J. Math. Anal. Appl. 384, 261–272 (2011)
Winkler, M.: Boundedness in a chemotaxis-May-Nowak model for virus dynamics with mildly saturated chemotactic sensitivity. Acta Appl. Math. 163, 1–17 (2019)
Winkler, M.: Finite-time blow-up in the higher-dimensional parabolic-parabolic Keller–Segel system. J. Math. Pures Appl. 100, 748–767 (2013)
Winkler, M.: Global asymptotic stability of constant equilibria in a fully parabolic chemotaxis system with strong logistic dampening. J. Differ. Equ. 257, 1056–1077 (2014)
Wu, S.: Global boundedness of a diffusive prey-predator model with indirect prey-taxis and predator-taxis. J. Math. Anal. Appl. 507, 125820 (2022)
Xiang, T.: Sub-logistic source can prevent blow-up in the 2D minimal Keller–Segel chemotaxis system. J. Math. Phys. 59, 081502 (2018)
Xiang, T.: Boundedness and global existence in the higher-dimensional parabolic-parabolic chemotaxis system with/without growth source. J. Differ. Equ. 258, 4275–4323 (2015)
Yoon, C., Kim, S., Hwang, H.J.: Global well-posedness and pattern formations of the immune system induced by chemotaxis. Math. Biosci. Eng. 17, 3426–3449 (2020)
Zhelev, D.V., Alteraifi, A.M., Chodniewicz, D.: Controlled pseudopod extension of human neutrophils stimulated with different chemoattractants. Biophys. J. 87, 688–695 (2004)
Zheng, P., Mu, C., Hu, X.: Persistence property in a two-species chemotaxis system with two signals. J. Math. Phys. 58, 111501 (2017)
Zheng, P., Mu, C., Mi, Y.: Global stability in a two-competing-species chemotaxis system with two chemicals. Diff. Integ. Equ. 31, 547–558 (2018)
Zheng, P., Xing, J.: Boundedness and large-time behavior for a two-dimensional quasilinear chemotaxis-growth system with indirect signal consumption. Z. Angew. Math. Phys. 71(3), 71–98 (2020)
Acknowledgements
The authors would like to deeply thank the editor and anonymous reviewers for their insightful and constructive comments. Pan Zheng thanks for the friendly hospitality of The Chinese University of Hong Kong during the postdoctoral research. The work is partially supported by National Natural Science Foundation of China (Grant Nos.: 11601053, 12271064), the Science and Technology Research Project of Chongqing Municipal Education Commission (Grant No. KJZDK202200602), Natural Science Foundation of Chongqing (Grant No. cstc2019jcyj-msxmX0082), China-South Africa Young Scientist Exchange Project in 2020, The Hong Kong Scholars Program (Grant Nos.: XJ2021042, 2021-005),Young Hundred Talents Program of CQUPT in 2022-2024 and Chongqing Postgraduate Research and Innovation Project in 2022 (Grant Nos: CYS22451).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Shan, W., Zheng, P. Global boundedness of the immune chemotaxis system with general kinetic functions. Nonlinear Differ. Equ. Appl. 30, 29 (2023). https://doi.org/10.1007/s00030-023-00840-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00030-023-00840-4