Skip to main content
SpringerLink
Log in

On the Faedo–Galerkin Method for Non-autonomous Nonlinear Differential Systems

  • Published:
Results in Mathematics Aims and scope Submit manuscript

Abstract

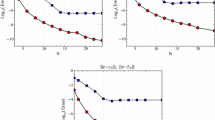
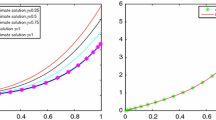
This article contemplates a non-autonomous nonlinear differential system in a separable Hilbert space \(\mathcal {X}\). The projection operators are used to confine our concern to a finite-dimensional subspace of \(\mathcal {X}\). The existence, uniqueness, and convergence of approximate solutions are explored by making use of the hypothesis of fractional powers of a closed linear operator. We also analyze the Faedo–Galerkin approximation of the solution. An example is also built to exhibit the significance of the acquired results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Data Availability

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Amann, H.: Parabolic evolution equations and nonlinear boundary conditions. J. Differ. Equ. 72(2), 201–269 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  2. Arora, S., Mohan, M.T., Dabas, J.: Existence and approximate controllability of non-autonomous functional impulsive evolution inclusions in Banach spaces. J. Differ. Equ. 307, 83–113 (2022)

    Article  MATH  MathSciNet  Google Scholar 

  3. Bahuguna, D., Shukla, R.: Approximations of solutions to second order semilinear integrodifferential equations. Numer. Funct. Anal. Optim. 24(3–4), 365–390 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  4. Balasubramaniam, P., Ali, M.S., Kim, J.H.: Faedo-Galerkin approximate solutions for stochastic semilinear integrodifferential equations. Comput. Math. Appl. 58(1), 48–57 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  5. Browder, F.E.: Non-linear equations of evolution. Ann. Math. (2) 80(3), 485–523 (1964)

    Article  MATH  MathSciNet  Google Scholar 

  6. Browder, F.E.: Existence of periodic solutions for nonlinear equations of evolution. Proc. Nat. Acad. Sci. USA 53, 1100–1103 (1965)

    Article  MATH  MathSciNet  Google Scholar 

  7. Cao, J., Huang, Z., N’Guérékata, G.M.: Existence of asymptotically almost automorphic mild solutions for nonautonomous semilinear evolution equations. Electron. J. Differ. Equ. 2018(37), 1–16 (2018)

    MATH  MathSciNet  Google Scholar 

  8. Chadha, A., Pandey, D.N.: Mild solutions for non-autonomous impulsive semi-linear differential equations with iterated deviating arguments. Electron. J. Differ. Equ. 2015(222), 1–14 (2015)

    MATH  MathSciNet  Google Scholar 

  9. Chen, P., Zhang, X., Li, Y.: Approximate controllability of non-autonomous evolution system with nonlocal conditions. J. Dyn. Control Syst. 26(1), 1–16 (2020)

    Article  MATH  MathSciNet  Google Scholar 

  10. Diagana, T.: Stepanov-like pseudo-almost periodicity and its applications to some nonautonomous differential equations. Nonlinear Anal. 69(12), 4277–4285 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  11. Fitzgibbon, W.E.: Semilinear functional differential equations in Banach space. J. Differ. Equ. 29(1), 1–14 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  12. Friedman, A.: Partial Differential Equations. Holt, Rinehart and Winston, New York (1969)

    MATH  Google Scholar 

  13. Fu, X.: Existence of solutions for non-autonomous functional evolution equations with nonlocal conditions. Electron. J. Differ. Equ. 2012(110), 1–15 (2012)

    MathSciNet  Google Scholar 

  14. Goethel, R.: Faedo–Galerkin approximations in equations of evolution. Math. Methods Appl. Sci. 6(1), 41–54 (1984)

    Article  MathSciNet  Google Scholar 

  15. Haloi, R., Pandey, D.N., Bahuguna, D.: Existence of solutions to a non-autonomous abstract neutral differential equation with deviated argument. J. Nonlinear Evol. Equ. Appl. 2011(5), 75–90 (2011)

    MATH  MathSciNet  Google Scholar 

  16. Henry, D.: Geometric Theory of Semilinear Parabolic Equations. Springer, Berlin (1981)

    Book  MATH  Google Scholar 

  17. Jerome, J.W.: The quantum Faedo–Galerkin equation: evolution operator and time discretization. Numer. Funct. Anal. Optim. 38(5), 590–601 (2017)

    Article  MATH  MathSciNet  Google Scholar 

  18. Kaliraj, K., Manjula, M., Ravichandran, C.: New existence results on nonlocal neutral fractional differential equation in concepts of Caputo derivative with impulsive conditions. Chaos Solitons Fractals 161(112284), 1–11 (2022)

    MATH  MathSciNet  Google Scholar 

  19. Kumar, S., Sharma, P.: Faedo–Galerkin method for impulsive second-order stochastic integro-differential systems. Chaos Solitons Fractals 158(111946), 1–16 (2022)

    MATH  MathSciNet  Google Scholar 

  20. Manjula, M., Kaliraj, K., Botmart, T., Nisar, K.S., Ravichandran, C.: Existence, uniqueness and approximation of nonlocal fractional differential equation of sobolev type with impulses. AIMS Math. 8(2), 4645–4665 (2023)

    Article  MathSciNet  Google Scholar 

  21. Miletta, P.D.: Approximation of solutions to evolution equations. Math. Methods Appl. Sci. 17(10), 753–763 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  22. Muslim, M.: Faedo–Galerkin approximation of second order nonlinear differential equation with deviated argument. Appl. Math. Comput. 329, 315–324 (2018)

    MATH  MathSciNet  Google Scholar 

  23. Muslim, M.: Faedo–Galerkin approximations to fractional integro-differential equation of order \(\alpha \in (1,2]\) with deviated argument. Dyn. Partial Differ. Equ. 13(4), 351–368 (2016)

    Article  MATH  MathSciNet  Google Scholar 

  24. Muslim, M., Agarwal, R.P.: Approximation of solutions to impulsive functional differential equations. J. Appl. Math. Comput. 34(1–2), 101–112 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  25. Pazy, A.: Semigroups of Linear Operators and Applications to Partial Differential Equations. Springer, New York (1983)

    Book  MATH  Google Scholar 

  26. Raheem, A., Kumar, M.: An approximate solution to a class of impulsive fractional differential equations in a reflexive Banach space. Int. J. Appl. Comput. Math. 5(111), 1–16 (2019)

    MATH  MathSciNet  Google Scholar 

  27. Rankin, S.M.: Existence and asymptotic behavior of a functional differential equation in Banach space. J. Math. Anal. Appl. 88(2), 531–542 (1982)

    Article  MATH  MathSciNet  Google Scholar 

  28. Sousa, J.V.C., Fečkan, M., Oliveira, E.C.: Faedo–Galerkin approximation of mild solutions of fractional functional differential equations. Nonauton. Dyn. Syst. 8(1), 1–17 (2021)

    Article  MATH  MathSciNet  Google Scholar 

  29. Tanabe, H.: Functional Analytic Methods for Partial Differential Equations. Marcel Dekker, New York (1997)

    MATH  Google Scholar 

  30. Xiao, T.J., Zhu, X.X., Liang, J.: Pseudo-almost automorphic mild solutions to nonautonomous differential equations and applications. Nonlinear Anal. 70(11), 4079–4085 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  31. Yagi, A.: Abstract Parabolic Evolution Equations and their Applications. Springer, Berlin (2010)

    Book  MATH  Google Scholar 

  32. Zadoyanchuk, N.V., Kas’yanov, P.O.: Faedo–Galerkin method for nonlinear second-order evolution equations with Volterra operators. Nonlinear Oscil. 10(2), 203–228 (2007)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Acknowledgements

The work of the first author is supported by IoE, University of Delhi.

Author information

Author notes

  1. Surendra Kumar and Paras Sharma have contributed equally to this work.

Authors and Affiliations

  1. Department of Mathematics, University of Delhi, New Delhi, Delhi, 110007, India

    Surendra Kumar & Paras Sharma

Authors
  1. Surendra Kumar
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Paras Sharma
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Surendra Kumar.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kumar, S., Sharma, P. On the Faedo–Galerkin Method for Non-autonomous Nonlinear Differential Systems. Results Math 78, 107 (2023). https://doi.org/10.1007/s00025-023-01894-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00025-023-01894-7

Keywords

Mathematics Subject Classification

Search

Navigation