Skip to main content
SpringerLink
Log in

Small Data Global Existence and Decay for Relativistic Chern–Simons Equations

  • Published:
Annales Henri Poincaré Aims and scope Submit manuscript

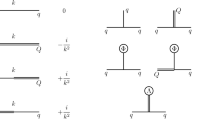
Abstract

We establish a general small data global existence and decay theorem for Chern–Simons theories with a general gauge group, coupled with a massive relativistic field of spin 0 or 1/2. Our result applies to a wide range of relativistic Chern–Simons theories considered in the literature, including the abelian/non-abelian self-dual Chern–Simons–Higgs equation and the Chern–Simons–Dirac equation. A key idea is to develop and employ a gauge invariant vector field method for relativistic Chern–Simons theories, which allows us to avoid the long-range effect of charge.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ao, W., Lin, C.-S., Wei, J.: On non-topological solutions of the \(A_2\) and \(B_2\) Chern–Simons system. Mem. Am. Math. Soc. 239(1132) (2016)

  2. Bergé, L., De Bouard, A., Saut, J.-C.: Blowing up time-dependent solutions of the planar. Chern–Simons gauged nonlinear Schrödinger equation. Nonlinearity 8(2), 235–253 (1995)

    MATH  Google Scholar 

  3. Bieri, L., Miao, S., Shahshahani, S.: Asymptotic Properties of Solutions of the Maxwell Klein Gordon Equation with Small Data. (2014). arXiv:1408.2550

  4. Bournaveas, N.: Low regularity solutions of the Chern–Simons–Higgs equations in the Lorentz gauge. Electron. J. Differ. Equ. 2009(114), 1–10 (2009)

    MathSciNet  MATH  Google Scholar 

  5. Bournaveas, N., Candy, T., Machihara, S.: A note on the Chern–Simons–Dirac equations in the Coulomb gauge. Discrete Cont. Dyn. Syst. A 34(7), 2693–2701 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  6. Brézis, H., Gallouet, T.: Nonlinear Schrödinger evolution equations. Nonlinear Anal. 4(4), 677–681 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bump, D.: Lie Groups, 2nd edn. Graduate Texts in Mathematics, vol. 225, Springer, New York (2013)

  8. Chae, D., Choe, K.: Global existence in the Cauchy problem of the relativistic Chern–Simons–Higgs theory. Nonlinearity 15(3), 747–758 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Chae, D.: Yu Imanuvilov, O.: The existence of non-topological multivortex solutions in the relativistic self-dual Chern–Simons theory. Commun. Math. Phys. 215, 119–142 (2000)

    Article  ADS  MATH  Google Scholar 

  10. Choe, K., Kim, N., Lin, C.-S.: Self-dual symmetric nontopological solutions in the SU(3) model in \({\mathbb{R}}^2\). Commun. Math. Phys. 334, 1–37 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  11. Christodoulou, D., Klainerman, S.: The Global Nonlinear Stability of the Minkowski Space. Princeton Mathematical Series, vol. 41, Princeton University Press, Princeton (1993)

  12. Delort, J.-M., Fang, D., Xue, R.: Global existence of small solutions for quadratic quasilinear Klein–Gordon systems in two space dimensions. J. Funct. Anal. 211(2), 288–323 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  13. Dunne, G.: Self-Dual Chern–Simons Theories, vol. 36. Springer, New York (1995)

    Book  MATH  Google Scholar 

  14. Dunne, G.: Mass degeneracies in self-dual models. Phys. Lett. B 345, 452–457 (1995)

    Article  ADS  MathSciNet  Google Scholar 

  15. Dunne, G.: Vacuum mass spectra for SU(4) self-dual Chern–Simons–Higgs systems. Nucl. Phys. B 433, 333–348 (1995)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  16. Gudnason, S.B.: Fractional and semi-local non-Abelian Chern–Simons vortices. Nucl. Phys. B 840, 160–185 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  17. Gudnason, S.B.: Non-Abelian Chern–Simons vortices with generic gauge groups. Nucl. Phys. B 821, 151–169 (2009)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  18. Hong, J., Kim, Y., Pac, P.Y.: Multivortex solutions of the Abelian Chern–Simons–Higgs theory. Phys. Rev. Lett 64, 2230 (1990)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Huang, H.-Y., Lin, C.-H.: On the entire radial solutions of the Chern–Simons SU(3) system. Commun. Math. Phys. 327, 815–848 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  20. Huh, H.: Cauchy problem for the fermion field equation coupled with the Chern–Simons gauge. Lett. Math. Phys. 79(1), 75–94 (2007)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  21. Huh, H.: Local and global solutions of the Chern–Simons–Higgs system. J. Funct. Anal. 242(2), 526–549 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  22. Huh, H.: Towards the Chern–Simons–Higgs equation with finite energy. Discrete Contin. Dyn. Syst. 30(4), 1145–1159 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  23. Huh, H., Oh, S.-J.: Low regularity solutions to the Chern–Simons–Dirac and the Chern–Simons–Higgs equations in the Lorenz gauge. Commun. Partial Differ. Equ. 41(3), 375–397 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  24. Jackiw, R., Weinberg, E.J.: Self-dual Chern–Simons vortices. Phys. Rev. Lett. 64, 2969 (1990)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  25. Jaffe, A., Taubes, C.: Vortices and Monopoles. Birkhäuser, Boston (1980)

    MATH  Google Scholar 

  26. Kao, H., Lee, K.: Self-dual SU(3) Chern–Simons–Higgs systems. Phys. Rev. D 50, 6626–6632 (1994)

    Article  ADS  MathSciNet  Google Scholar 

  27. Klainerman, S.: The null condition and global existence to nonlinear wave equations. In: Nonlinear Systems of Partial Differential Equations in Applied Mathematics, Part 1 (Santa Fe, N.M., 1984), Lectures in Applied Mathematics, vol. 23, pp. 293–326. American Mathematical Society, Providence (1986)

  28. Klainerman, S.: Global existence of small amplitude solutions to nonlinear Klein–Gordon equations in four space-time dimensions. Comm. Pure Appl. Math. 38(5), 631–641 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  29. Klainerman, S.: Uniform decay estimates and the Lorentz invariance of the classical wave equation. Commun. Pure Appl. Math. 38(3), 321–332 (1985)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Kobayashi, S., Nomizu, K.: Foundations of Differential Geometry, vol. 1. Wiley, New York (1963)

    MATH  Google Scholar 

  31. Kobayashi, S., Nomizu, K.: Foundations of Differential Geometry, vol. 2. Wiley, New York (1969)

    MATH  Google Scholar 

  32. Lin, C.-S., Yan, S.: Bubbling solutions for the SU(3) Chern–Simons model on a torus. Commun. Pure Appl. Math. 66, 991–1027 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  33. Lindblad, H., Rodnianski, I.: The global stability of Minkowski space-time in harmonic gauge. Ann. Math. 171(3), 1401–1477 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  34. Lindblad, H., Soffer, A.: A remark on asymptotic completeness for the critical nonlinear Klein–Gordon equation. Lett. Math. Phys. 73(3), 249–258 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  35. Lindblad, H., Soffer, A.: Scattering and small data completeness for the critical nonlinear Schrödinger equation. Nonlinearity 19(2), 345–353 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. Lindblad, H., Sterbenz, J.: Global Stability for Charged-Scalar Fields on Minkowski Space. International Mathematics Research Papers (IMRP) (2006)

  37. Liu, B., Smith, P.: Global Wellposedness of the Equivariant Chern–Simons–Schrödinger Equation. Rev. Mat. Iberoam. 32(3), 751–794 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  38. Liu, B., Smith, P., Tataru, D.: Local wellposedness of Chern–Simons–Schrödinger. Int. Math. Res. Not. 23, 6341–6398 (2014)

    MATH  Google Scholar 

  39. Lozano, G., Marques, D., Moreno, E., Schaposnik, F.: Non-abelian Chern–Simons theory. Commun. Math. Phys. 213, 599–639 (2000)

    Article  Google Scholar 

  40. Oh, S.-J.: Finite Energy Global Well-Posedness of the Chern–Simons–Higgs Equations in the Coulomb Gauge. (2013). arXiv:1310.3955

  41. Oh, S.-J.: Gauge choice for the Yang–Mills equations using the Yang–Mills heat flow and local well-posedness in \(H^1\). J. Hyperbolic Differ. Equ. 11(1), 1–108 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  42. Oh, S.-J.: Finite energy global well-posedness of the Yang–Mills equations on \(\mathbb{R}^{1+3}\): an approach using the Yang–Mills heat flow. Duke Math. J. 164(9), 1669–1732 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  43. Oh, S.-J., Pusateri, F.: Decay and scattering for the Chern–Simons–Schrödinger equations. Int. Math. Res. Not. 24, 13122–13147 (2015)

    Article  MATH  Google Scholar 

  44. Okamoto, M.: Well-posedness of the Cauchy problem for the Chern–Simons–Dirac system in two dimensions. J. Hyperbolic Differ. Equ. 10(4), 735–771 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  45. Pecher, H.: Low Regularity Local Well-Posedness for the Chern–Simons–Higgs System in Temporal Gauge. (2014). arXiv:1401.0145

  46. Pecher, H.: A Remark on Low Regularity Solutions of the Chern–Simons–Dirac System. (2014). arXiv:1409.4588

  47. Pecher, H.: Global Well-Posedness in Energy Space for the Chern–Simons–Higgs System in Temporal Gauge. J. Hyperbolic Differ. Equ. 13(2), 331–351 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  48. Psarelli, M.: Asymptotic behavior of the solutions of Maxwell–Klein–Gordon field equations in \(4\)-dimensional Minkowski space. Commun. Partial Differ. Equ. 24(1–2), 223–272 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  49. Psarelli, M.: Maxwell–Dirac equations in four-dimensional Minkowski space. Commun. Partial Differ. Equ. 30(1–3), 97–119 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  50. Selberg, S., Tesfahun, A.: Global Well-Posedness of the Chern–Simons–Higgs Equations with Finite Energy (2012). arXiv:1201.0975

  51. Speck, J.: The nonlinear stability of the trivial solution to the Maxwell-Born-Infeld system. J. Math. Phys. 53(8), 083703 (2012)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  52. Speck, J.: The global stability of the Minkowski spacetime solution to the Einstein-nonlinear system in wave coordinates. Anal. PDE 7(4), 771–901 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  53. Spruck, J., Yang, Y.: The existence of nontopological solitons in the self-dual Chern–Simons theory. Commun. Math. Phys. 149, 361–376 (1992)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  54. Spruck, J., Yang, Y.: Topological solutions in the self-dual Chern–Simons theory: existence and approximation. Ann. Inst. Henri Poincaré 12, 75–97 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  55. Yang, Y.: The relativistic non-Abelian Chern–Simons equations. Commun. Math. Phys. 186, 199–218 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  56. Uhlenbeck, K.: Connections with \(L^{p}\) bounds on curvature. Commun. Math. Phys. 83(1), 31–42 (1982)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  57. Wang, R.: The existence of Chern–Simons vortices. Commun. Math. Phys. 137, 587–597 (1991)

    Article  ADS  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Hankyung University, Anseong-si, Gyeonggi-do, Korea

    Myeongju Chae

  2. Korea Institute for Advanced Study, Seoul, Korea

    Sung-Jin Oh

Authors
  1. Myeongju Chae
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sung-Jin Oh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sung-Jin Oh.

Additional information

Communicated by James A. Isenberg.

The authors thank Hyungjin Huh for helpful discussions. M. Chae was partially supported by NRF-2015R1C1A2A01054919. A major part of this work was done, while S.-J. Oh was supported by the Miller Research Fellowship from the Miller Institute at UC Berkeley.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chae, M., Oh, SJ. Small Data Global Existence and Decay for Relativistic Chern–Simons Equations. Ann. Henri Poincaré 18, 2123–2198 (2017). https://doi.org/10.1007/s00023-016-0547-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00023-016-0547-9

Search

Navigation