Abstract
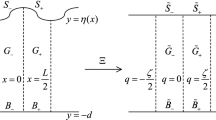
We discuss some aspects of the velocity field and particle trajectories beneath periodic traveling equatorial surface waves over a flat bed in a flow with uniform underlying currents. The system under study consists of the governing equations for equatorial ocean waves within a non-inertial frame of reference, where Euler’s equation of motion has to be suitably adjusted, in order to account for the influence of the earth’s rotation.
Article PDF
Similar content being viewed by others
Avoid common mistakes on your manuscript.
References
Chen, Y.-Y., Hsu, H.-C., Chen, G.-Y.: Lagrangian experiment and solution for irrotational finite-amplitude progressive gravity waves at uniform depth. Fluid Dyn. Res. 42, Art. No. 045511 (2010)
Constantin, A.: An exact solution for equatorially trapped waves. J. Geophys. Res. Oceans 117, Art. No. C05029 (2012)
Constantin, A.: On the modelling of equatorial waves. Geophys. Res. Lett. 39, Art. No. L05602 (2012)
Constantin A.: The trajectories of particles in Stokes waves. Invent. math. 166, 523–535 (2006)
Constantin A.: Some nonlinear, equatorially trapped, nonhydrostatic internal geophysical waves. J. Phys. Oceanogr. 44, 781–789 (2014)
Constantin, A., Escher, J.: Analyticity of periodic travelling free surface water waves with vorticity. Anal. Math. 173, 589–568 (2011)
Constantin A., Johnson R.S.: The dynamics of waves interacting with the equatorial undercurrent. Geophys. Astrophys. Fluid. Dyn. 109, 311–358 (2015)
Constantin, A., Johnson, R.S.: An exact, steady, periodic, purely azimuthal equatorial flow with a free surface. J. Phys. Oceanogr. doi:10.1175/JPO-D-15-0205.1 (in press)
Constantin A., Kalimeris K., Scherzer O.: A penalization method for calculating the flow beneath traveling water waves of large amplitude. SIAM J. Appl. Math. 75, 1513–1535 (2015)
Constantin A., Strauss W.: Pressure beneath a Stokes wave. Commun. Pure Appl. Math. 63, 533–557 (2010)
Clamond D.: Note on the velocity and related fields of steady irrotational two-dimensional surface gravity waves. Phil. Trans. R. Soc. A 370, 1572–1586 (2012)
Fedorov, A.V., Brown, J.N.: Equatorial waves. In: Steele, J. (ed.) Encyclopedia of Ocean Sciences, Academic Press, New York (2009)
Henry D.: An exact solution for equatorial geophysical water waves with an underlying current. Eur. J. Mech. B Fluids 38, 18–21 (2013)
Henry D.: Internal equatorial water waves in the f-plane. J. Nonlinear Math. Phys. 22, 499–506 (2015)
Henry D., Matioc A.-V.: On the symmetry of steady equatorial wind waves. Nonlinear Anal. Real World Appl. 18, 50–56 (2014)
Hsu H.-C.: An exact solution for equatorial waves. Monatsh. Math. 176, 143–152 (2015)
Ionescu-Kruse D.: An exact solution for geophysical equatorial edge waves in the \({\beta}\)-plane approximation. J. Math. Fluid Mech. 17, 699–706 (2015)
Johnson R.S.: An ocean undercurrent, a thermocline, a free surface, with waves: a problem in classical uid mechanics. J. Nonlinear Math. Phys. 22, 475–493 (2015)
Kogelbauer F.: Symmetric irrotational water waves are traveling waves. J. Differ. Equ. 259, 5271–5275 (2015)
Martin C.I.: Dynamics of the thermocline in the equatorial region of the Pacific ocean. J. Nonlinear Math. Phys. 22, 516–522 (2015)
Matioc, A.-V.: An exact solution for geophysical equatorial edge waves over a sloping beach. J. Phys. A 45, Art. No. 365501 (2012)
Quirchmayr R.: On the existence of benthic storms. J. Nonlinear Math. Phys. 22, 540–544 (2015)
Umeyama M.: Eulerian–Lagrangian analysis for particle velocities and trajectories in a pure wave motion using particle image velocimetry. Phil. Trans. R. Soc. A 370, 1687–1702 (2012)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by A. Constantin
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Quirchmayr, R. On Irrotational Flows Beneath Periodic Traveling Equatorial Waves. J. Math. Fluid Mech. 19, 283–304 (2017). https://doi.org/10.1007/s00021-016-0280-7
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00021-016-0280-7