Skip to main content
SpringerLink
Log in

An Improved Extrapolated Collocation Technique for Singularly Perturbed Problems using Cubic B-Spline Functions

  • Published:
Mediterranean Journal of Mathematics Aims and scope Submit manuscript

Abstract

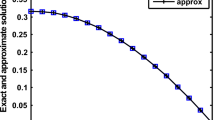
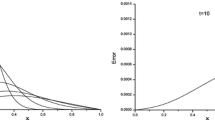
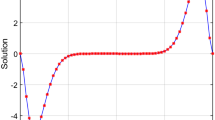
In this paper, an improved extrapolated collocation method with cubic B-spline as basis functions is presented. This method is applied to a class of self-adjoint singularly perturbed boundary value problems, with the equispaced discretization of the domain. As with the standard B-spline collocation method, the optimal order of convergence could not be achieved, so posteriori corrections are made in cubic B-spline interpolant and its higher-order derivatives. This results in the enhancement of order of convergence by several powers of step size ‘\({\hat{h}}\)’. The proposed technique has \(O({\hat{h}}^{6})\) convergence, which is established using Green’s function approach. A number of examples are solved numerically and the results are compared with the results available in the literature to illustrate the efficacy and reliability of the proposed numerical technique.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Kan-On, Y., Mimura, M.: Singular perturbation approach to a 3-component reaction-diffusion system arising in population dynamics. SIAM J. Math. Anal. 29(6), 1519–1536 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  2. Zhang, Y., Naidu, D.S., Cai, C., Zou, Y.: Singular perturbations and time scales in control theories and applications: an overview 2002–2012. Int. J. Syst. Sci. 9, 1–35 (2014)

    Google Scholar 

  3. McGough, J.S., Riley, K.L.: A priori bounds for reaction-diffusion systems arising in chemical and biological dynamics. Appl. Math. Comput. 163, 1–16 (2005)

    MathSciNet  MATH  Google Scholar 

  4. Andresen, P., Bache, M., Mosekilde, E., Dewel, G., Borckmanns, P.: Stationary spaceperiodic structures with equal diffusion coefficients. Phys. Rev. E 60, 297–301 (1999)

    Article  Google Scholar 

  5. Yaglom, A.M., Kader, B.A.: Heat and mass transfer between a rough wall and turbulent fluid flow at high Reynolds and Peclet numbers. J. Fluid Mech. 62, 601–623 (1974)

    Article  MATH  Google Scholar 

  6. Wasow, W.: On boundary layer problems in the theory of ordinary differential equations. Ph.D. Thesis, New York University (1941)

  7. Friedrichs, K.O., Wasow, W.: Singular perturbations of nonlinear oscillations. Duke Math. J. 13(3), 367–381 (1946)

    Article  MathSciNet  MATH  Google Scholar 

  8. O’Malley, R.E.: Introduction to Singular Perturbations. Academic Press, New York (1974)

    MATH  Google Scholar 

  9. Nayfeh, A.H.: Introduction to Perturbation Techniques. Wiley, New York (1981)

    MATH  Google Scholar 

  10. Smith, D.R.: Singular Perturbation Theory: An Introduction with Applications. Cambridge University Press, Cambridge (1985)

    MATH  Google Scholar 

  11. Miller, J.J.H.: Singular Perturbation Problems in Chemical Physics. Wiley, New York (1997)

    Google Scholar 

  12. Niijima, K.: On a three-point difference scheme for a singular perturbation problem without a first derivative term II. Mem. Numer. Math. 7, 11–27 (1980)

    MathSciNet  MATH  Google Scholar 

  13. Roberts, S.M.: A boundary-value technique for singular perturbation problems. J. Math. Anal. Appl. 87, 489–503 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  14. O’Riordan, E., Stynes, M.: A uniformly accurate finite element method for a singularly perturbed one-dimensional reaction-diffusion problem. Math. Comput. 47, 555–570 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  15. Gartland, E.C.: Graded-mesh difference schemes for singularly perturbed two-point boundary value problems. Math. Comput. 51, 631–657 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  16. Valanarasu, T., Ramanjam, N.: Asymptotic initial-value method for singularly perturbed boundary value problems for second order ordinary differential equations. J. Optim. Theory Appl. 116, 67–182 (2003)

    Article  MathSciNet  Google Scholar 

  17. Kopteva, N., Madden, N., Stynes, M.: Grid equidistribution for reaction diffusion problems in one dimension. Numer. Algor. 40, 305–322 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  18. Ramadan, M.A., Lashien, I.F., Zahra, W.K.: The numerical solution of singularly perturbed boundary value problems using nonpolynomial spline. Int. J. Pure Appl. Math. 41, 883–896 (2007)

    MathSciNet  MATH  Google Scholar 

  19. Kumar, M., Singh, P., Mishra, H.K.: A recent survey on computational techniques for solving singularly perturbed boundary value problems. Int. J. Comput. Math. 84, 1439–63 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  20. Roos, H.G., Stynes, M., Tobiska, L.: Robust Numerical Methods for Singularly Perturbed Differential Equations. Springer, Berlin (2008)

    MATH  Google Scholar 

  21. Mohapatra, J., Natesan, S.: Uniformly convergent numerical method for singularly perturbed differential difference equation using grid equidistribution. Int. J. Numer. Methods Biomed. Eng. 27, 1427–1445 (2011)

    MathSciNet  MATH  Google Scholar 

  22. Khuri, S.A., Sayfy, A.: Self-adjoint singularly perturbed boundary value problems: an adaptive variational approach. Math. Methods Appl. Sci. 36, 1070–1079 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  23. Zahra, W.K., El Mhlawy, A.M.: Numerical solution of two-parameter singularly perturbed boundary value problems via exponential spline. J. King Saud Univ. Sci. 25(3), 201–208 (2013)

    Article  Google Scholar 

  24. Brackbill, J.U.: An adaptive computational mesh for the solution of singular perturbation problems. J. Comput. Phys. 46, 342–368 (1982)

    Article  MathSciNet  Google Scholar 

  25. Kadalbajoo, M.K., Aggarwal, V.K.: Fitted mesh B-spline collocation method for solving self-adjoint singularly perturbed boundary value problems. Appl. Math. Comput. 161, 973–987 (2005)

    MathSciNet  MATH  Google Scholar 

  26. Kadalbajoo, M.K., Kumar, D.: Geometric mesh FDM for self-adjoint singular perturbation boundary value problems. Appl. Math. Comput. 190, 1646–1656 (2007)

    MathSciNet  MATH  Google Scholar 

  27. Vulanovic, R., Teofanov, L.: A modification of the Shishkin discretization mesh for one-dimensional reaction-diffusion problems. Appl. Math. Comput. 220, 104–116 (2013)

    MathSciNet  MATH  Google Scholar 

  28. Zahra, W.K.M.: Van Daele, M.: Uniformly convergent discrete spline scheme on a Shishkin mesh for the singular perturbation boundary value problem. In 15th International conference on Mathematical Methods in Science and Engineering 1261-1268. CMMSE (2015)

  29. Zahra, W.K., Van Daele, M.: Discrete Spline Solution of Singularly Perturbed Problem with Two Small Parameters on a Shishkin-Type Mesh. Comput. Math. Model. 29(3), 367–381 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  30. Doolan, E.P., Miller, J.J.H., Schilders, W.H.A.: Uniform Numerical Methods for Problems with Initial and Boundary Layers. Boole Press, Dublin (1980)

    MATH  Google Scholar 

  31. Boglaev, I.P.: A variational difference scheme for a boundary value problems with a small parameter in the highest derivative. USSR Comput. Math. Math. Phys. 21(4), 71–81 (1981)

    Article  MATH  Google Scholar 

  32. Schatz, A.H., Wahlbin, L.B.: On the finite element method for singularly perturbed reaction diffusion problems in two and one dimension. Math. Comput. 40, 47–89 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  33. Liu, S.T., Xu, Y.: Galerkin methods based on Hermite splines for singular perturbation problems. SIAM J. Numer. Anal. 43, 2607–2623 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  34. Roos, H.G., Skalický, T.: A comparison of the finite element method on Shishkin and Garlandtype meshes for convection diffusion problems. CWI Q. 10, 277–300 (1997)

    MATH  Google Scholar 

  35. Farrell, P.A., Hegarty, A., Miller, J.J.H., O’Riordan, E., Shishkin, G.I.: Robust Computational Techniques for Boundary Layers. Chapman and Hall, New York (2000)

    Book  MATH  Google Scholar 

  36. Kopteva, N., O’Riordan, E.: Shishkin meshes in the numerical solution of singularly perturbed differential equations. Int. J. Numer. Anal. Model. 7, 393–415 (2010)

    MathSciNet  MATH  Google Scholar 

  37. Ciarlet, P.G., Natterer, F., Varga, R.S.: Numerical methods of high-order accuracy for singular nonlinear boundary value problems. Numer. Math. 15(2), 87–99 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  38. Stojanović, M.: Splines difference methods for a singular perturbation problem. Appl. Numer. Math. 21(3), 321–333 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  39. Aziz, T., Khan, A.: A spline method for second-order singularly perturbed boundary-value problems. J. Comput. Appl. Math. 147, 445–452 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  40. Kadalbajoo, M.K., Patidar, K.C.: Numerical solution of singularly perturbed non-Linear two-point boundary value problems by spline in compression. Int. J. Comput. Math. 79, 271–88 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  41. Kadalbajoo, M.K., Arora, P.: B-splines with artificial viscosity for solving singularly perturbed boundary value problems. Math. Comput. Model. 52(5–6), 654–666 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  42. Prenter, P.M.: Splines and Variational Methods. Wiley, New York (1975)

    MATH  Google Scholar 

  43. Hall, C.A.: On error bounds for spline interpolation. J. Approx. Theory 1, 209–218 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  44. Ghasemi, M.: An efficient algorithm based on extrapolation for the solution of nonlinear parabolic equations. Int. J. Nonlinear Sci. Numer. Simul. 20(5), 527–541 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  45. Russell, R.D., Shampine, L.F.: A collocation method for boundary value problems. Numer. Math. 19(1), 1–28 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  46. Asrat, T., File, G., Aga, T.: Fourth-order stable central difference method for self-adjoint singular perturbation problems. Ethiop. J. Sci. Technol. 9(1), 53–68 (2016)

    Article  Google Scholar 

  47. Patidar, K.C., Kadalbajoo, M.K.: Exponentially fitted spline approximation method for solving self-adjoint singularly perturbed problem. Int. J. Math. Math. Sci. 61, 3873–3891 (2003)

    MATH  Google Scholar 

  48. Rao, S.C.S., Kumar, M.: Exponential B-spline collocation method for self-adjoint singularly perturbed boundary value problems. Appl. Numer. Math. 58, 1572–1581 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  49. Surla, K., Stojanović, M.: Solving singularly perturbed boundary-value problems by spline in tension. J. Comput. Appl. Math. 24(3), 355–363 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  50. Aziz, T., Khan, A.: Quintic spline approach to the solution of a singularly-perturbed boundary-value problem. J. Optim. Theory Appl. 112(3), 517–527 (2002)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

Ms. Shallu is thankful to CSIR New Delhi for providing financial assistance in the form of JRF with File No. 09/797(0016)/2018-EMR-I. Ms. Archna Kumari is thankful to MHRD New Delhi for providing financial assistance under the TEQIP-III project.

Author information

Authors and Affiliations

  1. Department of Mathematics, SLIET, Longowal, Punjab, 148106, India

    Shallu, Archna Kumari & Vijay Kumar Kukreja

Authors
  1. Shallu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Archna Kumari
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Vijay Kumar Kukreja
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vijay Kumar Kukreja.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shallu, Kumari, A. & Kukreja, V.K. An Improved Extrapolated Collocation Technique for Singularly Perturbed Problems using Cubic B-Spline Functions. Mediterr. J. Math. 18, 128 (2021). https://doi.org/10.1007/s00009-021-01738-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00009-021-01738-0

Keywords

Mathematics Subject Classification

Search

Navigation