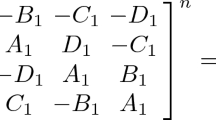Skip to main content
SpringerLink
Log in

On the Roots of Coquaternions

  • Published:
Advances in Applied Clifford Algebras Aims and scope Submit manuscript

A Correction to this article was published on 22 November 2018

This article has been updated

Abstract

In this paper we give a complete characterization of the nth roots of a coquaternion \(\mathsf {q}\). In particular, we show that the number and type of roots—isolated and/or hyperboloidal—depend on the nature of \(\mathsf {q}\), on the parity of n and (eventually) on the sign of the real part of \(\mathsf {q}\). We also show how the coquaternionic formalism can be used to obtain, in a simple manner, explicit expressions for the real nth roots of any \(2\times 2\) real matrix.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Change history

  • 22 November 2018

    Unfortunately, the reference Pogoruy [1] has been published with the incorrect Journal’s name.

References

  1. Alefeld, A., Scheider, N.: On square roots of \(M\)-matrices. Lin. Alg. Appl. 42, 119–132 (1982)

    Article  MathSciNet  Google Scholar 

  2. Björck, Å., Hammarling, S.: A Schur method for the square roots of a matrix. Lin. Alg. Appl. 52(53), 127–140 (1983)

    Article  MathSciNet  Google Scholar 

  3. Brody, D.C., Graefe, E.M.: On complexified mechanics and coquaternions. J. Phys. A: Math. Theory 44, 1–9 (2011)

    MathSciNet  MATH  Google Scholar 

  4. Cayley, A.: A memoir on the theory of matrices. Philos. Trans. R. Soc. London 148, 17–37 (1858)

    Article  ADS  Google Scholar 

  5. Choudhry, A.: Extraction of \(n\)th roots of \(2\times 2\) matrices. Lin. Alg. Appl. 387, 183–192 (2004)

    Article  MathSciNet  Google Scholar 

  6. Cockle, J.: On systems of algebra involving more than one imaginary; and on equations of the fifth degree. Philos. Mag. 35(3), 434–435 (1849)

    Google Scholar 

  7. Damphousse, P.: The arithmetic of powers and roots in \(GL_2({\mathbb{C}})\) and \(SL_2({\mathbb{C}})\). Fibonnacci Quart. 27, 386–401 (1989)

    MathSciNet  Google Scholar 

  8. Denman, E.D.: Roots of real matrices. Lin. Alg. Appl. 36, 133–139 (1981)

    Article  MathSciNet  Google Scholar 

  9. Falcão, M.I., Miranda, F., Severino, R., Soares, M.J.: Polynomials over quaternions and coquaternions: a unified approach. Lecture Notes Comput. Sci. 10405, 379–393 (2017)

    Article  MathSciNet  Google Scholar 

  10. Falcão, M.I., Miranda, F., Severino, R., Soares, M.J.: The number of zeros of unilateral polynomials over coquaternions revisited. Linear Multilinear Algebra. (2018). https://doi.org/10.1080/03081087.2018.1450828

  11. Falcão, M.I., Miranda, F., Severino, R., Soares, M.J.: Basins of attraction for a quadratic coquaternion map. Chaos Soliton. Fract. 104, 716–724 (2017)

    Article  ADS  Google Scholar 

  12. Falcão, M.I., Miranda, F., Severino, R., Soares, M.J.: The iteration of quadratic maps on coquaternions. Int. J. Bifurcat. Chaos 27(12), 1730039 (2017)

    Article  MathSciNet  Google Scholar 

  13. Frenkel, I., Libine, M.: Split quaternionic analysis and separation of the series for \(SL(2,{\mathbb{R}})\) and \(SL(2,{\mathbb{C}})/SL(2,{\mathbb{R}})\). Adv. Math. 228(2), 678–763 (2011)

    Article  MathSciNet  Google Scholar 

  14. Higham, N.H.: Computing real square roots of a real matrix. Lin. Alg. Appl. 88(89), 405–429 (1987)

    Article  MathSciNet  Google Scholar 

  15. Hoskins, W.D., Walton, D.J.: A faster method for computing the square root of a matrix. IEEE Trans. Automat. Control AC 23, 494–495 (1978)

    Article  Google Scholar 

  16. Kula, L., Yayli, Y.: Split quaternions and rotations in semi Euclidean space \(E^4_2\). J. Korean Math. Soc. 44, 1313–1327 (2007)

    Article  MathSciNet  Google Scholar 

  17. Özdemir, M.: The roots of a split quaternion. Appl. Math. Lett. 22(2), 258–263 (2009)

    Article  MathSciNet  Google Scholar 

  18. Özdemir, M., Ergin, A.: Rotations with unit timelike quaternions in Minkowski 3-space. J. Geometry Phys. 56(2), 322–336 (2006)

    Article  ADS  MathSciNet  Google Scholar 

  19. Pogoruy, A.A., Rodriguez-Dagnino, R.M.: Some algebraic and analytical properties of coquaternion algebra. Electron. Trans. Numer. Anal. 20(1), 79–84 (2010)

    MathSciNet  MATH  Google Scholar 

  20. Serôdio, R., Beites, P.D., Vitória, J.: Intersection of a double cone and a line in the split-quaternions context. Adv. Appl. Clifford Algebras 27, 2795–2803 (2017)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Centre of Mathematics, Department of Mathematics and Applications, University of Minho, Campus de Gualtar, 4710-057, Braga, Portugal

    M. Irene Falcão & Fernando Miranda

  2. Department of Mathematics and Applications, University of Minho, Campus de Gualtar, 4710-057, Braga, Portugal

    Ricardo Severino

  3. NIPE, Economic Policies Research Unit, Department of Mathematics and Applications, University of Minho, Campus de Gualtar, 4710-057, Braga, Portugal

    M. Joana Soares

Authors
  1. M. Irene Falcão
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Fernando Miranda
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ricardo Severino
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. M. Joana Soares
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Irene Falcão.

Additional information

Communicated by Rafał Abłamowicz.

Research at CMAT was financed by Portuguese Funds through FCT - Fundação para a Ciência e a Tecnologia, within the Project UID/MAT/00013/2013. Research at NIPE was carried out within the funding with COMPETE reference number POCI-01-0145-FEDER-006683 (UID/ECO/03182/2013), with the FCT/MEC’s (Fundação para a Ciência e a Tecnologia, I.P.) financial support through national funding and by the ERDF through the Operational Programme on “Competitiveness and Internationalization-COMPETE 2020” under the PT2020 Partnership Agreement.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Falcão, M.I., Miranda, F., Severino, R. et al. On the Roots of Coquaternions. Adv. Appl. Clifford Algebras 28, 97 (2018). https://doi.org/10.1007/s00006-018-0914-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00006-018-0914-3

Keywords

Mathematics Subject Classification

Search

Navigation