Skip to main content
SpringerLink
Log in

General two-sided quaternion Fourier transform, convolution and Mustard convolution

  • Published:
Advances in Applied Clifford Algebras Aims and scope Submit manuscript

Abstract

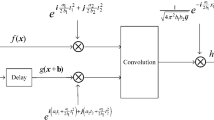
In this paper we use the general two-sided quaternion Fourier transform (QFT), and relate the classical convolution of quaternion-valued signals over \({{\mathbb R}^2}\) with the Mustard convolution. A Mustard convolution can be expressed in the spectral domain as the point wise product of the QFTs of the factor functions. In full generality do we express the classical convolution of quaternion signals in terms of finite linear combinations of Mustard convolutions, and vice versa the Mustard convolution of quaternion signals in terms of finite linear combinations of classical convolutions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bas, P.; Le Bihan, N.; Chassery, J.M.: Color image watermarking using quaternion Fourier transform. In: Acoustics, Speech, and Signal Processing, 2003. Proceedings (ICASSP03). IEEE International Conference on vol. 3, pp. III-521 (2003)

  2. Bayro-Corrochano E.: The theory and use of the quaternion wavelet transform. J. Math. Imaging Vis. 24, 19–35 (2006)

    Article  MathSciNet  Google Scholar 

  3. Bayro-Corrochano E., Trujillo N., Naranjo M.: Quaternion Fourier descriptors for the preprocessing and recognition of spoken words using images of spatiotemporal representations. J. Math. Imaging Vis. 28, 179–190 (2007)

    Article  MathSciNet  Google Scholar 

  4. Bülow, T.: Hypercomplex Spectral Signal Representations for the Processing and Analysis of Images. PhD Thesis, Univ. of Kiel (1999)

  5. Bujack R., De Bie H., De Schepper N., Scheuermann G.: Convolution products for hypercomplex Fourier transforms. J. Math. Imaging Vis. 48, 606–624 preprint: http://arxiv.org/abs/1303.1752 (2014)

  6. Coxeter H.S.M.: Quaternions and reflections. Am. Math. Mon. 53(3), 136–146 (1946)

    Article  MathSciNet  MATH  Google Scholar 

  7. De Bie H., De Schepper N., Ell T.A., Rubrecht K., Sangwine S.J.: Connecting spatial and frequency domains for the quaternion Fourier transform. Appl. Math. Comput. 271, 581–593 (2015)

    MathSciNet  Google Scholar 

  8. Denis P., Carré P., Fernandez-Maloigne C.: Spatial and spectral quaternionic approaches for colour images. Comput. Vis. Image Underst. 107, 74–87 (2007)

    Article  Google Scholar 

  9. Ell, TA.: Quaternionic-Fourier transform for analysis of two-dimensional linear time-invariant partial differential systems. In: Proceedings of the 32nd IEEE Conference on Decision and Control, December 15–17, vol. 2, pp. 1830–1841 (1993)

  10. Ell, TA., Sangwine, SJ.: Hypercomplex Fourier transforms of color images. IEEE Trans. Image Process. 16(1), 22–35 (2007)

  11. Guo C., Zhang L.: A novel multiresolution spatiotemporal saliency detection model and its applications in image and video compression. IEEE Trans. Image Process. 19, 185–198 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  12. Hildenbrand, D.: Foundations of Geometric Algebra Computing, Springer, Berlin (2013)

  13. Hitzer, E.: Quaternion Fourier transform on quaternion fields and generalizations. Adv. Appl. Clifford Algebra 17, 497–517 (2007). doi:10.1007/s00006-007-0037-8, preprint: http://arxiv.org/abs/1306.1023

  14. Hitzer, E.: Directional uncertainty principle for quaternion Fourier transforms, Adv. Appl. Clifford Algebra 20(2), 271–284 (2010). doi:10.1007/s00006-009-0175-2, preprint: http://arxiv.org/abs/1306.1276

  15. Hitzer, E.: OPS-QFTs: A new type of quaternion Fourier transforms based on the orthogonal planes split with one or two general pure quaternions. In: Numerical Analysis and Applied Mathematics ICNAAM 2011, AIP Conf. Proc. 1389, pp. 280–283 (2011). doi:10.1063/1.3636721, preprint: http://arxiv.org/abs/1306.1650

  16. Hitzer, E.: Creative Peace License. http://gaupdate.wordpress.com/2011/12/14/the-creative-peace-license-14-dec-2011/

  17. Hitzer, E.; Sangwine, SJ.: The orthogonal 2D planes split of quaternions and steerable quaternion Fourier transformations, in: E. Hitzer and S.J. Sangwine (Eds.), Quaternion and Clifford Fourier Transforms and Wavelets, Trends in Mathematics, vol. 27, pp. 15–40. Birkhäuser (2013) doi:10.1007/978-3-0348-0603-9_2, preprint: http://arxiv.org/abs/1306.2157

  18. Hitzer, E., Helmstetter, J., Abłamowicz, R.: Square Roots of 1 in Real Clifford Algebras. In: Hitzer, E., Sangwine S.J. (eds.) Quaternion and Clifford Fourier Transforms and Wavelets, Trends in Mathematics, vol. 27, pp. 123–153. Birkhäuser (2013). doi:10.1007/978-3-0348-0603-9_7, preprint: http://arxiv.org/abs/1204.4576

  19. Hitzer, E.: Two-Sided Clifford Fourier Transform with Two Square Roots of 1 in Cl(p, q). Adv. Appl. Clifford Algebras 24, 313–332 (2014). doi:10.1007/s00006-014-0441-9, preprint: http://arxiv.org/abs/1306.2092

  20. Hitzer, E.: The orthogonal planes split of quaternions and its relation to quaternion geometry of rotations. IOP J. Phys. Conf. Ser. (JPCS), 597, 012042 (2015). doi:10.1088/1742-6596/597/1/012042. Open Access URL: http://iopscience.iop.org/1742-6596/597/1/012042.pdf, preprint: http://arXiv.org/abs/1411.0362

  21. Jin L., Liu H., Xu X., Song E.: Quaternion-based impulse noise removal from color video sequences. IEEE Trans. Circ. Syst. Video Technol. 23, 741–755 (2013)

    Article  Google Scholar 

  22. Meister L., Schaeben H.: A concise quaternion geometry of rotations. Math. Meth. Appl. Sci. 28, 101–126 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  23. Moxey C.E., Sangwine S.J., Ell T.A.: Hypercomplex correlation techniques for vector images. IEEE Trans. Signal Process. 51, 1941–1953 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  24. Mustard D.: Fractional convolution. J. Aust. Math. Soc. Ser. B Vol. 40, 257–265 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  25. Sangwine S.J.: Color image edge detector based on quaternion convolution. Electron. Lett. 34, 969–971 (1998)

    Article  Google Scholar 

  26. Sangwine S.J.: Fourier transforms of colour images using quaternion, or hypercomplex, numbers. Electron. Lett. 32(21), 1979–1980 (1996)

    Article  Google Scholar 

  27. Sangwine, S.J., Le Bihan, N.: Quaternion and octonion toolbox for Matla. http://qtfm.sourceforge.net/. Accessed 29 Mar 2016

  28. Soulard R., Carré P.: Quaternionic wavelets for texture classification. Pattern Recognit. Lett. 32, 1669–1678 (2011)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Osawa 3-10-4, House M 472, 181-0015, Mitaka, Tokyo, Japan

    Eckhard Hitzer

Authors
  1. Eckhard Hitzer
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Eckhard Hitzer.

Additional information

Soli Deo Gloria.

In memory of Mrs. Lucy Baker, †31 December 2015, who worked with Seeds Of Hope Foundation, Mumbai, India. The use of this paper is subject to the Creative Peace License [16].

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hitzer, E. General two-sided quaternion Fourier transform, convolution and Mustard convolution. Adv. Appl. Clifford Algebras 27, 381–395 (2017). https://doi.org/10.1007/s00006-016-0684-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00006-016-0684-8

Keywords

Search

Navigation