Abstract
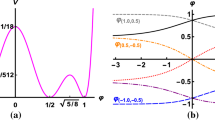
We study excitation spectra of BPS-saturated topological solutions — the kinks — of the φ8 scalar field model in (1 + 1) dimensions, for three different choices of the model parameters. We demonstrate that some of these kinks have a vibrational mode, apart from the trivial zero (translational) excitation. One of the considered kinks is shown to have three vibrational modes. We perform a numerical calculation of the kink-kink scattering in one of the considered variants of the φ8 model, and find the critical collision velocity vcr that separates the different collision regimes: inelastic bounce of the kinks at vin ≥ vcr, and capture at vin < vcr. We also observe escape windows at some values of vin < vcr where the kinks escape to infinity after bouncing off each other two or more times. We analyse the features of these windows and discuss their relation to the resonant energy exchange between the translational and the vibrational excitations of the colliding kinks.
Article PDF
Similar content being viewed by others
References
A. Vilenkin and E.P.S. Shellard, Cosmic strings and other topological defects, Cambridge University Press, Cambridge U.K. (2000).
N. Manton and P. Sutcliffe, Topological solitons, Cambridge University Press, Cambridge U.K. (2004).
T.I. Belova and A.E. Kudryavtsev, Solitons and their interactions in classical field theory, Phys. Usp. 40 (1997) 359 [Usp. Fiz. Nauk 167 (1997) 377].
G.L. Alfimov, A.S. Malishevskii and E.V. Medvedeva, Discrete set of kink velocities in Josephson structures: the nonlocal double sine-Gordon model, Physica D 282 (2014) 16.
P. Ahlqvist, K. Eckerle and B. Greene, Kink collisions in curved field space, JHEP 04 (2015) 059 [arXiv:1411.4631] [INSPIRE].
E. Greenwood, E. Halstead, R. Poltis and D. Stojkovic, Electroweak vacua, collider phenomenology, and possible connection with dark energy, Phys. Rev. D 79 (2009) 103003 [arXiv:0810.5343] [INSPIRE].
R. Poltis, Gravity waves seeded by turbulence and magnetic fields from a first order phase transition with non-renormalizable electroweak vacua, arXiv:1201.6362 [INSPIRE].
E. Braaten and L. Carson, Deuteron as a toroidal Skyrmion, Phys. Rev. D 38 (1988) 3525 [INSPIRE].
D. Foster and N.S. Manton, Scattering of nucleons in the classical Skyrme model, arXiv:1505.06843 [INSPIRE].
D. Foster and S. Krusch, Scattering of Skyrmions, Nucl. Phys. B 897 (2015) 697 [arXiv:1412.8719] [INSPIRE].
M.B. Hindmarsh and T.W.B. Kibble, Cosmic strings, Rept. Prog. Phys. 58 (1995) 477 [hep-ph/9411342] [INSPIRE].
E.J. Copeland and T.W.B. Kibble, Cosmic strings and superstrings, Proc. Roy. Soc. Lond. A 466 (2010) 623 [arXiv:0911.1345] [INSPIRE].
M. Quandt, N. Graham and H. Weigel, Quantum stabilization of a closed Nielsen-Olesen string, Phys. Rev. D 87 (2013) 085013 [arXiv:1303.0178] [INSPIRE].
O. Schroeder, N. Graham, M. Quandt and H. Weigel, Quantum stabilization of Z-strings, a status report on D = 3 + 1 dimensions, J. Phys. A 41 (2008) 164049 [arXiv:0710.4386] [INSPIRE].
H. Weigel, M. Quandt and N. Graham, Cosmic strings stabilized by fermion fluctuations, Int. J. Mod. Phys. A 27 (2012) 1260016 [arXiv:1111.4863] [INSPIRE].
R.V. Palvelev, Scattering of vortices in the abelian Higgs model, Theor. Math. Phys. 156 (2008) 1028 [Teor. Mat. Fiz. 156 (2008) 77] [INSPIRE].
J. Dziarmaga, More on scattering of Chern-Simons vortices, Phys. Rev. D 51 (1995) 7052 [hep-th/9412180] [INSPIRE].
E. Myers, C. Rebbi and R. Strilka, Study of the interaction and scattering of vortices in the Abelian Higgs (or Ginzburg-Landau) model, Phys. Rev. D 45 (1992) 1355 [INSPIRE].
P.M. Sutcliffe, BPS monopoles, Int. J. Mod. Phys. A 12 (1997) 4663 [hep-th/9707009] [INSPIRE].
R.S. Ward, Nontrivial scattering of localized solitons in a (2 + 1)−dimensional integrable system, Phys. Lett. A 208 (1995) 203.
L.P. Pitaevskii, On the momentum of solitons and vortex rings in a superfluid, JETP 119 (2014) 1097 [Zh. Eksp. Teor. Fiz. 146 (2014) 1252].
A.Y. Loginov, Rotating skyrmions of the (2 + 1)-dimensional Skyrme gauge model with a Chern-Simons term, JETP 118 (2014) 217 [Zh. Eksp. Teor. Fiz. 145 (2014) 250].
A.Y. Loginov, Bound fermion states in the field of a soliton of the nonlinear O(3) σ model, JETP Lett. 100 (2014) 346 [Pisma Zh. Eksp. Teor. Fiz. 100 (2014) 385] [INSPIRE].
S.W. Goatham, L.E. Mannering, R. Hann and S. Krusch, Dynamics of multi-kinks in the presence of wells and barriers, Acta Phys. Polon. B 42 (2011) 2087 [arXiv:1007.2641] [INSPIRE].
S.P. Popov, Influence of dislocations on kink solutions of the double sine-Gordon equation, Comput. Math. Math. Phys. 53 (2013) 1891 [Zh. Vychisl. Mat. Mat. Fiz. 53 (2013) 2072].
S.P. Popov, Interactions of breathers and kink pairs of the double sine-Gordon equation, Comput. Math. Math. Phys. 54 (2014) 1876 [Zh. Vychisl. Mat. Mat. Fiz. 54 (2014) 1954].
A.M. Gumerov, E.G. Ekomasov, F.K. Zakir’yanov and R.V. Kudryavtsev, Structure and properties of four-kink multisolitons of the sine-Gordon equation, Comput. Math. Math. Phys. 54 (2014) 491 [Zh. Vychisl. Mat. Mat. Fiz. 54 (2014) 481].
D. Saadatmand, S.V. Dmitriev, D.I. Borisov and P.G. Kevrekidis, Interaction of sine-Gordon kinks and breathers with a parity-time-symmetric defect, Phys. Rev. E 90 (2014) 052902 [arXiv:1408.2358].
D. Saadatmand et al., The effect of the ϕ 4 kink’s internal mode during scattering on PT-symmetric defect, JETP Lett. 101 (2015) 497 [Pisma Zh. Eksp. Teor. Fiz. 101 (2015) 550].
D. Saadatmand et al., Kink scattering from a parity-time-symmetric defect in the ϕ 4 model, Commun. Nonlinear Sci. Numer. Simulat. 29 (2015) 267 [arXiv:1411.5857].
Z. Fei, Y.S. Kivshar and L. Vazquez, Resonant kink-impurity interactions in the sine-Gordon model, Phys. Rev. A 45 (1992) 6019.
T.I. Belova and A.E. Kudryavtsev, Soliton interaction with an impurity in the λφ 42 theory, JETP 81 (1995) 817 [Zh. Eksp. Teor. Fiz. 108 (1995) 1489].
T.S. Mendonça and H.P. de Oliveira, The collision of two-kinks defects, arXiv:1502.03870 [INSPIRE].
T.S. Mendonça and H.P. de Oliveira, A note about a new class of two-kinks, JHEP 06 (2015) 133 [arXiv:1504.07315] [INSPIRE].
M.A. Lohe, Soliton structures in P (φ)2, Phys. Rev. D 20 (1979) 3120 [INSPIRE].
S.V. Pavlov, M.L. Akimov, Phenomenological theory of isomorphous phase transitions, Crystall. Rep. 44 (1999) 297.
E.B. Bogomolny, Stability of classical solutions, Sov. J. Nucl. Phys. 24 (1976) 449 [Yad. Fiz. 24 (1976) 861].
M.K. Prasad and C.M. Sommerfield, Exact classical solution for the ’t Hooft monopole and the Julia-Zee dyon, Phys. Rev. Lett. 35 (1975) 760 [INSPIRE].
A. Khare, I.C. Christov and A. Saxena, Successive phase transitions and kink solutions in ϕ 8 , ϕ 10 and ϕ 12 field theories, Phys. Rev. E 90 (2014) 023208 [arXiv:1402.6766] [INSPIRE].
P. Dorey, K. Mersh, T. Romanczukiewicz and Y. Shnir, Kink-antikink collisions in the ϕ 6 model, Phys. Rev. Lett. 107 (2011) 091602 [arXiv:1101.5951] [INSPIRE].
V.A. Gani and A.E. Kudryavtsev, Kink-antikink interactions in the double sine-Gordon equation and the problem of resonance frequencies, Phys. Rev. E 60 (1999) 3305 [cond-mat/9809015] [INSPIRE].
D. Campbell, M. Peyrard and P. Sodano, Kink-antikink interactions in the double sine-Gordon equation, Physica D 19 (1986) 165.
M. Peyrard and D. Campbell, Kink-antikink interactions in a modified sine-Gordon model, Physica D 9 (1983) 33.
V.A. Gani, A.E. Kudryavtsev and M.A. Lizunova, Kink interactions in the (1 + 1)-dimensional ϕ 6 model, Phys. Rev. D 89 (2014) 125009 [arXiv:1402.5903] [INSPIRE].
A.E. Kudryavtsev, Solitonlike solutions for a Higgs scalar field, JETP Lett. 22 (1975) 82 [Pisma Zh. Eksp. Teor. Fiz. 22 (1975) 178].
Open Access
This article is distributed under the terms of the Creative Commons Attribution License (CC-BY 4.0), which permits any use, distribution and reproduction in any medium, provided the original author(s) and source are credited.
Author information
Authors and Affiliations
Corresponding author
Additional information
ArXiv ePrint: 1506.02313
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (https://creativecommons.org/licenses/by/4.0), which permits use, duplication, adaptation, distribution, and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Gani, V.A., Lensky, V. & Lizunova, M.A. Kink excitation spectra in the (1+1)-dimensional φ8 model. J. High Energ. Phys. 2015, 147 (2015). https://doi.org/10.1007/JHEP08(2015)147
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/JHEP08(2015)147