Abstract
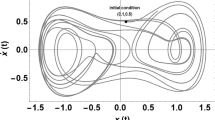
A chaos maximizing optimal control problem is formulated and applied to Duffing's equation to maximize the largest Lyapunov exponent. The resulting bang-bang optimal controller yields a positive value of the largest Lyapunov exponent, indicating chaotic behavior. In fact, the largest Lyapunov exponent is approximately twice as large as that achieved with simple sinusoidal forcing at the same amplitude bounds. However, the resulting phase portrait of the optimal trajectory is a limit cycle and is not chaotic at all. This paradoxical result contradicts the basic theory that a bounded trajectory with at least one positive Lyapunov exponent must be chaotic. Details concerning the development of a chaos measurement that is viable for current optimal control theory, a method of continuous normalization, the paradoxical chaotic limit cycle, resolution of the paradox, and closed-loop optimal jump condition in an augmented space are presented. In particular, for systems of differential equations with only piecewise differentiable right-hand sides due to a switching control, a jump discontinuity condition must be imposed on the state perturbations in order to compute correct Lyapunov exponents.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Brockett, R. W., 1982, “On Conditions Leading to Chaos in Feedback Systems”,Proc. IEEE Conf. on Decision and Control, pp. 932–936.
Cook, P. A., 1985, “Simple Feedback Systems with Chaotic Behavior,”Systems and Control Letters, Vol. 6, No. 4, pp. 223–227.
Cook, P. A., 1986, “Chaotic Behavior in Feedback Systems”,Proc. of 25th Conf. on Decision and Control, Athens, Greece, pp. 1151–1154.
Haken, H., 1983, “At Least One Lyapunov Exponent Vanishes if the Trajectory of an Attractor Does Not Contain a Fixed Point”,Physics Letters, Vol. 94A, No. 2, pp. 71–72.
Kaplan, J. L. and Yorke, J. A., 1979, “Chaotic Behavior of Multidimensional Difference Equations”,Lecture Notes in Mathematics, No. 730, pp. 204–227.
Lee, B., August 1991, “Chaos in an Optimal Control System”, Ph. D. Dissertation. Department of Mechanical and Materials Engineering. Washington State University, Pullman, WA 99163.
Lee, B., Grantham, W. J. and Fisher, M., 1994, “Differential Equation for Continuous Normalization”,KSME J., Vol. 8, No. 1, pp. 63–68.
Grantham, W. J. and Lee, B., 1993, “A Chaotic Limit Cycle Paradox,”Dynamics and Control, Vol. 3, pp. 159–173.
Leitmann, G., 1981,The Calculus of Variations and Optimal Control: An Introduction, Plenum, New York, pp. 247–222.
Lorenz, E. N., March 1963, “Deterministic Nonperiodic Flow,”J. of the Atmospheric Sciences, Vol. 20, pp. 130–141.
Pontryagin, L. S., et. al., 1964,The Mathematical Theory of Optimal Processes, Pergamon Press, New York.
Rubio, F. R., Aracil, J. and Camacho, E. F., 1985, “Chaotic Motion in an Adaptive Control System,”Int. J. Control, Vol. 42, No. 2, pp. 353–360.
Shampine, L. F. and Gordon, M. K., 1975,Computer Solution of Ordinary Differential Equations: The Initial Value Problem, Freeman, W. H., San Francisco.
Ushio, Toshimitsu and Hirai, Kazumasa, 1983, “Chaos in Non-Linear Sampled-Data Control Systems,”Int. J. Control, Vol. 38, No. 5, pp. 1023–1033.
Wolf, A., Swift, J., Swinney, H. and Vastano, A., 1985, “Determining Lyapunov Exponents from a Time Series,”Physica 16D, pp. 285–317.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Lee, B. Chaos maximizing optimal control. KSME Journal 9, 397–409 (1995). https://doi.org/10.1007/BF02953638
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02953638