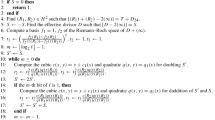Skip to main content
SpringerLink
Log in

Mordell-Weil groups and Selmer groups of twin- prime elliptic curves

  • Published:
Science in China Series A: Mathematics Aims and scope Submit manuscript

Abstract

LetE=E σ :y 2 =x(x+σp)(x+σq) be elliptic curves, where σ= ±1,p andq are prime numbers withp + 2= q. (i) Selmer groups S2(E/Q), S03D5(E/Q), and\(S^{\widehat{(\varphi )}} \left( {E/Q} \right)\) are explicitly determined, e.g. S2(E+1/Q)= (Z/2Z)2, (Z/2Z)3, and (Z/2Z)4 when p ≡ 5, 1 (or 3), and 7(mod 8), respectively. (ii) Whenp ≡ 5 (3, 5 for σ= ?1) (mod 8), it is proved that the Mordell-Weil group E(Q) ≅ Z/2Z ⊕ Z/2Z, rankE(Q) = 0, and Shafarevich-Tate group III (E/Q)[2]= 0. (iii) In any case, the sum of rankE(Q) and dimension of III (E/Q)[2] is given, e.g. 0, 1, 2 whenp ≡ 5, 1 (or 3), 7 (mod 8) for σ= 1. (iv) The Kodaira symbol, the torsion subgroup E(K)tors for any number fieldK, etc. are also obtained.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bremner, A., On the equation y2 = x(x 2 + p), in Number Theory and Applications (ed. Mollin, R. A.), Dordrecht: Kluwer, 1989, 3–23.

    Google Scholar 

  2. Bremner, A., Cassels, J. W. S., On the equation y2 = x(x2 + p), Math. Comp., 1984, 42: 257–264.

    Article  MathSciNet  Google Scholar 

  3. Stroeker, R. J., Top, J., On the equation y2 = (x + p)(x2 + p2), Rocky Mountain J. of Math., 1994, 24: 1135–1161.

    Article  MathSciNet  MATH  Google Scholar 

  4. Silverman, J. H., The Arithmetic of Elliptic Curves, GTM 106, Berlin: Springer-Verlag, 1986.

    MATH  Google Scholar 

  5. Silverman, J. H., The Advanced Topics in the Arithmetic of Elliptic Curves, GTM 151, Berlin: Springer- Verlag, 1994.

    MATH  Google Scholar 

  6. Tate, J., Algorithm for determining the type of singular fiber in an elliptic pencil, in Modular Functions of One Variable IV, Lect. Notes Math. 476, Berlin: Springer-Verlag, 1975, 33–52.

    Google Scholar 

  7. Cremona, J. E., Algorithms for Modular Elliptic Curves, Cambridge: Cambridge Univ. Press, 1992.

    MATH  Google Scholar 

  8. Cassels, J. W. S., Lectures on Elliptic Curves, LMS Student Texts, Cambridge: Cambridge Univ. Press, 1991.

    Google Scholar 

  9. Merriman, J. R., Siksek, S., Smart, N. P., Explicit 4-descents on an elliptic curve, Acta Arithmetica, 1996, LXXVII(4): 385–404.

    MathSciNet  Google Scholar 

  10. Qiu, D. R., Zhang, X. K., Elliptic curves and their torsion subgroups over number fields of type (2, 2, ⋯, 2), Science in China, Ser. A, 2001, 44(2): 159–167.

    Article  MathSciNet  MATH  Google Scholar 

  11. Müller, H. H., Ströher, H., Zimmer, H. G., Torsion subgroups of elliptic curves with integral j-invariant over quadratic fields, J. Reine Angew. Math., 1989, 397: 100–161.

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Center for Advanced Study, Tsinghua University, 100084, Beijing, China

    Qiu Derong

  2. Department of Mathematical Sciences, Tsinghua University, 100084, Beijing, China

    Zhang Xianke

Authors
  1. Qiu Derong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhang Xianke
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhang Xianke.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Derong, Q., Xianke, Z. Mordell-Weil groups and Selmer groups of twin- prime elliptic curves. Sci. China Ser. A-Math. 45, 1372–1380 (2002). https://doi.org/10.1007/BF02880031

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02880031

Keywords

Search

Navigation