A semi-classical approximation for two-dimensional scattering by nonseparable potentials

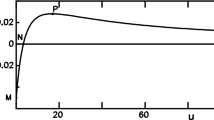
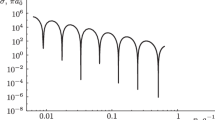
Summary

The Schrödinger equation for the sum of a central and a noncentral potential is solved approximately by wirting the wave function in terms of two variable quantities, a real phase and a complex amplitude. The variable phase satisfies the Hamilton-Jacobi equation which contains the central part of the potential only and, therefore, the phase is expressible as a definite integral. The complex amplitude, on the other hand, satisfies a nonlinear partial differential equation in which the noncentral part of the potential appears. This amplitude is calculated approximately first by using the technique of quasi-linearization and then by solving the resulting linear equation by the method of characteristics. In this way the asymptotic phase and the amplitude of the wave function are obtained from the recursive solution of a set of linear differential equations. The method has been applied to find the scattering phase shifts for several separable and nonseparable potential functions.

Riassunto

Si risolve l’equazione di Schrödinger per la somma di un potenziale centrale e di uno non centrale in maniera approssimativa scrivendo la funzione d’onda in termini di due quantità variabili, una fase reale e un’ampiezza complessa. La fase variabile soddisfa l’equazione di Hamilton-Jacobi che contiene la parte centrale del potenziale solamente,e quindi la fase è esprimibile come un integrale definito. L’ampiezza complessa, d’altra parte, soddisfa un’equazione differenziale parziale non lineare in cui compare la parte non centrale del potenziale. Quest’ampiezza è calcolata in maniera approssimativa, prima usando la tecnica di quasi linearizzazione e poi risolvendo l’equzione lineare risultante per mezzo del metodo delle caratteristiche. In questo modo si ottengono la fase asimptotica e l’ampiezza della funzione d’onda dalla soluzione ricorsiva di un sistema di equazioni differenziali lineari. Il metodo è stato applicato per trovare gli spostamenti di fase dello scattering per parecchie funzioni di potenziali separabili e non separabili.

Аннотация

Приближенно решается уравнение Шредингера для суммы центрального и нецентрального потенциалов, записывая волновую функцию в терминах двух переменных, реальной фазы и комплексной амплитуды. Переменная фаза удовлетворяет уравнению Гамильтона-Якоби, которое содержит только центральную льную насть потенциала, и, следовательно, фаза выражается в виде определенного интеграла. С другой стороны, комплексная амплируда удовлетворяет нелинейному дифференциальному уравнению в частных производных, в котором содержится нецентральная часть потенциала. Сначала приближенно вычисляется амплитуда, используя технику квазилинеаризации, а затем решается полученное линейное уравнение методом характеристик. Таким образом, получаются асимптоическая фаза и амплитуда волновой функции из рекурентного решения системы линейных дифференциальных уравнений. Предложенный метод применяется для нахождения фазовых сдвигов рассеяния для некоторых разделяемых и неразделяемых потенциальных функций.