Skip to main content
SpringerLink
Log in

Individually shaped volume conductor models of the head in EEG source localisation

  • Modelling
  • Published:
Medical and Biological Engineering and Computing Aims and scope Submit manuscript

Abstract

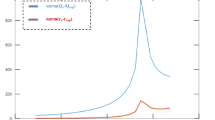
Inverse solution techniques based on electroencephalograph (EEG) measurements have become a powerful means of gaining knowledge about the functioning of the brain. A model of the head and a potential computation method are necessary to describe the EEG problem mathematically. The generation of realistically shaped three-compartment models of the head is discussed. The isolated problem approach for the boundary element method is applied to develop a fast and accurate numerical solution of the EEG forward problem. Accuracy studies with this approach show that dipole positions can be reconstructed within a distance of 3 mm from the original positions. Inverse simulations indicate that the incorporation of the individual head shape may significantly influence the reconstructed dipole position but not its magnitude and orientation, in comparison with the commonly used three-sphere model. However, the presence of noise in the simulated potential data affects the solutions based on realistically shaped models more than those of the simple three-sphere model. The increased censitivity of the former models to noise in the data remains a serious drawback for their practical application to EEG source localisations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Barr, C., Ramsey, III, M., andSpach, M. S. (1977): ‘Relating epicardial to body surface potential distributions by means of transfer coefficients based on geometry measurements’,IEEE Trans.,BME-24, (1), pp. 1–11

    MathSciNet  Google Scholar 

  • Meljs, J. W. H., Weier, O. W., Peters, M. J., andvan Oosterom, A. (1989): ‘On the numerical accuracy of the boundary element method’,IEEE Trans.,BME-36, (10), pp. 1038–1049

    Google Scholar 

  • Bertrand, O., Thevenet, M., andPerrin, F. (1991): ‘3D finite element method in brain electrical activity studies’in Nenonen, J., Rajala, H. M., andKatila, T. (Eds.) ‘Biomagnetic localization and 3D modelling’. Helsinki University of Technology, Department of Technical Physics, Report TKK-F-A689, pp. 154‐171

  • Cuffin, B. N. (1990): ‘Effects of head shape on EEG’s and MEG’s’,IEEE Trans.,BME-37, (1), pp. 44–52

    Google Scholar 

  • de Munck, J. C. (1992): ‘A linear discretization of the volume conductor boundary integral equation using analytically integrated elements’,IEEE Trans.,BME-39, (9), pp. 986–990

    Google Scholar 

  • Engeln-Müllges, G., andReutter, F. (1990): ‘Formelsammlung zur numerischen Mathematik mit C-Programmen’ (BI Widdenschaftsverlag, Mannheim/Wien/Zürich) pp. 245–270

    MATH  Google Scholar 

  • Geselowitz, D. B. (1967): ‘On the bioelectric potentials in an inhomogeneous volume conductor’,Biophys. J.,7, pp. 1–11

    Article  Google Scholar 

  • Hämäläinen, M. S., andSarvas, J. (1989): ‘Realistic conductivity geometry model of the human head for interpretation of neuromagnetic data’,IEEE Trans.,BME-36, (2), pp. 165–171

    Google Scholar 

  • Heller, L. (1990): ‘Computation of the return current in encephalography: the auto solid angle’in Gmitro, A. F., Idell, P. S., andLaHaie, I. J. (Eds.): ‘Digital imaging and inverse optics’. Proc. SPIE 1251, pp. 376–390

  • Lynn, M. S., andTimlake, W. P. (1967): ‘The use of multiple deflations in the numerical solution of singular systems of equations, with applications to potential theory’,SIAM J. Num. Anal.,5, (2), pp. 303–322

    Article  MathSciNet  Google Scholar 

  • Mosher, J. C., Lewis, P. S., andLeahy, R. M. (1992): ‘Multiple diode modeling and localization from spatio-temporal MEG data’,IEEE Trans.,BME-39, (6), pp. 541–557

    Google Scholar 

  • Roth, B. J., Balish, M., Gorbach, A., andSato, S. (1993): ‘How well does a three-sphere model predict positions of dipoles in a realistically shaped head?’Electroenceph. Clin. Neurophysiol.,87, pp. 175–184

    Article  Google Scholar 

  • Wieringa, H. J. (1993): ‘MEG, EEG and the integration with magnetic resonance images’. PhD Thesis, University of Twente, The Netherlands

    Google Scholar 

  • Wieringa, H. J., andPeters, M. J. (1993): ‘Processing MRI data for electromagnetic source imaging’,Med. Biol. Eng. Comput., 1993,31, pp. 600–606

    Article  Google Scholar 

  • Zhou, H., andvan Oosterom, A. (1992): ‘Computation of the potential distribution in a four-layer anisotropic concentric spherical volume conductor’,IEEE Trans.,BME-39, (2), pp. 154–158

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Applied Physics, University of Twente, PO Box 217, 7500 AE, Enschede, The Netherlands

    F. Zanow & M. J. Peters

Authors
  1. F. Zanow
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. J. Peters
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zanow, F., Peters, M.J. Individually shaped volume conductor models of the head in EEG source localisation. Med. Biol. Eng. Comput. 33, 582–588 (1995). https://doi.org/10.1007/BF02522518

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02522518

Keywords

Search

Navigation