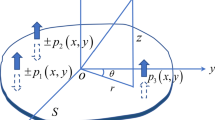
Abstract
The penny-shaped cracks periodically distributed in infinite elastic body are studied. The problem is approximately simplified to that of a single crack embedded in finite length cylinder and the stress intensity factor is obtained by solving a Fredholm integral equation. Numerical results are given and the effects of crack interaction on the stress intensity factor are discussed.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Sneddon I N and Lowengrub M. Crack Problems in the Classical Theory of Elasticity. John Wiley and Sons, 1969
Sneddon I N. Int J Engng Sci, 1963, 1: 391–409
Nied H A and Arin K Fracture Mechanics of Ceramics. N. Y.: Plenum Press, 1977. Vol. 3, 67–83
Sneddon I N. Mixed boundary value problems in potential theory. North Holland Publishing Co., 1966
Sneddon I N and Srivastav R P. Proc Royal Society of Edinburgh. Sect A, 1964, 66: 150–172
Gradshteyn I S and Ryzhik I M. Tables of Integrals, Series and Products. Academic Press, 1980
Zhou Jianping. The Doctor Thesis of Engineering Science, National Univ of Defence Tech, Oct. 1989 (in Chinese)
Author information
Authors and Affiliations
Additional information
The project suppoted by National Natural Science Foundation of China
Rights and permissions
About this article
Cite this article
Jianping, Z., Yinchu, L. The approximate solution of penny-shaped cracks periodically distributed in infinite elastic body. Acta Mech Sinica 8, 61–68 (1992). https://doi.org/10.1007/BF02486917
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02486917