Skip to main content
SpringerLink
Log in

The perturbed Korteweg-de Vries equation: Evolution of solitons

  • Published:
Il Nuovo Cimento D

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

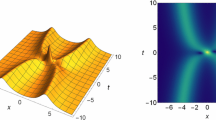
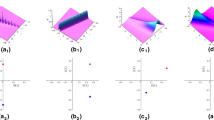
Summary

In this paper we examine the dynamic of solitons in the presence of external forces expressed by an-degree polynomial perturbative term or by a combination of polynomial and differential terms in the dependent variable. Under the action of these forces the soliton profile will no longer be a simple translation: asymptotic behaviour of the wave amplitude to threshold values (stationary equilibrium states) are now possible and “explosions” may occur at some finite “critical time” at which the soliton amplitude becomes infinite.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. C. S. Gardner, J. M. Green, M. D. Kruskal andR. M. Miura:Phys. Rev. Lett.,19, 1095 (1967).

    Article  MATH  ADS  Google Scholar 

  2. C. S. Gardner, J. M. Green, M. D. Kruskal andR. M. Miura:Comments Pure Appl. Math.,27, 97 (1974).

    MATH  Google Scholar 

  3. R. M. Miura:SIAM Review,18, 412 (1976).

    Article  MATH  MathSciNet  Google Scholar 

  4. W. Eckhaus andA. Van Harten:The Inverse Scattering Transformation and the Theory of Solitons (North Holland, Amsterdam 1981).

    Google Scholar 

  5. G. L. Lamb Jr.:Elements of Soliton Theory(Wiley Interscience, New York, N.Y., 1980).

    Google Scholar 

  6. P. G. Drazin:Solitons (Cambridge University Press, Cambridge, 1983).

    Google Scholar 

  7. G. B. Whitham:Linear and Nonlinear Waves (J. Wiley, New York, N.Y., 1974).

    Google Scholar 

  8. V. I. Karpmann andM. S. Maslov:Sov. Phys. JETP,46, 281 (1977).

    Google Scholar 

  9. V. I. Karpmann andM. S. Maslov:Sov. Phys. JETP,48, 252 (1978).

    Google Scholar 

  10. V. I. Karpmann:Phys. Lett. A,66, 13 (1978).

    Article  MathSciNet  ADS  Google Scholar 

  11. V. I. Karpmann:Phys. Scri.,20, 462 (1979).

    ADS  Google Scholar 

  12. J. Engelbrecht andA. Jeffrey:Wave Motion,9, 533 (1987).

    Article  MATH  Google Scholar 

  13. J. Engelbrecht andY. Khamidullin:Phys. Earth Planet. Inter.,50, 39 (1988).

    Article  ADS  Google Scholar 

  14. J. Engelbrecht:Wave Motion,14, 85 (1991).

    Article  MATH  MathSciNet  Google Scholar 

  15. L. A. Ostrovsky:Soliton in Active Media, inProceeding of the IUTAM Symposium on Nonlinear Deformation Waves, Tallin 1982, edited byU. Nigul andJ. Engelbrecht, (Springer, Berlin, 1983), p. 30.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica dell'Università di Roma «La Sapienza», Roma, Italia

    N. Virgopia & F. Ferraioli

Authors
  1. N. Virgopia
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Ferraioli
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Virgopia, N., Ferraioli, F. The perturbed Korteweg-de Vries equation: Evolution of solitons. Il Nuovo Cimento D 14, 821–832 (1992). https://doi.org/10.1007/BF02456686

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02456686

PACS 47.10

PACS 47.35

Search

Navigation