Abstract
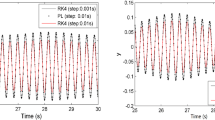
By taking example of the 2D Navier-Stokes equations, a kind of improved version of the nonlinear Galerkin method of Marion-Temam type based on the new concept of the inertial manifold with delay (IMD) is presented, which is focused on overcoming the defect that the feasibility of the M-T type nonlinear Galerkin method heavily depended on the least solving scale. It is shown that the improved version can greatly reduce the feasible conditions as well as preserve the superiority of the former version. Therefore, the version obtained here is an applicable, high performance and stable algorithm.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Foias C, Sell G R, Temam R. Inertial manifolds for nonlinear evolutionary equations[J].J Diff Eqs, 1998,73(2):309–353.
Foias C, Manley O, Temam R. On the interaction of small eddies in two-dimensional turbulence flows[J].Math Modeling and Numerical Analysis, 1998,22:93–114.
Marion M, Temam R. Nonlinear Galerkin methods[J].SIAM J Numer Anal, 1989,21(5): 1139–1157.
Marion M, Temam R. Nonlinear Galerkin methods: the finite elements case[J].Numer Math, 1990,57(3):205–226.
Marion M, XU Jin-chao. Error estimates on a new nonlinear Galerkin method based on two-grid finite elements[J].SIAM J Numer Anal, 1995,32(4):1170–1184.
SHEN Jie, Temam R. Nonlinear Galerkin method using Chebyshev and Legendre polynomials I: the one-dimensional case[J].SIAM J Numer Anal, 1995,32(1):215–234.
LI Kai-tai, HOU Yan-ren. Fourier nonlinear Galerkin method for the Navier-Stokes equations[J].Discrete and Continuous Dynamical Systems, 1996,2(4):497–524.
Dubois T, Jauberteau F, Marion M,et al. Subgrid modelling and the interaction of small and large wavelengths in turbulent flows[J].Computer Physics Communications, 1991,65(1–3):100–106.
Debussche A, Temam R, Inertial manifolds with delay[J].Applied Math Letters, 1995,8(1): 21–24.
LI Kai-tai, HOU Yan-ren. Inertial manifold with delay and the family of approximate inertial manifold with delay[J].Acta Mathematica Sinica, 2000,43(3):435–444. (in Chinese)
Temam R,Navier-Stokes Equations[M]. 3rd Edition. Amsterdam: North-Holland, 1984.
Canuto C, Hussaini M Y, Quarteroni A,et al.Spectral Methods in Fluid Dynamics[M]. New York-Heidelberg-Berlin: Springer-Verlag, 1987.
Author information
Authors and Affiliations
Additional information
Communicated by ZHANG Hong-qing
Foundation items: the National Natural Science Foundation of China (19671067); the Special Foundation for Major State Basic Research (973) Projects (G1999032801-01-5)
Biography: HOU Yun-ren (1969-)
Rights and permissions
About this article
Cite this article
Yan-ren, H., Kai-tai, L. IMD based nonlinear Galerkin method. Appl Math Mech 24, 326–337 (2003). https://doi.org/10.1007/BF02438270
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF02438270