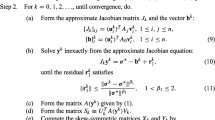Abstract
The Gauss-Newton step belonging to an appropriately chosen bordered nonlinear system is analyzed. It is proved that the Gauss-Newton step calculated after a sequence of Newton steps is equal to the doubled Newton step within the accuracy ofO(‖x−x *‖2). The theoretical insight given by the proof can be exploited to derive a Gauss-Newton-like algorithm for the solution of singular equations.
Zusammenfassung
In der Arbeit wird der Gauß-Newton-Schriftt für ein geeignet gewähltes erweitertes nichtlineares System analysiert. Es wird bewiesen, daß ein im Anschluß an eine Folge von Newton-Schritten berechneter Gauß-Newton-Schritt einem doppelten Newton-Schritt mit einer Genauigkeit vonO(‖x−x *‖2) entspricht. Die beim Beweis gewonnenen theoretischen Einsichten können genutzt werden, um einen Gauß-Newton-ähnlichen Algorithmus zur Lösung singulärer Gleichungssysteme abzuleiten.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Griewank, A., Osborne, M. R.: Newton's method for singular problems when the dimension of the null space is >1. SIAM J. Numer Anal.18, 145–149 (1981).
Griewank, A.: On solving nonlinear equations with simple singularities nearly singular solutions. SIAM Review27, 537–563 (1985).
Kelley, C. T., Suresh, R.: A new acceleration method for Newton's method at singular points. SIAM J. Number. Anal.20, 1001–1009 (1983).
Frank, P., Schnabel, R. B.: Tensor methods for nonlinear equations. SIAM J. Numer. Anal.21, 815–843 (1984).
Schwetlick, H.: Numerische Lösung nichtlinearer Gleichungen. Berlin: VEB Deutscher Verlag der Wissenschaften 1979.
Weber, H., Werner, W.: On the accurate determination of nonisolated solutions of nonlinear equations. Computing26, 315–326 (1981).
Griewank, A., Reddien, G. W.: Characterization and computation of generalized turning points. SIAM J. Numer. Anal.21, 176–185 (1984).
Yamamoto, N.: Regularization of solutions of nonlinear equations with singular Jacobian matrices. J. Inform. Proc.7, 16–21 (1984).
Moore, G., Spence, A.: The calculation of turning points of nonlinear equations. SIAM J. Numer. Anal.17, 567–576 (1980).
Pönisch, G.: Computing simple bifurcation points using a minimally extended system of nonlinear equations. Computing35, 277–294 (1985).
Schwetlick, H.: Algorithms for finite-dimensional turning point problems for viewpoint to relationships with unconstrained optimization. In: Numerical methods for bifurcation problems (Küpper, T., Mittelmann, H. D., Weber, H., eds.), pp. 459–479 (ISNM 70). Basel: Birkhäuser 1984.
Greiwank, A., Osborne, M. R.: Analysis of Newton's method at irregular singularities. SIAM J. Numer. Anal.20, 747–773 (1983).
Kubiček, M., Holodniok, M.: Numerical determination of bifurcation points in steady state and periodic solutions — numerical algorithms and examples. In: Numerical methods for bifurcation problems (Küpper, T., Mittelmann, H. D., Weber, H., eds.), pp. 247–270 (ISNM 70). Basel: Birkhäuser 1984.
Menzel, R.: Numerical determination of multiple bifurcation points. In: Numerical methods for bifurcation problems (Küpper, T., Mittelmann, H. D., Weber, H., eds.), pp. 310–318 (ISNM 70). Basel: Birkhäuser 1984.
Menzel, R.: On solving nonlinear least-squares problems in case of rankdeficient Jacobians. Computing34, 63–72 (1985).
Rall, L. B.: Convergence of the Newton process to multiple solutions. Numer. Math.9, 23–37 (1966).
Pönisch, G., Schwetlick, H.: Computing turning points of curves implicitly defined by nonlinear equations depending on a parameter. Computing26, 107–121 (1981).
Hoy, A.: An efficiently implementable Gauss-Newton-like method for solving singular nonlinear equations. In preparation.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Hoy, A. A relation between Newton and Gauss-Newton steps for singular nonlinear equations. Computing 40, 19–27 (1988). https://doi.org/10.1007/BF02242187
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF02242187