Abstract
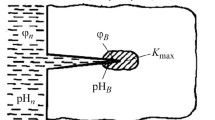
A method has been developed for predicting the growth of surface fatigue cracks with allowance for the shape of the crack front under regular cyclic loading and the presence of tensile overloads in the loading spectrum. The crack model is constructed on the basis of consideration of the specific contributions of the mechanisms that determine the advance of the crack. It is shown that allowance for such important factors as the variation in the severity of the stress state along the crack front is essential in model representations.
Similar content being viewed by others
References
J. Schijve, “Observations on the prediction of fatigue crack growth propagation under unstable-amplitude loading,” in: Fatigue-Crack Growth under Spectrum Loads. Am. Soc. Test. Mater. Spec. Tech. Publ., No. 595, 3–23.
“Current status of flight simulation fatigue crack growth under unstable amplitude loading,” Proc. 3rd Int. Spring Meeting French Met. Soc., Paris, June 15–17, 1988. London, New York (1988), pp. 267–275.
W. Elber, “The significance of fatigue crack closure,” in: Damage Tolerance in Aircraft Structures, Am. Soc. Test. Mater. Spec. Tech. Publ., No. 486, 230–242 (1971).
J. C. Newman Jr., “A crack closure model for predicting fatigue crack growth under aircraft spectrum loading,” in: Methods and Models for Predicting Fatigue Crack Growth under Random Loading. Am. Soc. Test. Mater. Spec. Tech. Publ., No. 748, 53–84 (1981).
O. N. Romaniv, G. N. Nikiforchin, and B. N. Andrusiv, “Crack closure effect and evaluation of the cyclic fracture toughness of structural alloys,” Fiz. Khim. Mekh. Mater., No. 3, 47–61 (1983).
M. Jolles and V. Tortoniello, “Effect of constraints variation on the fatigue growth of surface flaws,” Am. Soc. Test. Mater. Spec. Tech. Publ., No. 833, 300–311 (1984).
A. Clerivet and C. Bathias, “Crack closure effects in fatigue propagation of elliptical cracks,” Proc. Europ. Conf. on Fracture Control of Engng. Struct., Amsterdam, Netherlands (1989), pp 1255–1267.
A. Clerivet, “Overload influence on the crack closure mechanisms in 20 and 30 fatigue crack propagation in aluminum alloys. Fatigue crack growth under variable-amplitude loading,” Proc. 3rd Int. Spring. Meeting. French Met. Soc., Paris, June 15–17, 1988. London, New York (1988), pp. 35–47.
O. N. Romaniv, A. N. Tkach, and Yu. P. Lenets, “Possible distortion of the invariance of fatigue curves due to crack closure,” Fiz. Khim. Mekh. Mater., No. 6, 62–70 (1984).
V. T. Troshchenko, P. V. Yasnii, V. V. Pokrovskii, et al., “Effect of one-time preliminary plastic deformation on fracture toughness. Report No. 1. Rate of growth of fatigue cracks in heat-resistant steel,” Probl. Prochn., No. 12, 9–14 (1988).
V. T. Troshchenko, P. V. Yasnii, V. V. Pokrovskii, and Yu. S. Skorenko, “Method and certain results of study of the opening of a fatigue crack,” Ibid., No. 10, 8–13 (1987).
V. V. Pokrovskii and Yu. V. Tkach, “Prediction of the growth of fatigue cracks during irregular loading,” ibid., No. 8, 3–16 (1994).
J. Schijve, “Some formulas for the crack opening stress level,” Eng. Fract. Mech.,14, No. 4, 461–465 (1981).
M. Kartcher and M. Kaplan, “Effect of R-factor and crack closure on fatigue crack growth for aluminum and titanium alloys,” Am. Soc. Test. Mater. Spec. Tech. Publ., No. 559, 264–282 (1974).
S. Chen and Z. Cui, “Surface crack growth behavior under tensile cyclic loading,” Int. J. Fract,10, No. 1, 43–47 (1988).
I. S. Raju and J. C. Newman, “Stress-intensity factor for a wide range of semielliptical surface cracks in finitethickness plates,” Eng. Fract. Mech.,11, 817–829 (1979).
J. C. Newman and I. S. Raju, “An empirical stress-intensity factor equation for the surface crack,” Ibid.,15, No. 1, 2, 185–192 (1981).
A. V. Ovchinnikov, “Interpolation method of calculating stress-intensity factors,” Probl. Prochn., No. 6, 9–15 (1988).
A. A. Aboutorabi and M. J. Cowling, “Determination of stress-intensity factors for surface crack using fatigue crack growth data,” Int. J. Fract., 31, 67–79 (1986).
K. T. Venkatesvara and R. O. Ritchie, “Micromechanisms of transient fatigue crack growth behavior in aluminumlithium alloys following single tensile overloads,” Fatigue Crack Growth under Variable Amplitude Loading. Proc. 3rd Int. Spring Meeting. French Met. Soc., Paris, June 15–17 (1988). London, New York (1988), pp. 134–135.
C. M. Ward-Close, A. F. Blom, and R. O. Ritchie, “Mechanisms associated with transient fatigue crack growth under variable-amplitude loading: an experimental and numerical study,” Eng. Fract. Mech.,32, No. 4, 613–638.
S. Suresh, “Micromechanisms of fatigue crack growth retardation following overloads,” Ibid.,18, No. 7, 577–593.
D. Brock, Principles of Fracture Mechanics [Russian translation], Vyssh. Shk. (1980).
Author information
Authors and Affiliations
Additional information
Translated from Problemy Prochnosti, No. 1, pp. 36–47, January, 1996.
Rights and permissions
About this article
Cite this article
Pokrovskii, V.V., Tkach, Y.V. & Ivanchenko, A.G. Method of evaluating the residual life of structural elements with crack-like surface defects. Report no. 1. Modeling the growth of a surface fatigue crack. Strength Mater 28, 24–32 (1996). https://doi.org/10.1007/BF02209095
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02209095