Abstract
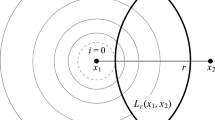
We analyze the behaviour of thek center and median problems forn points randomly distributed in an arbitrary regionA ofR d. Under a mild assumption on the regionA, we show that fork≦k(n)=o(n/logn), the objective function values of the discrete and continuous versions of these problems are equal to each otheralmost surely. For the two-dimensional case, both these problems can be solved by placing the centers or medians in an especially simple regular hexagonal pattern (the ‘honeycomb heuristic’ of Papadimitriou). This yields the exact asymptotic values for thek center and median problem, namely, α(|A|/k)1/2 and β(|A|/k)1/2, where |A| denotes the volume ofA, α and β are known constants, and the objective of the median problem is given in terms of the average, rather than the usual total, distance. For the 3- and 4-dimensional case, similar results can be obtained for the center problem to within an accuracy of roughly one percent. As a by-product, we also get asymptotically optimal algorithms for the 2-dimensionalp-normk median problem and for the twin problems of minimizing the maximum number of vertices served by any center and similarly for maximizing the minimum.
Similar content being viewed by others
References
R.B. Bambah, On lattice coverings by spheres, Proc. Nat. Inst. Sci. India 20(1954)25.
E.S. Barnes, The coverings of space by spheres, Can. J. Math. 8(1957)293.
J. Beardwood, J.H. Halton and J.M. Hammersely, The shortest path through many points, Proc. Camb. Phil. Soc. 55(1959)299.
H.S.M. Coxeter, L. Few and C.A. Rogers, Covering space with equal spheres, Mathematica 6, (1959)247.
L. Fejes Toth, Lagerungen in der Ebene, auf der Kugel und im Raum (Berlin, 1953).
L. Fejes Toth, Sum of moments of convex polygons, Acta Math. Acad. SciHHungaricae 24 (3–4)(1973)417.
L. Few, Covering space by spheres, Mathematica 7(1960)136.
M.L. Fischer and D.S. Hochbaum, Probabilistic analysis of the euclideank-median problem, Math. Oper. Res. (1983)
P.J. Heawood, Map colour theorem, Quart. J. Math. 24(1980)332.
D.S. Hochbaum, The probabilistic asymptotic properties of some combinatorial geometric problems (Carnegie-Mellon University, Nov. 1979) manuscript.
R.M. Karp, The probabilistic analysis of some combinatorial search algorithms, in: Algorithms and Complexity: New Directions and Recent Results, ed. J.F. Traub (Academic Press, New York, 1977).
R.M. Karp, Probabilistic analysis of partioning algorithms for the travelling salesman problem, Math. Oper. Res. 2(1977)209.
R. Kershner, The number of circles covering a set, Amer. J. Math. 61(1939)665.
S. Masuyama, T. Ibaraki and T. Hasegawa, The computational complexity of them-center problem on the plane, Trans. IECE of Japan 64, No. 2 (1981)57.
N. Megiddo and K.J. Supowit, On the complexity of some common geometric location problems (1981) manuscript.
C.H. Papadimitriou, Worst case and probabilistic analysis of a geometric location problem, SIAM J. Comp. 10, No. 3 (1981)542.
A. Renyi, Foundations of Probability (Holden Day, Inc., San Francisco, 1970).
C.A. Roger, Packing and Covering (Cambridge Tracts in Mathematics and Mathematical Physics, 1964).
J. Michael Steele, Subaddative euclidean functionals and non-linear growth in geometric probability, Ann. Prob.
E. Zemel, Probabilistic analysis of geometric location problems, SIAM J. on Discrete and Algebraic Methods, to appear.
Author information
Authors and Affiliations
Additional information
Supported, in part, by NSF Grant ECS-8121741, and by the Israel Institute of Business Research, Tel Aviv University. Part of this work was done when the author was visiting Tel Aviv University.
A revised version of this manuscript will appear as [20].
Rights and permissions
About this article
Cite this article
Zemel, E. Probabilistic analysis of geometric location problems. Ann Oper Res 1, 215–238 (1984). https://doi.org/10.1007/BF01874390
Issue Date:
DOI: https://doi.org/10.1007/BF01874390