Skip to main content
SpringerLink
Log in

Geometry of optimality conditions and constraint qualifications: The convex case

  • Published:
Mathematical Programming Submit manuscript

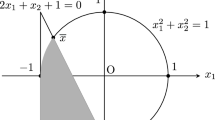
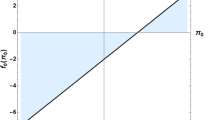
Abstract

The cones of directions of constancy are used to derive: new as well as known optimality conditions; weakest constraint qualifications; and regularization techniques, for the convex programming problem. In addition, the “badly behaved set” of constraints, i.e. the set of constraints which causes problems in the Kuhn—Tucker theory, is isolated and a computational procedure for checking whether a feasible point is regular or not is presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J. Abadie, “On the Kuhn—Tucker theorem”, in: J. Abadie, ed.,Nonlinear Programming (North-Holland Publishing Co., Amsterdam, 1967).

    Google Scholar 

  2. R.A. Abrams and L. Kerzner, “A simplified test for optimality”,Journal of Optimization Theory and Applications 25 (1978) 161–170.

    Google Scholar 

  3. K. Arrow, L. Hurwicz and H. Uzawa, “Constraint qualifications in maximization problems”,Naval Research Logistics Quarterly 8 (1961) 175–191.

    Google Scholar 

  4. C.C. Augunwamba, “Optimality conditions: constraint regularization”,Mathematical Programming 13 (1977) 38–48.

    Google Scholar 

  5. M.S. Bazaraa, J.J. Goode and C.M. Shetty, “Constraint qualifications revisited”,Management Science 18 (1972) 567–573.

    Google Scholar 

  6. M.S. Bazaraa, C.M. Shetty, J.J. Goode and M.Z. Nashed, “Nonlinear programming without differentiability in Banach spaces: necessary and sufficient constraint qualification”,Applicable Analysis 5 (1976) 165–173.

    Google Scholar 

  7. A. Ben-Israel, A. Ben-Tal and S. Zlobec, “Characterization of optimality in convex programming without a constraint qualification”,Journal of Optimization Theory and Applications 20 (1976) 417–437.

    Google Scholar 

  8. A. Ben-Israel, A. Ben-Tal and S. Zlobec, “Optimality conditions in convex programming”, The IX International Symposium on Mathematical Programming (Budapest, Hungary, August 1976).

    Google Scholar 

  9. A. Ben-Tal and A. Ben-Israel, “Characterizations of optimality in convex programming: the nondifferentiable case”,Applicable Analysis, to appear.

  10. A. Ben-Tal, L. Kerzner and S. Zlobec, “Optimality conditions for convex semi-infinite programming problems”, Report (1976).

  11. A. Ben-Tal and S. Zlobec, “A new class of feasible direction methods”, Report (1977).

  12. J. Borwein, “Proper efficient points for maximizations with respect to cones”,SIAM Journal of Control and Optimization 15 (1977) 57–63.

    Google Scholar 

  13. J. Borwein and H. Wolkowicz, “Characterizations of optimality without constraint qualification for the abstract convex program. Part I”, Report No. 14, Dalhousie University (Halifax, June 1979).

    Google Scholar 

  14. J. Borwein and H. Wolkowicz, “Characterizations of optimality without constraint qualification for the abstract convex program. Part II: finite dimensional range space”, Report No. 19, Dalhousie University (Halifax, July 1979).

    Google Scholar 

  15. F.H. Clarke, “Nondifferentiable functions in optimization”,Applied Mathematics Notes 2 (Dec. 1976).

  16. V.F. Dem'yanov and V.N. Malozemov,Introduction to minimax, Translated from Russian by D. Louvish (John Wiley & Sons, New York, 1974).

    Google Scholar 

  17. J. Gauvin, “A necessary and sufficient regularity condition to have bounded multipliers in nonconvex programming”,Mathematical Programming 12 (1977) 136–138.

    Google Scholar 

  18. A.M. Geoffrion, “Duality in nonlinear programming: a simplified applications-oriented development”,SIAM Review 13 (1971) 1–37.

    Google Scholar 

  19. I.V. Girsanov,Lectures on mathematical theory of extremum problems, Lecture Notes in Economics and Mathematical Systems, No. 67 (Springer-Verlag, Berlin, 1972).

    Google Scholar 

  20. F.J. Gould and J.W. Tolle, “Geometry of optimality conditions and constraint qualifications”,Mathematical Programming 2 (1972) 1–18.

    Google Scholar 

  21. F.J. Gould and J.W. Tolle, “A necessary and sufficient qualification for constrained optimization”,SIAM Journal of Applied Mathematics 20 (1971) 164–171.

    Google Scholar 

  22. M. Guignard, “Generalized Kuhn—Tucker conditions for mathematical programming problems in a Banach space”,SIAM Journal of Control 7 (1969) 232–241.

    Google Scholar 

  23. R.B. Holmes,A course on optimization and best approximation, Lecture Notes in Mathematics, No. 257 (Springer-Verlag, Berlin, 1972).

    Google Scholar 

  24. B.N. Pshenichnyi,Necessary conditions for an extremum (Marcel Dekker, New York, 1971).

    Google Scholar 

  25. S.M. Robinson, “Stability theory for systems of inequalities. Part I: linear systems”,SIAM Journal of Numerical Analysis 12 (1975) 754–769.

    Google Scholar 

  26. S.M. Robinson, “Stability theory for systems of inequalities. Part II: differentiable nonlinear systems”,SIAM Journal of Numerical Analysis 13 (1976) 497–513.

    Google Scholar 

  27. R.T. Rockafellar,Convex analysis (Princeton University Press, Princeton, NJ, 1970).

    Google Scholar 

  28. R.T. Rockafellar, “Some convex programs whose duals are linearly constrained”, in: J.B. Rosen, O.L. Mangasarian and K. Ritter, eds.,Nonlinear programming (Academic Press, New York, 1970) 293–322.

    Google Scholar 

  29. H. Tuy, “Stability property of a system of inequalities”,Mathematische Operationsforschung und Statistik, Ser. Optimization 8 (1977) 27–39.

    Google Scholar 

  30. H. Wolkowicz, “Calculating the cone of directions of constancy”,Journal of Optimization Theory and Applications 25 (1978) 451–457.

    Google Scholar 

  31. H. Wolkowicz, “Constructive approaches to approximate solutions of operator equations and convex programming”, Ph.D. Thesis, McGill University (Montreal, Que., 1978).

    Google Scholar 

  32. H. Wolkowicz, “Shadow prices for an unstable convex program”, Report No. 15, Dalhousie University (Halifax, May 1979).

    Google Scholar 

  33. S. Zlobec,Mathematical Programming, Lecture notes (McGill University, Montreal, Que., 1977).

    Google Scholar 

  34. S. Zlobec, “Feasible direction methods in the absence of Slater's condition”,Mathematische Operationsforschung und Statistik, Ser. Optimization 9 (1978) 185–196.

    Google Scholar 

  35. G. Zoutendijk,Methods of feasible directions: a study in linear and non-linear programming (Elsevier, New York, 1960).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, The University of Alberta, Edmonton, Alta., Canada

    Henry Wolkowicz

Authors
  1. Henry Wolkowicz
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

This research was supported by the National Research Council of Canada and le Gouvernement du Quebec and is part of the author's Ph.D. Dissertation done at McGill University, Montreal, Que., under the guidance of Professor S. Zlobec.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wolkowicz, H. Geometry of optimality conditions and constraint qualifications: The convex case. Mathematical Programming 19, 32–60 (1980). https://doi.org/10.1007/BF01581627

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01581627

Key words

Search

Navigation