Abstract
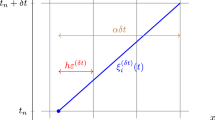
A method is described for efficient treatment of the time-history effects that arise in closure models constructed by truncation of renormalized perturbation expansions. The scheme is based on rational polynomial (Padé) approximations for the time-lagged functions, combined with Gaussian quadrature applied to a coarse-time evolution equation for equal-time covariances. Power series coefficients required for the Padé approximants are generated by recursion relations resulting from successive differentiation of the slow-varying form of the dynamic equations for time-lagged functions. This strategy is illustrated by applications to equations of the direct interaction approximation for fluid turbulence. Several benefits accrue from such an approach. Computational efficiency comparable to that of Markovian closures is obtained without introduction of arbitrary constants orad hoc Markovianization assumptions: (1) Scaling of the leading operations count is reduced fromO(n 3 T ) toO(n g n t ), wheren T is the number of steps in coarse time, and ng≈ 4–8. For typical problems in fluid turbulence, this results in savings of afactor∼40 in computing time. (2) Scaling of working array size is reduced fromO(n 2 T ) toO(p), wherep is the order of Padé approximant; this reduces in-core storage requirements for dynamical quantities by afactor ∼20. These and other advantages depend on the order of Padé approximant required to give acceptable accuracy. Test cases from several problems in fluid turbulence indicate very substantial savings, such that certain CPU-intensive problems with spatial inhomogeneities become computationally feasible.
Similar content being viewed by others
References
Baker, G. (1965). The theory and application of the Padé approximant method,Adv. Theor. Phys. 1, 1.
Baker, G. (1975).Essentials of Padé Approximants, Academic Press, New York.
Baker, G., and Graves-Morris, P. (1981). Padé approximants: Basic theory, inEncyclopedia of Mathematics, Vol. 13, Addison-Wesley, Reading, Massachusetts.
Bender, C. M., and Orszag, S. A. (1978).Advanced Mathematical Methods for Scientists and Engineers, McGraw-Hill, New York.
Carnevale, G. F., and Frederiksen, J. S. (1983). A statistical dynamical theory of strongly nonlinear internal gravity waves,Geophys. Astrophys. Fluid Dynamics 23, 175.
Carnevale, G. F., and Martin, P. C. (1982). Field theoretical techniques in statistical fluid dynamics: with application to nonlinear wave dynamics,Geophys. Astrophys. Fluid Dynamics 20, 131.
Dannevik, W. (1985). Two-Point Closure Study of Covariance Budgets for Turbulent Rayleigh-Benard Convection, National Center for Atmospheric Research, NCAR/Ct-85.
Dannevik, W. (1986). Two-point closure model for weakly-turbulent thermal convection, In preparation.
Deker, U., and Haake, F. (1975). Fluctuation-dissipation theorems for classical processes,Phys. Rev. A 11, 6, 2043.
De Leener, M., and Resibois, P. (1969). Irreversibility in Heisenberg spin systems. IV. Time-dependent fluctuations at the Curie point,Phys. Rev. 178, 819.
Domaradzki, A., and Orszag, S. A. (1986). Direct interaction approximation for anisotropic turbulence,Phys. Fluids, submitted.
Dubois, D. F., and Rose, H. A. (1981). Statistical theories of Langmuir turbulence. I. Direct-interaction-approximation responses,Phys. Rev. A 24, 1476.
Enz, C. P., and Garrido, L. (1976). Perturbation theory for classical thermodynamic Green's functions,Phys. Rev. A 14, 1258.
Frisch, U., and Bourrett, R. (1970). Parastochastic,J. Math. Phys. 11, 364.
Frisch, U., Lesieur, M., and Brissaud, A. (1974). A Markovian random coupling model for turbulence,J. Fluid Mech. 65, 145.
Fujisaka, H., and Yamada, T. (1977). Theoretical study of a chemical turbulence,Prog. Theor. Phys. 57, 734.
Graves-Morris, P. (1973).Padé Approximates, The Institute of Physics, London.
Herring, J. R. (1963). Investigation of problems in thermal convection,J. Atmos. Sci. 20, 325.
Herring, J. R. (1965). Self-consistent-field approach to turbulence theory,Phys. Fluids 8, 2219.
Herring, J. R. (1966). Self-consistent field approach to non-stationary turbulence,Phys. Fluids 9, 2106.
Herring, J. R. (1969). Statistical theory of thermal convection at large Prandtl number,Phys. Fluids 12, 39.
Herring, J. R. (1985). An introduction and overview of various theoretical approaches to turbulence, in Dwoyer, D. L., Hussaini, M. Y., and Voight, R. G. (eds.),Theoretical Approaches to Turbulence, Springer, New York.
Herring, J. R., and Kerr, R. (1982). Comparisons of direct numerical simulations with predictions of two-point closures for isotropic turbulence convecting a passive scalar,J. Fluid Mech. 118, 205.
Herring, J. R., and Kraichnan, R. H. (1972). Comparison of some approximations for isotropic turbulence, inStatistical Models and Turbulence, Springer, New York.
Kadanoff, L. P., and Baym, G. (1962).Quantum Statistical Mechanics, W. A. Benjamin, New York.
Kraichnan, R. H. (1958). Irreversible statistical mechanics of incompressible hydromagnetic turbulence,Phys. Rev. 109, 1407.
Kraichnan, R. H. (1963). Direct-interaction approximation for a system of several interacting simple shear waves,Phys. Fluids 6, 1603.
Kraichnan, R. H. (1964a). Decay of isotropic turbulence in the direct-interaction approximation,Phys. Fluids 7, 1030.
Kraichnan, R. H. (1964b). Approximations for steady-state isotropic turbulence,Phys. Fluids 7, 1163.
Kraichnan, R. H. (1965). Lagrangian-history closure approximation for turbulence,Phys. Fluids 8, 575.
Kraichnan, R. H. (1968). Convergents to infinite series in turbulence theory,Phys. Rev. 174, 240.
Kraichnan, R. H. (1971). An almost-Markovian Galilean-invariant turbulence model,J. Fluid Mech. 47, 513.
Krommes, J. A. (1982). The direct-interaction approximation and statistically steady states of three nonlinearly coupled modes,Phys. Fluids 25, 1393.
Martin, P. C., Siggia, E. D., and Rose, H. A. (1973). Statistical dynamics of classical systems,Phys. Rev. A 8, 423.
Meneguzzi, M., Sulem, C., Sulem, P. L., and Thual, O. (1985). Time-dependent Rayleigh-Benard convection in low Prandtl number fluids, in Frisch, U.,el al. (eds.),Macroscopic Modelling of Turbulent Flows, Springer, New York.
Orszag, S. A. (1970). Analytical theories of turbulence,J. Fluid Mech. 41, 363.
Orszag, S. A., and Patterson, G. (1972). Numerical simulation of turbulence, inStatistical Models and Turbulence, Springer. New York.
Phythian, R. (1975). The operator formalism of classical statistical dynamics,J. Phys. 8, 1423.
Phythian, R. (1976). Further application of the Martin, Siggia, Rose formalism,J. Phys. 9, 269.
Rose, H. A. (1985). An efficient non-Markovian theory of non-equilibrium dynamics,Physica 14D, 216.
Takayasu, H. (1985). Statistical properties of a 3-wave system.J. Phys. Soc. Japan 54, 1317.
Yoshizawa, A. (1980). Statistical theory for Boussinesq turbulence,J. Phys. Soc. Japan 48, 647.
Yoshizawa, A. (1982). A statistically-derived subgrid model for the large eddy simulation of turbulence,Phys. Fluids 25, 1532.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Dannevik, W.P. Efficient solution of non-Markovian covariance evolution equations in fluid turbulence. J Sci Comput 1, 151–182 (1986). https://doi.org/10.1007/BF01061391
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF01061391