Abstract
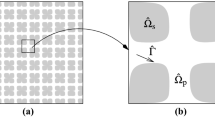
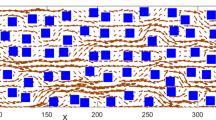
We develop a multi-length-scale (multifractal) theory for the effect of rock heterogeneity on the growth of the mixing layer of the flow of a passive tracer through porous media. The multifractal exponent of the size of the mixing layer is determined analytically from the statistical properties of a random velocity (permeability) field. The anomalous diffusion of the mixing layer can occur both on finite and on asymptotic length scales.
Similar content being viewed by others
References
Y. Amirat, K. Hamdache, and A. Ziani, Homogénéisation d'équations hyperboliques du premier ordre et application aux écoulements miscibles en milieu poreux,Ann. Inst. Henri Poincaré 6:397 (1989).
J. A. Aronovitz and D. R. Nelson, Anomalous diffusion in steady fluid flow through a porous medium,Phys. Rev. A 30:1048 (1984).
A. Arya, T. A. Hewett, R. G. Larson, and L. W. Lake, Dispersion and reservoir heterogeneity, SPE 15386, 60th Annual Technical Conference of the Society of Petroleum Engineers, Las Vegas, Nevada (1985).
A. A. Bakr, L. W. Gelhar, A. L. Gutjahr, and J. R. MacMillan, Stochastic analysis of spatial variable in subsurface flows, i 1. Comparison of one- and three-dimension flows,Water Resources Res. 14:263 (1978).
R. E. Collins,Flow of Fluids Through Porous Materials (Van Nostrand-Reinhold, Princeton, New Jersey, 1961).
G. Dagan, Solute transport in heterogenous porous formations,J. Fluid Mech. 145:151 (1984).
A. S. Emanuel, G. K. Alameda, R. A. Behrens, and T. A. Hewett, Reservoir performance prediction methods based on fractal geostatistics,SPE Reservoir Eng. 4:313 (1988).
D. S. Fisher, Random walks in random environments,Phys. Rev. A 30:960 (1984).
R. A. Freeze, Stochastic-conceptual analysis of one-dimensional groundwater flow in nonuniform homogeneous media,Water Resources Res. 11:725 (1975).
F. Furtado, J. Glimm, B. Lindquist, and F. Pereira, Multi-length scale components of the mixing length growth in tracer flow, inProceedings of the Emerging Technologies Conference, F. Kovarik, ed. (Houston, Texas, 1990), p. 251.
F. Furtado, J. Glimm, B. Lindquist, and F. Pereira, Characterization of mixing length growth for flow in heterogeneous porous media, SPE 21233, inProceedings of the 11th SPE Symposium on Reservoir Simulation (1991).
F. Furtado, J. Glimm, B. Lindquist, F. Pereira, and Q. Zhang, Time dependent anomalous diffusion for flow in multi-fractal porous media, inProceedings of the Workshop on Numerical Methods for the Simulation of Multiphase and Complex Flow, T. M. Verheggan, ed. (Springer-Verlag, New York, 1991).
L. W. Gelhar, Stochastic subsurface hydrology from theory to applications,Water Resources Res. 22:153S (1986).
L. W. Gelhar and C. L. Axness,Water Resources Res. 19:161 (1983).
J. Glimm and D. H. Sharp, A random field model for anomalous diffusion in heterogeneous porous media,J. Stat. Phys. 62:415 (1991).
N. Goldenfield, O. Martin, Y. Oono, and F. Liu, Anomalous dimensions and the renormalization group in a nonlinear diffusion process,Phys. Rev. Lett. 64:1361 (1990).
A. L. Gutjahr and L. W. Gelhar, Stochastic models of subsurface flow: Infinite versus finite domains and stationarity,Water Resources Res. 17:337 (1981).
T. A. Hewett and R. A. Behrens, Conditional simulation of reservoir heterogeneity with fractals, SPE 18326, SPE Annual Technical Conference and Exhibition, Houston, Texas (1988).
H. Kesten and G. Papanicolaou, A limit theorem for turbulent diffusion,Comm. Math. Phys. 65:97 (1979).
P. R. King, The use of field theoretic method for the study of flow in a heterogeneous porous medium,J. Phys. A 20:3935 (1987).
J. Koplik, Creeping flow in two-dimensional networks,J. Fluid Mech. 119:219 (1982).
R. H. Kraichman, Eulerian and Lagrangian renormalization in turbulence theory,J. Fluid Mech. 83:349 (1977).
L. Lake and H. Carroll, eds.,Reservoir Characterization (Academic Press, 1986).
A. Lallemand-Barres and P. Peaudecerf, Recherche des relations entre le valeur de la dispersivité macroscopic, ses autres caractéristiques et les conditions de mesure, Bulletin BRGM, 2e Série, Section III, No. 4 (1978).
W. D. McComb,The Physics of Turbulence (Oxford University Press, Oxford, 1990).
S. P. Neuman, Universal scaling of hydraulic conductivities and dispersivities in geologic media,Water Resources Res. 26:1749 (1990).
S. P. Neuman and Y. K. Zhang, A quasi-linear theory of non-Fickian subsurface dispersion,Water Resources Res. 26:887 (1990).
P. J. Smith and C. E. Brown, Stochastic modeling of two-phase flow in porous media, SPE 11128, 57th Annual Fall Technical Conference and Exhibition of the Society of Petroleum Engineers, New Orleans, Louisiana (1982).
L. Tartar, Nonlocal effects induced by homogeneization, inPartial Differential Equations and the Calculus of Variations, Vol. 2, Essays in Honor of Ennio De Giorgi (Birkhauser, 1989), pp. 925–938.
C. L. Winter, C. M. Neuman, and S. P. Newman, A perturbation expansion for diffusion in a random velocity field,SIAM J. Appl. Math. 44:411 (1984).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Zhang, Q. A multi-length-scale theory of the anomalous mixing-length growth for tracer flow in heterogeneous porous media. J Stat Phys 66, 485–501 (1992). https://doi.org/10.1007/BF01060076
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01060076