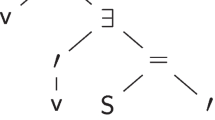A note on the ω-incompleteness formalization

Abstract

The paper studies two formal schemes related to ω-completeness.

LetS be a suitable formal theory containing primitive recursive arithmetic and letT be a formal extension ofS. Denoted by (a), (b) and (c), respectively, are the following three propositions (where α(x) is a formula with the only free variable x): (a) (for anyn) (⊢ _T α(n)), (b) ⊢ _T ∀ _x Pr _T (⁻α(x)⁻) and (c) ⊢ _T ∀xα(x) (the notational conventions are those of Smoryński [3]). The aim of this paper is to examine the meaning of the schemes which result from the formalizations, over the base theoryS, of the implications (b) ⇒ (c) and (a) ⇒ (b), where α ranges over all formulae. The analysis yields two results overS : 1. the schema corresponding to (b) ⇒ (c) is equivalent to ¬Cons _T and 2. the schema corresponding to (a) ⇒ (b) is not consistent with 1-CON _T. The former result follows from a simple adaptation of the ω-incompleteness proof; the second is new and is based on a particular application of the diagonalization lemma.