Skip to main content
SpringerLink
Log in

Immiscible displacement in vertically fractured reservoirs

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

Abstract

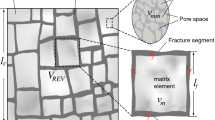
A dual-porosity model is defined for saturated, two-phase, compressible, immiscible flow in a vertically fractured reservoir or aquifer. This model allows detailed simulation of the matrix-fracture interaction as well as the matrix flow itself. This is accomplished by directly coupling the matrix and fracture systems along the vertical faces of the matrix blocks, incorporating gravitational effects directly, and simulating flow inside the block. Thus fluid segregation due to gravitational effects and heterogeneities can be simulated. We show that our model can be derived via homogenization techniques. The model (in incompressible form for simplicity of exposition) is then approximated by a computationally efficient finite difference scheme. Calculations are presented to show the convergence of the scheme and to indicate the behavior of the model as a function of several parameters.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

B :

a block

D :

areal extent (horizontal cross-section) of the reservoir domain

e :

Cartesian unit vector

E :

scaling tensor for the homogenization

g,g :

gravitational constant

h,H :

grid spacing

þ :

reservoir thickness

I=(0,þ):

vertical extent of the reservoir domain

k,K,K :

absolute permeability

k r :

relative permeability

L,l :

grid nodal numbers

n,N :

number of grid cells

N :

grid nodal positions

p,P :

pressure

p c,P c :

capillary pressure function

P −1 c :

inverse of capillary pressure function

q :

source of sink

Q :

cross-section of a block

q m :

matrix-to-fracture source (i.e., the transfer function)

s,S :

saturation (with no phase subscript,s=s w)

s r :

residual saturation

t :

time

w :

auxiliary function used to solve the closure problem

x,y :

position

xit',yit' :

horizontal position

y:

gravity-density term

γ:

boundary of a matrix block

δ:

1 ifi=j, 0 otherwise

∂B, ∂Q, ∂Ω:

boundary of the given domain

∇t :

time step

ε :

homogenization parameter

References

  1. Arbogast, T., A simplified dual-porosity model for two-phase flow, in T. F. Russell, R. E. Ewing, C. A. Brebbia, W. G. Gray, and G. F. Pindar (eds),Computational Methods in Water Resources IX, Vol. 2: Mathematical Modeling in Water Resources, Computational Mechanics Publications, Southampton, U.K., 1992, pp. 419–426.

    Google Scholar 

  2. Arbogast, T.Gravitational forces in dual-porosity systems. I. Model derivation by homogenization, (to appear).

  3. Arbogast, T.Gravitational forces in dual-porosity systems. II. Computational validation of the homogenized model, (to appear).

  4. Arbogast, T., Douglas, Jr. J., and Hornung, U., Modeling of naturally fractured reservoirs by formal homogenization techniques, in R. Dautray (ed.),Frontiers in Pure and Applied Mathematics, Elsevier, Amsterdam, 1991, pp. 1–19.

    Google Scholar 

  5. Arbogast, T., Douglas, Jr., J. and Hornung, U., Derivation of the double porosity model of single phase flow via homogenization theory,SIAM J. Math. Anal.,21 (1990), 823–836.

    Google Scholar 

  6. Arbogast, T., Douglas, Jr., J. and Santos, J. E., Two-phase immiscible flow in naturally fractured reservoirs, in Mary F. Wheeler (ed.),Numerical Simulation in Oil Recovery, The IMA Volumes in Mathematics and its Applications, Springer-Verlag, Berlin, 1988, pp. 47–66.

    Google Scholar 

  7. Aziz, K. and Settari, T.,Petroleum Reservoir Simulation, Applied Science Publishers, London, 1979.

    Google Scholar 

  8. Bear, J.,Dynamics of Fluids in Porous Media, Dover Publications, New York, 1988.

    Google Scholar 

  9. Bensoussan, A., Lions, J. L. and Papanicolaou, G.,Asymptotic Analysis for Periodic Structures, North-Holland, Amsterdam, 1978.

    Google Scholar 

  10. Chen, Z. and Liu, C., Theory of fluid displacement in a medium with double porosity,Energy Sources 6 (1982), 193–214.

    Google Scholar 

  11. Douglas, Jr. J. and Arbogast, T., Dual porosity models for flow in naturally fractured reservoirs, in John H. Cushman (ed.),Dynamics of Fluids in Hierarchical Porous Media, Academic Press, London, 1990, pp. 177–221.

    Google Scholar 

  12. Douglas, Jr., J., Arbogast, T. and Paes Lerne, P. J., Two models for the waterflooding of naturally fractured reservoirs, inProceedings, Tenth SPE Symposium on Reservoir Simulation, Paper SPE 18425, Society of Petroleum Engineers, Dallas, Texas, U.S.A., 1989, pp. 219–225.

    Google Scholar 

  13. Douglas, Jr., J., Hensley, J. L. and Arbogast, T., A dual-porosity model for waterflooding in naturally fractured reservoirs;Comp. Math. in Appl. Mech. and Engng. 87 (1991), 157–174.

    Google Scholar 

  14. Douglas, Jr., J., Hensley, J. L., Arbogast, T., Paes-Leme, P. J. and Nunes, N. P., Medium and tall block models for immiscible displacement in naturally fractured reservoirs, in preparation.

  15. Douglas, Jr., J., Paes Lerne, P. J. and Hensley, J. L., A limit form of the equations for immiscible displacement in a fractured reservoir,Transport in Porous Media 6 (1991), 549–565.

    Google Scholar 

  16. Douglas, Jr., J., Peaceman, D. W. and Rachford, Jr., H. H., A method for calculating mutidimensional displacement,Trans. AIME 216 (1959), 297–308.

    Google Scholar 

  17. Ene, H. I. and Vernescu, B., Homogenization of a singular perturbation problem,Rev. Roumaine Math. Pures Appl. 30 (1985), 815–822.

    Google Scholar 

  18. Ene, H. I., Application of the homogenization method to transport in porous media, in John H. Cushman (ed.),Dynamics of Fluids in Hierarchical Porous Media, Academic Press, London, 1990, pp. 223–241.

    Google Scholar 

  19. Firoozabadi, A. and Thomas, L. K., Sixth SPE comparative solution project: Dual-porosity simulators,J. Petrol. Technol. 42 (1990), 710–715, 762–763.

    Google Scholar 

  20. Gilman, J. R., An efficient finite-difference method for simulating phase segregation in the matrix blocks in double-porosity reservoirs,Soc. Petrol. Engr. J. 26 (1986), 403–413.

    Google Scholar 

  21. Gilman, J. R. and Kazemi, H., Improvements in simulation of naturally fractured reservoirs,Soc. Petrol. Engr. J. 23 (1983), 695–707.

    Google Scholar 

  22. Gilman, J. R. and Kazemi, H., Improved calculations for viscous and gravity displacement in matrix blocks in dual-porosity simulators,J. Petrol. Technol. 40 (1988), 60–70.

    Google Scholar 

  23. Huyakorn, P. S., Lester, B. H. and Faust, C. R., Finite element techniques for modeling groundwater flow in fractured aquifers,Water Resour. Res. 19 (1983), 1019–1035.

    Google Scholar 

  24. Levy, T., Filtration in a porous fissured rock: influence of the fissures connexity,Eur. J. Mech., B/Fluids 9 (1990), 309–327.

    Google Scholar 

  25. Paes Leme, P. J., Douglas, Jr., J., Arbogast, T. and Nunes, N. P., A tall block model for immiscible displacement in naturally fractured reservoirs, inProc. SPE Meeting, Rio de Janeiro, Paper SPE 21104, October 15–19, 1990.

  26. Peaceman, Donald W.,Fundamentals of Numerical Reservoir Simulation, Elsevier, New York, 1977.

    Google Scholar 

  27. Preuss, K. and Narasimhan, T. N., A practical method for modeling fluid and heat flow in fractured porous media;Soc. Petroleum Engr. J. 25 (1985), 14–26.

    Google Scholar 

  28. Sanchez-Palencia, E.,Non-homogeneous Media and Vibration Theory, Lecture Notes in Physics 127, Springer-Verlag, Berlin, 1980.

    Google Scholar 

  29. Sonier, F., Souillard, P. and Blaskovich, F. T., Numerical simulation of naturally fractured reservoirs,SPE Reservoir Eng. 3 (1988), 1114–1122.

    Google Scholar 

  30. de Swaan, A., Theory of waterflooding in fractured reservoirs,Soc. Petrol. Engr. J. 18 (1978), 117–122.

    Google Scholar 

  31. Thomas, L. K., Dixon, T. N. and Pierson, R. G., Fractured reservoir simulation,Soc. Petrol. Engr. J. 23 (1983), 42–54.

    Google Scholar 

  32. Witherspoon, P. A.et al., Validity of cubic law for fluid flow in a deformable rock fracture,Water Resour. Res. 16 (1980), 1016–1024.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Purdue University, 47907, West Lafayette, IN, USA

    Jim Douglas Jr.

  2. Department of Computational and Applied Mathematics, Rice University, 77251-1892, Houston, TX, USA

    Todd Arbogast

  3. Departamento de Matemática, Pontificia Universidade Católica do RJ, 22453, Rio de Janeiro, RJ, Brazil

    Paulo J. Paes-Leme

  4. Center for Parallel and Scientific Computing, The University of Tulsa, 74104, Tulsa, OK, USA

    Jeffrey L. Hensley

  5. Instituto Politécnico do Rio de Janeiro, 28600, Nova Friburgo, RJ, Brazil

    Neci P. Nunes

Authors
  1. Jim Douglas Jr.
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Todd Arbogast
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Paulo J. Paes-Leme
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jeffrey L. Hensley
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Neci P. Nunes
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Douglas, J., Arbogast, T., Paes-Leme, P.J. et al. Immiscible displacement in vertically fractured reservoirs. Transp Porous Med 12, 73–106 (1993). https://doi.org/10.1007/BF00616363

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF00616363

Key-words

Search

Navigation