Abstract
The thickness of the thin liquid film and its effects have always been a research hotspot in nuclear power applications and operating nuclear power plants, because the flow phenomenon of the liquid film is extremely common in multiphase flow research. Based on papers published in recent years, novel research progress on thin film thickness is reviewed from the following two perspectives: the experimental measurement and the theoretical model of liquid film thickness. For the experimental measurement, methods mainly include the PLIF method, the capacitance method, and the ultrasonic method. The following contents in this part are mainly reviewed from the PLIF method, the capacitance method, the ultrasonic method, and other methods for the measurement of the liquid film thickness on the wall, the liquid film thickness on the tube wall, and other aspects of the liquid film thickness. For the model, the theoretical model of liquid film thickness on the wall is mainly reviewed from two aspects: vertical flow and horizontal flow. Other models are mainly reviewed from the following aspects. They are annular film thickness, liquid film thickness for gas–liquid annular flow, and film thickness in pipes.
Similar content being viewed by others
Change history
05 February 2022
A Correction to this paper has been published: https://doi.org/10.1007/s42757-022-0132-z
Abbreviations
- Ar :
-
Archimedes number
- Bo :
-
Bond number based on the bubble acceleration
- C :
-
Capacitance between two parallel conductive plates
- Ca :
-
Capillary number
- Ca**:
-
Criterion for the limit in capillary number
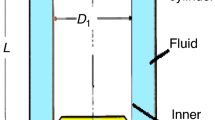
- D :
-
Outside diameter of tube
- D i :
-
Tube inner diameter
- Fr G :
-
Froude number for gas phase
- h :
-
Liquid film thickness
- I t :
-
Sum of the reflected light intensity
- I 0 :
-
Incident intensity
- k :
-
Spectral absorption coefficient
- n 1 :
-
Refractive indexes of air absorption medium
- n 2 :
-
Refractive indexes of liquid absorption medium
- R :
-
Reflectivity on the liquid-gas interface
- Re :
-
Liquid film Reynolds number
- Q :
-
Liquid feeding rate
- S :
-
Intertube spacing
- v*:
-
Dimensionless slip velocity
- We :
-
Weber number
- We L :
-
Weber number for liquid phase
- We G :
-
Weber number for gas phase
- α :
-
Modification coefficient
- μ :
-
Dynamic viscosity
- θ :
-
Incident angle
- β 1 :
-
Circumferential angle measured from the top of the horizontal tube
- β :
-
Circumferential angle
- τ :
-
Liquid flow rate on one side per unit length of cylinder
- τ 1 :
-
Flow rate of the liquid on one side of the tube
- ρ :
-
Density of the liquid
- ρ L :
-
Density of liquid
- ρ G :
-
Density of gas
- δ :
-
Liquid film thickness
- δ 0 :
-
Initial liquid film thickness
- ε r :
-
Dielectric constant
- σ :
-
Surface tension of the liquid
- Ω :
-
Disc spinning speed
- CFD:
-
Computational fluid dynamics
- DLAS:
-
Diode laser absorption spectroscopy
- LCDM:
-
Laser confocal displacement meter
- LDV:
-
Laser Doppler velocimeter
- LFDM:
-
Laser focus displacement meter
- LIF:
-
Laser induced fluorescence
- PLIF:
-
Planar laser induced fluorescence
- TAB:
-
Taylor analogy breakup
- TFB:
-
Turbulent fluidized bed
- TFCI:
-
Thin film colorimetric interferometry
- WFT:
-
Water film thickness
- Decel:
-
Decelerated condition
- G:
-
Gas phase
- L:
-
Liquid phase
- Steady:
-
Steady condition
References
Abdulkadir, M., Azzi, A., Zhao, D., Lowndes, I. S., Azzopardi, B. J. 2014. Liquid film thickness behaviour within a large diameter vertical 180° return bend. Chem Eng Sci, 107: 137–148.
Abdulkadir, M., Samson, J. N., Zhao, D., Okhiria, D. U., Hernandez-Perez, V. 2018. Annular liquid film thickness prediction in a vertical 180° return bend. Exp Therm Fluid Sci, 96: 205–215.
Åkesjö, A., Vamling, L., Sasic, S., Olausson, L., Innings, F., Gourdon, M. 2018. On the measuring of film thickness profiles and local heat transfer coefficients in falling films. Exp Therm Fluid Sci, 99: 287–296.
Al-Aufi, Y. A., Hewakandamby, B. N., Dimitrakis, G., Holmes, M., Hasan, A., Watson, N. J. 2019. Thin film thickness measurements in two phase annular flows using ultrasonic pulse echo techniques. Flow Meas Instrum, 66: 67–78.
Bai, D., Shibuya, E., Masuda, Y., Nishio, K., Nakagawa, N., Kato, K. 1995. Distinction between upward and downward flows in circulating fluidized beds. Powder Technol, 84: 75–81.
Berna, C., Escrivá, A., Muñoz-Cobo, J. L., Herranz, L. E. 2014. Review of droplet entrainment in annular flow: Interfacial waves and onset of entrainment. Prog Nucl Energ, 74: 14–43.
Bi, H.-T., Zhou, J., Qin, S.-Z., Grace, J. R. 1996. Annular wall layer thickness in circulating fluidized bed risers. Can J Chem Eng, 74: 811–814.
Bonilla-Riaño, A., Rodriguez, I. H., Bannwart, A. C., Rodriguez, O. M. H. 2015. Film thickness measurement in oil–water pipe flow using image processing technique. Exp Therm Fluid Sci, 68: 330–338.
Bonilla-Riaño, A., Velasco-Peña, H. F., Bannwart, A. C., Prasser, H. M., Rodriguez, O. M. H. 2019. Water film thickness measurement system for oil-water pipe flow. Flow Meas Instrum, 66: 86–98.
Bretherton, F. P. 1961. The motion of long bubbles in tubes. J Fluid Mech, 10: 166–188.
Cen, H., Lugt, P. M. 2019. Film thickness in a grease lubricated ball bearing. Tribol Int, 134: 26–35.
Chao, Q., Zhang, J.-H., Xu, B., Wang, Q. 2018. Multi-position measurement of oil film thickness within the slipper bearing in axial piston pumps. Measurement, 122: 66–72.
Chen, B.-W., Li, J.-S., Mao, F., Tian, R.-F. 2019. Numerical study on the characteristics of single wetted flat wire with single droplet impact under the disturbance of airflow. Nucl Eng Des, 345: 74–84.
Chen, B.-W., Tian, R.-F., Mao, F. 2020. Analysis of special phenomena of droplet impact on horizontal liquid film at low velocity. Ann Nucl Energy, 136: 107038.
Chen, X., Shen, S., Wang, Y., Chen, J., Zhang, J. 2015. Measurement on falling film thickness distribution around horizontal tube with laser-induced fluorescence technology. Int J Heat Mass Tran, 89: 707–713.
Cheng, Y.-S., Deng, K.-Y., Li, T. 2010. Measurement and simulation of wall-wetted fuel film thickness. Int J Therm Sci, 49: 733–739.
Conte, G., Azzopardi, B. J. 2003. Film thickness variation about a T-junction. Int J Multiphase Flow, 29: 305–328.
Donniacuo, A., Charnay, R., Mastrullo, R., Mauro, A. W., Revellin, R. 2015. Film thickness measurements for annular flow in minichannels: Description of the optical technique and experimental results. Exp Therm Fluid Sci, 69: 73–85.
Drosos, E. I. P., Paras, S. V., Karabelas, A. J. 2004. Characteristics of developing free falling films at intermediate Reynolds and high Kapitza numbers. Int J Multiphase Flow, 30: 853–876.
Estrada-Pérez, C. E., Hassan, Y. A., Tan, S.-C. 2011. Experimental characterization of temperature sensitive dyes for laser induced fluorescence thermometry. Rev Sci Instrum, 82: 074901.
Fairbrother, F., Stubbs, A. E. 1935. 119. Studies in electro-endosmosis. Part VI. The “bubble-tube” method of measurement. J Chem Soc: 527–529.
Fryza, J., Sperka, P., Krupka, I., Hartl, M. 2018. Effects of lateral harmonic vibrations on film thickness in EHL point contacts. Tribol Int, 117: 236–249.
Fu, Q.-F, Yang, L.-J, Qu, Y.-Y. 2011. Measurement of annular liquid film thickness in an open-end swirl injector. Aerospace Sci Technol, 15: 117–124.
Fukano, T., Furukawa, T. 1998. Prediction of the effects of liquid viscosity on interfacial shear stress and frictional pressure drop in vertical upward gas–liquid annular flow. Int J Multiphase Flow, 24: 587–603.
Ghiaasiaan, S. M., Wu, X., Sadowski, D. L., Abdel-Khalik, S. I. 1997. Hydrodynamic characteristics of counter-current two-phase flow in vertical and inclined channels: Effects of liquid properties. Int J Multiphase Flow, 23: 1063–1083.
Han, Y. B., Shikazono, N. 2009. Measurement of liquid film thickness in micro square channel. Int J Multiphase Flow, 35: 896–903.
Han, Y. B., Shikazono, N. 2010. The effect of bubble acceleration on the liquid film thickness in micro tubes. Int J Heat Fluid Fl, 31: 630–639.
Han, Y., Kanno, H., Ahn, Y. J., Shikazono, N. 2015. Measurement of liquid film thickness in micro tube annular flow. Int J Multiphase Flow, 73: 264–274.
Han, Y., Shikazono, N. 2009. Measurement of the liquid film thickness in micro tube slug flow. Int J Heat Fluid Fl, 30: 842–853.
Harris, A. T., Thorpe, R. B., Davidson, J. F. 2002. Characterisation of the annular film thickness in circulating fluidised-bed risers. Chem Eng Sci, 57: 2579–2587.
Henstock, W. H., Hanratty, T. J. 1976. The interfacial drag and the height of the wall layer in annular flows. AIChE J, 22: 990–1000.
Hori, K., Nakasatomi, M., Nishikawa, K., Sekoguchi, K. 1978. On ripple of annular two-phase flow: 3rd report. effect of liquid viscosity on characteristics of wave and interfacial friction factor. Transactions of the Japan Society of Mechanical Engineers, 44: 3847–3856.
Hou, H., Bi, Q., Ma, H., Wu, G. 2012. Distribution characteristics of falling film thickness around a horizontal tube. Desalination, 285: 393–398.
Ishii, M., Grolmes, M. A. 1975. Inception criteria for droplet entrainment in two-phase concurrent film flow. AIChE J, 21: 308–318.
Jablonka, K., Glovnea, R., Bongaerts, J. 2018. Quantitative measurements of film thickness in a radially loaded deep-groove ball bearing. Tribol Int, 119: 239–249.
Ju, P., Liu, Y., Ishii, M., Hibiki, T. 2018a. Prediction of rod film thickness of vertical upward co-current adiabatic flow in rod bundle. Ann Nucl Energy, 121: 1–10.
Ju, P., Yang, X., Schlegel, J. P., Liu, Y., Hibiki, T., Ishii, M. 2018b. Average liquid film thickness of annular air-water two-phase flow in 8×8 rod bundle. Int J Heat Fluid Fl, 73: 63–73.
Kim, M.-G., Choi, G. 2019. Accurate determination of two-dimensional thin film thickness in spectroscopic imaging reflectometer using color camera and tunable aperture. Opt Commun, 435: 75–80.
Kim, S. W., Kirbas, G., Bi, H., Lim, C., Grace, J. R. 2004. Flow structure and thickness of annular downflow layer in a circulating fluidized bed riser. Powder Technol, 142: 48–58.
Lewis, J. M., Wang, Y. 2018. Two-phase frictional pressure drop and water film thickness in a thin hydrophilic microchannel. Int J Heat Mass Tran, 127: 813–828.
Li, L.-G., Zhao, Z.-W., Zhu, J., Kwan, A. K. H., Zeng, K. L. 2018. Combined effects of water film thickness and polypropylene fibre length on fresh properties of mortar. Constr Build Mater, 174: 586–593.
Li, Y.-Z., Li, T., Zhang, H.-T., Sun, Q., Ying, W. 2017. LDV measurements of particle velocity distribution and annular film thickness in a turbulent fluidized bed. Powder Technol, 305: 578–590.
Luo, D., Ghiaasiaan, S. M. 1997. Liquid-side interphase mass transfer in cocurrent vertical two-phase channel flows. Int J Heat Mass Tran, 40: 641–655.
Mac Giolla Eain, M., Egan, V., Punch, J. 2013. Film thickness measurements in liquid–liquid slug flow regimes. Int J Heat Fluid Fl, 44: 515–523.
Mantripragada, V. T., Sarkar, S. 2017. Prediction of drop size from liquid film thickness during rotary disc atomization process. Chem Eng Sci, 158: 227–233.
Martínez-Galván, E., Ramos, J. C., Antón, R., Khodabandeh, R. 2011. Film thickness and heat transfer measurements in a spray cooling system with R134a. J Electron Packag, 133: 011002.
Mignot, G., Dupont, J., Paranjape, S., Ouldrebai, H., Bissels, W. M., Paladino, D., Prasser, H. M. 2018. Measurement of liquid films thickness in a condensing and re-evaporating environment using attenuation of near infrared light. Nucl Eng Des, 336: 64–73.
Morokuma, T., Utaka, Y. 2016. Variation of the liquid film thickness distribution between contacting twin air bubbles during the coalescence process in water and ethanol pools. Int J Heat Mass Tran, 98: 96–107.
Muramatsu, K., Youn, Y., Han, Y., Hasegawa, Y., Shikazono, N. 2015. Numerical study on the effect of initial flow velocity on liquid film thickness of accelerated slug flow in a micro tube. Int J Heat Fluid Fl, 54: 77–86.
Niese, S., Quodbach, J. 2018. Application of a chromatic confocal measurement system as new approach for in-line wet film thickness determination in continuous oral film manufacturing processes. Int J Pharmaceut, 551: 203–211.
Obert, P., Füßer, H.-J., Bartel, D. 2019. Oil distribution and oil film thickness within the piston ring-liner contact measured by laser-induced fluorescence in a reciprocating model test under starved lubrication conditions. Tribol Int, 129: 191–201.
Olgac, U., Muradoglu, M. 2013. Effects of surfactant on liquid film thickness in the Bretherton problem. Int J Multiphase Flow, 48: 58–70.
Patel, R. S., Weibel, J. A., Garimella, S. V. 2017. Characterization of liquid film thickness in slug-regime microchannel flows. Int J Heat Mass Trans, 115: 1137–1143.
Patience, G. S., Chaouki, J. 1993. Gas phase hydrodynamics in the riser of a circulating fluidized bed. Chem Eng Sci, 48: 3195–3205.
Qi, R.-H, Lu, L., Yang, H.-X., Qin, F. 2013. Investigation on wetted area and film thickness for falling film liquid desiccant regeneration system. Appl Energ, 112: 93–101.
Qiu, Q.-G., Zhu, X.-J., Mu, L., Shen, S. 2015. Numerical study of falling film thickness over fully wetted horizontal round tube. Int J Heat Mass Tran, 84: 893–897.
Schmidt, A., Kühnreich, B., Kittel, H., Tropea, C., Roisman, I. V., Dreizler, A., Wagner, S. 2018. Laser based measurement of water film thickness for the application in exhaust after-treatment processes. Int J Heat Fluid Fl, 71: 288–294.
Schubring, D., Ashwood, A. C., Shedd, T. A., Hurlburt, E. T. 2010a. Planar laser-induced fluorescence (PLIF) measurements of liquid film thickness in annular flow. Part I: Methods and data. Int J Multiphase Flow, 36: 815–824.
Schubring, D., Shedd, T. A. 2011. A model for pressure loss, film thickness, and entrained fraction for gas–liquid annular flow. Int J Heat Fluid Fl, 32: 730–739.
Schubring, D., Shedd, T. A., Hurlburt, E. T. 2010b. Planar laser-induced fluorescence (PLIF) measurements of liquid film thickness in annular flow. Part II: Analysis and comparison to models. Int J Multiphase Flow, 36: 825–835.
Shri Vignesh, K., Vasudevan, C., Arunkumar, S., Suwathy, R., Venkatesan, M. 2018. Laser induced fluorescence measurement of liquid film thickness and variation in Taylor flow. Eur J Mech B, 70: 85–92.
Sun, Y.-H., Guo, C.-H., Jiang, Y. Y., Wang, T., Zhang, L. 2018. Transient film thickness and microscale heat transfer during flow boiling in microchannels. Int J Heat Mass Tran, 116: 458–470.
Tan, S., Gao, P., Su, G. 2008. Experimental research on natural circulation complex oscillations under rolling motion conditions. Atomic Energy Science and Technology, 42: 1007–1011.
Tan, S.-C., Su, G.-H., Gao, P.-Z. 2009. Heat transfer model of singlephase natural circulation flow under a rolling motion condition. Nucl Eng Des, 239: 2212–2216.
Tatterson, D. F., Dallman, J. C., Hanratty, T. J. 1977. Drop sizes in annular gas–liquid flows. AIChE J, 23: 68–76.
Tiwari, R., Damsohn, M., Prasser, H.-M. 2014. The effect of initial flow velocity on the liquid film thickness in micro tube accelerated slug flow. Flow Meas Instrum, 40: 124–132.
Wang, B., Chen, B.-W., Tian, R.-F. 2019a. Review of research progress on flow and rupture characteristics of liquid film on corrugated plate wall. Ann Nucl Energy, 132: 741–751.
Wang, B., Chen, B.-W., Tian, R.-F. 2020. Analysis of fluctuation and breakdown characteristics of liquid film on corrugated plate wall. Ann Nucl Energy, 135: 106946.
Wang, B., Tian, R.-F. 2019a. Investigation on flow and breakdown characteristics of water film on vertical corrugated plate wall. Ann Nucl Energy, 127: 120–129.
Wang, B., Tian, R.-F. 2019b. Judgement of critical state of water film rupture on corrugated plate wall based on SIFT feature selection algorithm and SVM classification method. Nucl Eng Des, 347: 132–139.
Wang, B., Tian, R.-F. 2019c. Study on characteristics of water film breakdown on the corrugated plate wall under the horizontal shear of airflow. Nucl Eng Des, 343: 76–84.
Wang, B., Tian, R.-F. 2019d. Study on fluctuation feature and breakdown characteristic of water film on the wall of corrugated plate. Int J Heat Mass Tran, 143: 118501.
Wang, J., Chen, X., Lu, T., Chen, X., Shen, S., Liu, B. 2019b. Three-dimensional film thickness distribution of horizontal tube falling film with column flow. Appl Therm Eng, 154: 140–149.
Wang, R.-L., Lee, B. A., Lee, J. S., Kim, K. Y., Kim, S. 2012. Analytical estimation of liquid film thickness in two-phase annular flow using electrical resistance measurement. Appl Math Model, 36: 2833–2840.
Werther, J. 1994. Fluid mechanics of large-scale CFB units. In: Circulating Fluidized Bed Technology IV. Avidan, A. A. Ed. New York: AIChE, 1–14.
Xue, T., Yang, L., Ge, P., Qu, L. 2015. Error analysis and liquid film thickness measurement in gas–liquid annular flow. Optik, 126: 2674–2678.
Yang, H.-N., Wei, W., Su, M.-X., Chen, J., Cai, X. 2018. Measurement of liquid water film thickness on opaque surface with diode laser absorption spectroscopy. Flow Meas Instrum, 60: 110–114.
Youn, Y. J., Han, Y., Shikazono, N. 2018. Liquid film thicknesses of oscillating slug flows in a capillary tube. Int J Heat Mass Tran, 124: 543–551.
Youn, Y. J., Muramatsu, K., Han, Y., Shikazono, N. 2015. The effect of initial flow velocity on the liquid film thickness in micro tube accelerated slug flow. Int J Multiphase Flow, 73: 108–117.
Youn, Y. J., Muramatsu, K., Han, Y., Shikazono, N. 2016. The effect of bubble deceleration on the liquid film thickness in microtubes. Int J Heat Fluid Fl, 58: 84–92.
Yu, Y.-X, Ma, L., He, H.-Y., Zheng, Y., Ma, Y. 2017. Research of non-contact measurement for high viscous fluid falling film thickness on spherical series surface. Measurement, 101: 1–8.
Zhang, H., Liu, Q., Qin, B., Bo, H. 2015a. Modeling droplet-laden flows in moisture separators using k-d trees. Ann Nucl Energy, 75: 452–461.
Zhang, H., Liu, Q., Qin, B., Bo, H. 2015b. Simulating particle collision process based on Monte Carlo method. J Nucl Sci Technol, 52: 1393–1401.
Zhang, S., Liu, J.-X., Zuo, Z.-X., Zhang, Y. 2017. An analytical investigation of oil film thickness for the apex seal in a small Wankel rotary engine. Tribol Int, 116: 383–393.
Zhang, W., Johnsson, F., Leckner, B. 1995. Fluid-dynamic boundary layers in CFB boilers. Chem Eng Sci, 50: 201–210.
Zhao, C.-Y, Ji, W.-T., Jin, P.-H., Zhong, Y., Tao, W. 2018. Hydrodynamic behaviors of the falling film flow on a horizontal tube and construction of new film thickness correlation. Int J Heat Mass Tran, 119: 564–576.
Zhou, D.-W., Gambaryan-Roisman, T., Stephan, P. 2009. Measurement of water falling film thickness to flat plate using confocal chromatic sensoring technique. Exp Therm Fluid Sci, 33: 273–283.
Acknowledgements
The authors would like to acknowledge the financial support provided by the Ph.D. Student Research and Innovation Fund of the Fundamental Research Funds for the Central Universities, the Fundamental Research Funds for the Central Universities, the National Natural Science Foundation of China (No. 51676052), and Chinese Universities Scientific Fund.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Wang, B., Ke, B., Chen, B. et al. A technical review of research progress on thin liquid film thickness measurement. Exp. Comput. Multiph. Flow 2, 199–211 (2020). https://doi.org/10.1007/s42757-019-0051-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s42757-019-0051-9