1 Introduction

In the conventional press-and-sinter process of powder metallurgy, green parts obtained by cold compaction are sintered to form dense bulk parts via the metallic bonding between the powder particles. This occurs through the formation and growth of the so-called necks, promoted by mass transport phenomena towards the inter-particle contact region. When the source of atoms flowing towards the neck region lies within the bulk of the particles, sintering is accomplished by shrinkage, leading to an overall dimensional change of the parts.[1] The prediction of such a dimensional change is of great importance, since one of the key attributes of powder metallurgy is net shape sintering of complex parts with excellent dimensional precision, without any need of machining.

In the literature, various approaches are used to investigate sintering at different scales. The microscale approach is based on the classical theory of sintering that describes the growth of the sintering necks as a function of temperature and time, due to the driving force linked to the free surface area of the powders and to the occurrence of various mass transport mechanisms. Shrinkage kinetics laws are proposed for each mass transport mechanism, based on the assumption that powder particles are rigid spheres in a point contact at the beginning of the sintering process.[2,3,4,5,6,7,8,9,10,11,12,13,14,15,16] The effect of the initial cold compaction is therefore mostly neglected. The macroscale approach is utilized in most modern studies, where the behavior of the porous body during sintering is described using continuum mechanics.[17,18,19,20,21,22,23,24,25,26] This approach is similar to the theories of plasticity of porous bodies[27,28,29,30,31,32,33,34,35,36] employed for modeling of powder pressing. It investigates the dimensional changes of porous bodies as a consequence of the flow of material from the solid matrix containing pores, and treats sintering as a rheological phenomenon.[37,38]

Solid state sintering is a thermally activated process, where the contributions from several mass transport mechanisms, such as surface, grain boundary, and lattice diffusion, are responsible for neck growth and powder consolidation. Surface diffusion is mostly accountable for neck growth and the consequent material strengthening.[13,39,40,41] Different works[42,43,44] show, in the case of sinter-forging and constrained sintering, that the sintering shrinkage of powders can be anisotropic, due to the sintering conditions. Otherwise in the case of free sintering it has been demonstrated that the anisotropic shrinkage is a consequence of the anisotropic plastic deformation of the particles promoted by uniaxial cold compaction.[45] Zavaliangos et al.[46,47] have considered anisotropy to be a consequence of the different flattening and arrangement of particle-particle contacts, the density of pores present on the grain boundaries, and oxide fragmentation. In a previous work, Molinari et al.[48] proposed that anisotropy is due to the effect of the structural defect densities introduced by cold compaction on bulk diffusivity. This hypothesis was further developed by some of the authors of the present paper; with studies on the kinetics of the diffusion mechanisms,[48,49] microstructural analysis on the deformed powder particles after cold compaction,[50,51] and an initial investigation on the effect of defects on diffusion.[52] One result indicated that shrinkage kinetics are influenced by the dislocations formed in the powder particles during cold compaction (structural activity). Namely, the inhomogeneous dislocation density distribution inside the particles leads to anisotropic shrinkage kinetics, characterized by greater densification parallel to the compaction direction (longitudinal) than perpendicular to the compaction direction (transverse).

Grain boundary diffusion is generally considered to be the principal mass transfer mechanism in solid state sintering.[40,44,46,53] However, taking into account the large strains present within the particles of the initial green compact and the effect of dislocations on diffusivity, especially during the early stages of sintering, volume diffusion can be of greater importance. The effect of dislocations on bulk diffusivity is displayed through the dislocation pipe diffusion model, according to which bulk diffusivity is proportional to the dislocation density, as reported by Hart.[54,55] Based on Hart’s equation for dislocation pipe diffusion and the equations of the classical theory of sintering, Molinari et al.[49] estimated the dislocation density in the inter-particle contact regions, obtaining quite high values, comparable to those typical of heavily cold worked metals. However, the classic theories of sintering, as mentioned above, do not consider the actual geometry of the contact regions, which is different than a single point, and occupies an area that changes depending on the contact direction. These geometric aspects can be analyzed and quantified in the metallographic sections using image analysis software. Specifically, for the measurement of the contact lengths, a general method, including a preliminary correction factor due to “distortion” and measurement imprecisions, was demonstrated in previous studies.[50,51] Such microstructural features of the green parts may further contribute to the anisotropic dimensional change (geometric activity), since atoms flowing towards the neck surface originate in the inter-particle regions. Other authors investigated the influence of shape and orientation of particles and pores on shrinkage.[56,57,58,59]

In the present work, the continuum theory of sintering is used to develop a micromechanical model of the anisotropic dimensional change, inclusive of both the contributions of structural and geometric activities, namely presence of dislocations and of the flattened porous material morphology. The model proposed by Olevsky et al.[44] is adapted to this case, in which volume diffusion is the principal mass transport mechanism. This model is applied to the sintering of pure iron. Such a material choice was made in order to rule out potential complications arising from the presence of additional alloying elements altering the diffusivity of the powder compact, and to rely on the wide availability of physical and chemical data on pure iron in the literature. The dislocation densities along the different directions are calculated by plugging the experimental data on the isothermal shrinkage of the sample at the different sintering temperatures into the derived equations. The validity of the model is then assessed by experimentally estimating dislocation densities with two different methods. The first method is based on the correlation between dislocation densities and hardness measured via nanoindentation, as proposed by Nix and Gao.[60,61,62] The second method relies on EBSD lattice curvature data.[63,64,65] The comparison between calculated and measured dislocation density values is accompanied by a parameter sensitivity study, and the reliability of the model is discussed.

2 Model Formulation

The proposed micromechanical model is the adaptation of the work of Olevsky et al.[44] to the volume diffusion case. In the earlier work by Olevsky et al., it was assumed that the main mass transfer mechanism was grain boundary diffusion, and diffusion was related to micromechanical considerations through the chemical potential, according to Johnson.[11] In the present case, the induced plastic deformation activates dislocation pipe diffusion mechanisms, and therefore leads to noticeably enhanced volume diffusivity.[52]

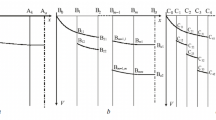
Therefore, for the study domain definition, the 2-D geometry employed in Olevsky et al.[44] is extended to the 3-D case. The porous structure of the material can be schematized with an assembly of simple-packed, oriented, prismatic particles, with ellipsoidal pores at the junctions between them, as depicted in Figure 1(a).

Fig. 1
figure 1

Schematics of the study domain representative of the porous material’s structure: (a) 3-D representation, where δ is the inter-particle boundary thickness; (b) 2-D view with micromechanics parameters designation

Full size image

A representative unit cell for the micromechanical study definition is chosen to be a cluster of eight particles with a central lenticular pore. Note that, due to the symmetry of the structure, it is sufficient to only take into consideration 1/8 of each particle, accordingly to the representation given in Figure 1(a). The prismatic shape is a good approximation for powder particles that have been flattened by cold compaction and allows a simple definition of all the geometric parameters that influence the shrinkage kinetics. As depicted in Figure 1(a), the y-axis is defined oriented along the pre-sintering cold compaction direction (longitudinal direction), and thus x and z are defined as transverse directions. The notations, a, b and c define the semi-contact of the particles along x, z and y, respectively, and ap, bp and cp the semi-axes of the pores in the same directions. In this coordinate system, the geometric features along the x and z direction are equivalent (a = b, ap = bp), and the semi-axes and contact along x and z are greater than the those along y (a > c, ap > cp). This geometry represents transversely isotropic symmetry.[45]

The radii of curvature ra, rb, and rc for the ellipsoidal pores are thus defined as follows:

$$ r_{a} = r_{b} = {\raise0.7ex\hbox{${c_{\text{p}}^{2} }$} \!\mathord{\left/ {\vphantom {{c_{\text{p}}^{2} } {a_{\text{p}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${a_{\text{p}} }$}};\;r_{c} = {\raise0.7ex\hbox{${a_{\text{p}}^{2} }$} \!\mathord{\left/ {\vphantom {{a_{\text{p}}^{2} } {c_{\text{p}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${c_{\text{p}} }$}} $$
(1)

and the surface area of the pore is given by

$$ S_{\text{p}} \approx 2\pi \left( {a_{\text{p}}^{2} + c_{\text{p}}^{2} \frac{{\tanh^{ - 1} \left( {\sin \left( {\cos^{ - 1} \left( {{\raise0.7ex\hbox{${c_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{c_{\text{p}} } {a_{\text{p}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${a_{\text{p}} }$}}} \right)} \right)} \right)}}{{\sin \left( {\cos^{ - 1} \left( {{\raise0.7ex\hbox{${c_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{c_{\text{p}} } {a_{\text{p}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${a_{\text{p}} }$}}} \right)} \right)}}} \right) $$
(2)

As a result of this equivalence along the two transverse directions, the model is hereby developed in the reference system defined by the axes x and y, as shown in Figure 1(b).

According to Johnson,[11] at the inter-particle boundaries, the following normal stress distributions exist:

$$ \left\{ {\begin{array}{*{20}l} {\sigma_{x} = b_{1x} \cdot y^{2} + b_{2x} \cdot y + b_{3x} } \\ {\sigma_{y} = b_{1y} \cdot x^{2} + b_{2y} \cdot x + b_{3y} } \\ \end{array} } \right. $$
(3)

Due to the geometry of the study domain, the boundary conditions that these should satisfy for the x direction are

$$ \left\{ {\begin{array}{l} {\int_{0}^{c} {\sigma_{x} {\text{d}}x = - \gamma_{\text{sv}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} } \\ {\sigma_{x} \left( c \right) = {\raise0.7ex\hbox{${\gamma_{\text{sv}} }$} \!\mathord{\left/ {\vphantom {{\gamma_{\text{sv}} } {r_{\text{c}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${r_{\text{c}} }$}}} \\ {\nabla \sigma_{x} \left( 0 \right) = \frac{{\partial \sigma_{x} }}{\partial y}\left( {y = 0} \right) = 0} \\ \end{array} } \right. $$
(4)

where γsv (N/m) is the surface energy and ϕ is the dihedral angle. The dihedral angle is related to surface and grain boundary energy γss (N/m) as follows:

$$ \gamma_{\text{ss}} = 2 \cdot \gamma_{\text{sv}} \cos \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right) $$
(5)

The three equations in Eq. [4] represent the following: the balance of the forces acting at inter-particle junctions with the free surface tension of the pore, the normal stress in correspondence of the pore surface (y = c), and the symmetry of the stress distribution.

With these boundary conditions, the values of the coefficients b1x, b2x, and b3x [3] are obtained:

$$ \left\{ {\begin{array}{l} {b_{1x} = {\raise0.7ex\hbox{${3\gamma_{\text{sv}} }$} \!\mathord{\left/ {\vphantom {{3\gamma_{\text{sv}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {c^{2} \cdot r_{c} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {c^{2} \cdot r_{c} } \right)}$}} - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {c^{3} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${c^{3} }$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right]} \\ {b_{2x} = 0} \\ {b_{3x} = \gamma_{\text{sv}} \left[ {{\raise0.7ex\hbox{$3$} \!\mathord{\left/ {\vphantom {3 {\left( {2 \cdot c} \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {2 \cdot c} \right)}$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right) - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {2 \cdot r_{c} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {2 \cdot r_{c} } \right)}$}}} \right]} \\ \end{array} } \right. $$
(6)

Thus, the normal stress distribution along the transverse direction becomes

$$ \sigma_{x} = 3{\raise0.7ex\hbox{${\gamma_{\text{sv}} }$} \!\mathord{\left/ {\vphantom {{\gamma_{\text{sv}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\left\{ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {c^{2} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${c^{2} }$}}\left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {r_{c} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${r_{c} }$}} - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 c}}\right.\kern-0pt} \!\lower0.7ex\hbox{$c$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right] \cdot y^{2} + \left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 c}}\right.\kern-0pt} \!\lower0.7ex\hbox{$c$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right) - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {3 \cdot r_{c} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {3 \cdot r_{c} } \right)}$}}} \right]} \right\} $$
(7)

An analogous procedure can be applied in the longitudinal direction to obtain

$$ \sigma_{x} = 3{\raise0.7ex\hbox{${\gamma_{\text{sv}} }$} \!\mathord{\left/ {\vphantom {{\gamma_{\text{sv}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\left\{ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {a^{2} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${a^{2} }$}}\left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {r_{a} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${r_{a} }$}} - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 a}}\right.\kern-0pt} \!\lower0.7ex\hbox{$a$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right] \cdot x^{2} + \left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 a}}\right.\kern-0pt} \!\lower0.7ex\hbox{$a$}} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right) - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {3 \cdot r_{a} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {3 \cdot r_{a} } \right)}$}}} \right]} \right\} $$
(8)

As anticipated, volume diffusion enhanced by dislocation activity is considered as the prevailing mass transport mechanism. Accordingly, the fluxes of matter in the x and y directions (J V x and J V y , mol/m2 s) are proportional to the chemical potential gradient, given by

$$ \left\{ {\begin{array}{*{20}l} {J_{x}^{\text{V}} = {\raise0.7ex\hbox{${ - D_{{{\text{eff,}}x}}^{\text{V}} }$} \!\mathord{\left/ {\vphantom {{ - D_{{{\text{eff,}}x}}^{\text{V}} } {\left( {k \cdot T \cdot \varOmega } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {k \cdot T \cdot \varOmega } \right)}$}} \cdot \frac{\partial \mu }{\partial x}} \\ {J_{y}^{\text{V}} = {\raise0.7ex\hbox{${ - D_{{{\text{eff,}}y}}^{\text{V}} }$} \!\mathord{\left/ {\vphantom {{ - D_{{{\text{eff,}}y}}^{\text{V}} } {\left( {k \cdot T \cdot \varOmega } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {k \cdot T \cdot \varOmega } \right)}$}} \cdot \frac{\partial \mu }{\partial y}} \\ \end{array} } \right. $$
(9)

where k is the Boltzmann’s constant; T (K) is the absolute temperature; Ω (m3) is the atomic volume; µ (kJ/mol) is the chemical potential; and D Veff, x and D Veff, y (m2/s) are the effective volume diffusion coefficients in the transverse and longitudinal directions, respectively. Hart[54] has shown that in presence of dislocation pipe diffusion mechanisms, the coefficient of bulk diffusion increases proportionally to the dislocation density. Due to the anisotropic plastic deformation of the particles, the value for pipe diffusivity is different in x and y:

$$ \left\{ {\begin{array}{*{20}l} {D_{{{\text{eff,}}x}}^{\text{V}} = D_{\text{V}} \left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,x} \cdot {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)} \\ {D_{{{\text{eff,}}y}}^{\text{V}} = D_{\text{V}} \left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,y} \cdot {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)} \\ \end{array} } \right. $$
(10)

where DV and DP (m2/s) are the coefficient of volume diffusion and pipe diffusion in equilibrium conditions, respectively; rp (μm) is the pipe radius; dd,x and dd,y (1/m2) are the dislocation densities along the two directions, x and y, respectively.

The relation between chemical potential and stresses is given by

$$ \left\{ {\begin{array}{*{20}l} {\frac{\partial \mu }{\partial x} = - \varOmega \cdot \frac{{\partial \sigma_{y} }}{\partial x}} \\ {\frac{\partial \mu }{\partial y} = - \varOmega \cdot \frac{{\partial \sigma_{x} }}{\partial y}} \\ \end{array} } \right. $$
(11)

Introducing Eqs. [10] and [11] into [9], the diffusion fluxes are given by

$$ \left\{ {\begin{array}{*{20}l} {J_{x}^{\text{V}} = D_{\text{V}} \cdot \frac{{\left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,x} {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)}}{{\left( {k \cdot T} \right)}} \cdot \frac{{\partial \sigma_{y} }}{\partial x}} \\ {J_{y}^{\text{V}} = D_{\text{V}} \cdot \frac{{\left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,y} {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)}}{{\left( {k \cdot T} \right)}} \cdot \frac{{\partial \sigma_{x} }}{\partial y}} \\ \end{array} } \right. $$
(12)

Substituting expressions [7] and [8] in [12] the diffusion fluxes can be rewritten as

$$ \left\{ {\begin{array}{*{20}l} {J_{x}^{\text{V}} = 3 \cdot D_{\text{V}} \cdot \frac{{\left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,x} {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)}}{{\left( {k \cdot T} \right)}} \cdot \gamma_{\text{sv}} \cdot {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 a}}\right.\kern-0pt} \!\lower0.7ex\hbox{$a$}} \cdot \left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {r_{a} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${r_{a} }$}} - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 a}}\right.\kern-0pt} \!\lower0.7ex\hbox{$a$}}\sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right]} \\ {J_{y}^{\text{V}} = 3 \cdot D_{\text{V}} \cdot \frac{{\left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,y} {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right)}}{{\left( {k \cdot T} \right)}} \cdot \gamma_{\text{sv}} \cdot {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 c}}\right.\kern-0pt} \!\lower0.7ex\hbox{$c$}} \cdot \left[ {{\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {r_{c} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${r_{c} }$}} - {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 c}}\right.\kern-0pt} \!\lower0.7ex\hbox{$c$}}\sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right]} \\ \end{array} } \right. $$
(13)

On the other hand, the diffusional fluxes are proportional to the change in the contact lengths between the powder particles, Δx and Δy as follows:

$$ \left\{ {\begin{array}{*{20}l} {J_{x}^{\text{V}} \left( a \right) = - {\raise0.7ex\hbox{${{\text{d}}\left( {\Delta_{y} } \right)}$} \!\mathord{\left/ {\vphantom {{{\text{d}}\left( {\Delta_{y} } \right)} {{\text{d}}t}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${{\text{d}}t}$}} \cdot \frac{{a + a_{\text{p}} }}{{\varOmega \cdot {\raise0.7ex\hbox{${S_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{S_{\text{p}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} \cdot \delta } \\ {J_{y}^{\text{V}} \left( c \right) = - {\raise0.7ex\hbox{${{\text{d}}\left( {\Delta_{x} } \right)}$} \!\mathord{\left/ {\vphantom {{{\text{d}}\left( {\Delta_{x} } \right)} {{\text{d}}t}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${{\text{d}}t}$}} \cdot \frac{{c + c_{\text{p}} }}{{\varOmega \cdot {\raise0.7ex\hbox{${S_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{S_{\text{p}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}} \cdot \delta } \\ \end{array} } \right. $$
(14)

where δ (nm) is the grain boundary thickness.

The strain rates along x and y can be written as

$$ \left\{ {\begin{array}{*{20}l} {\dot{\varepsilon }_{x} = {\raise0.7ex\hbox{${{\text{d}}\left( {\Delta_{x} } \right)}$} \!\mathord{\left/ {\vphantom {{{\text{d}}\left( {\Delta_{x} } \right)} {{\text{d}}t}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${{\text{d}}t}$}} \cdot {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {c + c_{\text{p}} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {c + c_{\text{p}} } \right)}$}}} \\ {\dot{\varepsilon }_{y} = {\raise0.7ex\hbox{${{\text{d}}\left( {\Delta_{y} } \right)}$} \!\mathord{\left/ {\vphantom {{{\text{d}}\left( {\Delta_{y} } \right)} {{\text{d}}t}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${{\text{d}}t}$}} \cdot {\raise0.7ex\hbox{$1$} \!\mathord{\left/ {\vphantom {1 {\left( {a + a_{\text{p}} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {a + a_{\text{p}} } \right)}$}}} \\ \end{array} } \right. $$
(15)

Therefore, combining [14] and [15], a relationship between diffusion fluxes and shrinkage rates is obtained:

$$ \left\{ {\begin{array}{*{20}l} {\dot{\varepsilon }_{x} = - \frac{{J_{y}^{\text{V}} \left( c \right)}}{{\left( {a + a_{\text{p}} } \right)}} \cdot {\raise0.7ex\hbox{${\left( {\varOmega \cdot \frac{{S_{\text{p}} }}{2}} \right)}$} \!\mathord{\left/ {\vphantom {{\left( {\varOmega \cdot \frac{{S_{\text{p}} }}{2}} \right)} {\left[ {\left( {c + c_{\text{p}} } \right) \cdot \delta } \right]}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left[ {\left( {c + c_{\text{p}} } \right) \cdot \delta } \right]}$}}} \\ {\dot{\varepsilon }_{x} = - \frac{{J_{x}^{\text{V}} \left( a \right)}}{{\left( {c + c_{\text{p}} } \right)}} \cdot {\raise0.7ex\hbox{${\left( {\varOmega \cdot \frac{{S_{\text{p}} }}{2}} \right)}$} \!\mathord{\left/ {\vphantom {{\left( {\varOmega \cdot \frac{{S_{\text{p}} }}{2}} \right)} {\left[ {\left( {a + a_{\text{p}} } \right) \cdot \delta } \right]}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left[ {\left( {a + a_{\text{p}} } \right) \cdot \delta } \right]}$}}} \\ \end{array} } \right. $$
(16)

Let us consider the case of free pressure-less sintering. Plugging Eqs. [13] into [16], the strain rates can be written as

$$ \left\{ {\begin{array}{*{20}l} {\dot{\varepsilon }_{x}^{f.s} = - 3\frac{{D_{\text{V}} \left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,y} {\raise0.7ex\hbox{${D_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{D_{\text{p}} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right) \cdot \varOmega }}{k \cdot T \cdot \delta } \cdot \gamma_{sv} \cdot \frac{{{\raise0.7ex\hbox{${S_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{S_{\text{p}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}}{{c \cdot \left( {a + a_{\text{p}} } \right) \cdot \left( {c + c_{\text{p}} } \right)}} \cdot \left[ {\frac{1}{{r_{c} }} - \frac{1}{c} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right]} \\ {\dot{\varepsilon }_{y}^{f.s} = - 3\frac{{D_{\text{V}} \left( {1 + \pi \cdot r_{\text{p}}^{2} \cdot d_{d,x} {\raise0.7ex\hbox{${D_{p} }$} \!\mathord{\left/ {\vphantom {{D_{p} } {D_{\text{V}} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${D_{\text{V}} }$}}} \right) \cdot \varOmega }}{k \cdot T \cdot \delta } \cdot \gamma_{sv} \cdot \frac{{{\raise0.7ex\hbox{${S_{\text{p}} }$} \!\mathord{\left/ {\vphantom {{S_{\text{p}} } 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}}}{{a \cdot \left( {c + c_{\text{p}} } \right) \cdot \left( {a + a_{\text{p}} } \right)}} \cdot \left[ {\frac{1}{{r_{a} }} - \frac{1}{a} \cdot \sin \left( {{\raise0.7ex\hbox{$\phi $} \!\mathord{\left/ {\vphantom {\phi 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}} \right)} \right]} \\ \end{array} } \right. $$
(17)

These strain rate equations can be used to obtain expressions of the shrinkage rates as functions of the geometric parameters of the porous material, diffusivities, and deformation state.

3 Experimental Procedure for Model Validation

In order to validate the proposed micromechanical model, a variety of experimental data were collected. The free-sintering strain rates were obtained from dilatometric tests, the geometric features with image analysis of scanning electron microscopy images, and detailed information on iron material properties were found in the literature. The dislocation densities in the transverse and longitudinal directions are calculated based on the work of Kuhn and Downey.[15] These dislocation density values become the model validation parameters, comparing these calculated values with experimental values. Dislocation densities can be experimentally derived with both nanoindentation and from electron backscatter diffraction (EBSD) techniques. Nanoindentation allows the estimation of the overall dislocation densities (statistically stored dislocations (SSDs) and geometrically necessary dislocations (GNDs) with the only exception being those due to the indentation process itself), but it is limited in accuracy when it comes to selecting the most appropriate areas for the measurements. On the other hand, EBSD produces detailed misorientation maps, from which dislocation density distributions can be derived, but such values only include GNDs. Thus, in order to obtain accurate experimental data for our model validation procedure, both nanoindentation and EBSD were performed. In the results section (Section IV), a good correlation between modeling and experiments is achieved, while in the following sub-sections the details of the various experimental procedures are described.

3.1 Dilatometric Analysis

A water atomized iron powder (d25 < 45 µm) was mixed with 0.6 wt pct amide wax as lubricant. Charpy bars [55 × 10 × 10 (mm)] with 6.9 g/cm3 green density were produced by uniaxial cold compaction (Höganäs AB, Höganäs, Sweden). After debinding at 500 °C for 1 hour in an Ar atmosphere, parallelepiped-shaped samples [10 × 5 × 5 (mm)] were cut from the Charpy bars along the compaction direction (y or longitudinal direction) and in the compaction plane (x or transverse direction) as shown in Figure 2.

Fig. 2
figure 2

Dilatometry samples for the longitudinal (y, in red) and transverse (x, in blue) shrinkage rates analysis, as derived from cold-pressed Charpy bars. The direction of uniaxial compaction is marked (Color figure online)

Full size image

These samples were used in dilatometric experiments, in which the shrinkage kinetics along the two directions could be measured. The specimens cut in such a way that the long side corresponded to the y-axis in the Charpy bars (red in Figure 2) provided data on the longitudinal shrinkage rates (\( \dot{\varepsilon }_{y}^{f.s.} \)), while the specimens with long sides aligned with the x-axis of the Charpy bar (blue in Figure 2) were used for the transverse shrinkage rates (\( \dot{\varepsilon }_{x}^{f.s.} \)).

The samples were isothermally sintered for 1 hour at different temperatures in the range between 640 °C and 1010 °C, reached with a heating rate of 30 °C/min. An example of dilatometric curve is shown in Figure 3, where the different shrinkages along the two directions are presented and highlighted at the bottom.

Fig. 3
figure 3

Example of dilatometric curve for sintering with holding at 960 °C: (a) temperature regime and shrinkages in the longitudinal (y) and transverse (x) direction for the entire processing route; (b) shrinkage curves during the isothermal holding time

Full size image

The additional steep changes in shrinkage, both along x and y direction, marked in the top part of the same Figure 3 before and after the isotherm segment, are related to well-known phase changes in iron. Specifically, at the Curie temperature (T = 770 °C), the magnetic transformation leads to a steep increase of iron’s self-diffusivity, and therefore to a shrinkage enhancement (and correspondingly to a slight contraction during cooling).[66,67,68] At 910 °C, on the other hand, the austenitic transformation also leads to a shrinkage increase during heating and to a volume expansion upon cooling.[52]

3.2 Image Analysis

The surfaces of the sintered samples were prepared according to the standard metallographic procedure and were observed in a scanning electron microscope (FEI XL30 ESEM). The obtained micrographs were then processed with the image analysis software ImageJ®, in order to measure the various geometric characteristics of the microstructure of the porous material, namely particle and pore semi-axes.

The method used to evaluate the extension of the particle semi-contacts a and c is described in a previous work.[49] Figure 4 shows an example of identification of the semi-axis of a particle.

Fig. 4
figure 4

Individuation of inter-particle contacts length (L) and inclination (λ) from image analysis of SEM micrographs

Full size image

The length L and the inclination λ with respect to the horizontal direction (lying in the compaction plane) of the inter-particle contact lines were measured, and the projections along directions x and y were calculated as

$$ \left\{ {\begin{array}{*{20}l} {a = {\raise0.7ex\hbox{$L$} \!\mathord{\left/ {\vphantom {L 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\cos \lambda } \\ {c = {\raise0.7ex\hbox{$L$} \!\mathord{\left/ {\vphantom {L 2}}\right.\kern-0pt} \!\lower0.7ex\hbox{$2$}}\sin \lambda } \\ \end{array} } \right. $$
(18)

The pores semi-axes ap and cp were calculated based on the schematic presented in Figure 5.

Fig. 5
figure 5

Individuation of the pores’ geometrical parameters from image analysis of SEM micrographs

Full size image

The shape of each pore is approximated as an ellipse with an area equivalent to its extension, according to the stereological approach. This allows obtaining reasonable mean values of the radii, which take into account both the small radii of pores at junctions with grain boundaries and the lower curvatures of larger voids. The average pore curvature values at grain boundary/pore junctions are related to the dihedral angle, which is generally well known for a certain material system. Note, therefore, that even though the choice of the elliptical morphology is only an approximation, an appropriate value of the dihedral angle (ϕ = 157 deg = 2.75 rad) has been taken into account in the model, as specified in Eq. [5], and this leads to the results comparable with the experimental values reported in literature.[69] The inclination of its major axis with respect to the horizontal direction of the image (β) provides the orientation of the pore. The pores that are not fully captured in the image are excluded from the analysis. The software returns the values of axes of the ellipses (M and m) and of the angle β. By using β to project the ellipses axes along longitudinal and transverse directions, the pores semi-axes ap and cp are calculated as

$$ \left\{ {\begin{array}{l} {0 \le \beta < 45\;{\text{deg}} :a_{\text{p}} = M \cdot \cos \;\beta ;_{{}} c_{\text{p}} = m \cdot \sin \beta } \\ {45\;{\text{deg}} \le \beta < 135\;{\text{deg}} :a_{\text{p}} = m \cdot \left| {\cos \;\beta } \right|;c_{\text{p}} = M \cdot \sin \beta } \\ {135\;{\text{deg}} \le \beta < 185\;{\text{deg}} :a_{\text{p}} = M \cdot \left| {\cos \;\beta } \right|;c_{\text{p}} = m \cdot \sin \beta } \\ \end{array} } \right. $$
(19)

3.3 Nanoindentation

Hardness data from indentation tests can be used to estimate dislocation densities. In the present study, the choice of nanoindentation was imposed by the small dimensions of the iron powder particles, and the consequently restricted inter-particle contact areas, where the plastic deformation is assumed to be concentrated.

For nanoindentation analyses, the surface preparation is a critical step to get consistent experimental values. In order to obtain an optimal surface quality, the metallographic samples were polished using SiC papers with decreasing roughness, followed by polishing with 3- and 1-μm diamond paste, and finally with an OP-S suspension with 0.04-μm-diameter alumina particles. With this type of polishing procedure, it is possible to obtain results comparable to those typical of electrochemical polishing.[70]

Nanoindentation was performed on samples pressureless sintered at 640 °C, 730 °C, 860 °C, and 960 °C. Three different indentation forces (55, 75, and 95 mN) were employed, with a 20-second hold before unloading. The measurements were carried out in the contact areas aligned along the x and y directions, and not in ambiguously oriented ones, in order to observe the state of deformation in these two distinct regions of the porous material. Figure 6 shows an example of micrographs of nanoindentation for each direction.

Fig. 6
figure 6

Examples of micrographs of nanoindentation tests: (a) with 55 mN load on a sample sintered at 640 °C (for a longitudinal contact); (b) with a 95 mN load on a sample sintered at 960 °C (for a transverse contact). In the latter case, the two involved powder particles have been highlighted

Full size image

3.4 Electron Backscatter Diffraction (EBSD)

EBSD is a technique performed in the SEM that allows direct measurement of the lattice orientation in crystalline materials. Lattice orientation can, in turn, be related to dislocation density. Here the experimental procedure is described, and in Section IV–D the dislocation densities calculation is presented.

The iron samples sintered at 640 °C, 730 °C, 860 °C, and 1010 °C were mounted in hot resin. Grinding was performed at 300-rpm under a force of 20N for 5 minutes through 220, 600, and 1200 grit. Polishing was performed at 150-rpm under a force of 15 N for 5 minutes using 3-µm diamond suspension, 10 minutes using 1-µm diamond suspension, and 10 N force for 20 minutes using 0.04-µm colloidal silica solution. A finishing polish step was then applied for 60 minutes using the colloidal silica activated disk and deionized water under a force of 5 N.

Prior to EBSD imaging in the SEM, we carried out a step size analysis and determined an appropriate step size to be ~ 200 nm for the EBSD scans reported herein. Furthermore, in order to present valid GND results, the lower bound of log10 GND density is estimated, i.e., noise floor at a step size of 200 nm, to be 9.93 × 1013 per m2, and this value is used as a lower bound filter for GND post-processing. A misorientation threshold is set to 2 deg to filter out GND calculated from minimum misorientation of non-indexed points or neighboring grains. The misorientation mask also produced clear-cut grain boundaries and an average disorientation (i.e., minimum misorientation) angle map that can be readily visualized, as shown in Figure 7.

Fig. 7
figure 7

Example of EBSD results for GND density distribution (measured as log10 of GNDs per m2) and average disorientation angle distribution: (a) GND density distribution for a sample sintered at 1010 °C, acquired at 2000 times and with 200 nm step size, with selection of boxes around representative x and y inter-particle contacts; (b) GND density along y and (d) along x; (c) average disorientation angle distribution around an inter-particle boundary along y and (e) along x

Full size image

The GND analysis of inter-particle boundaries can be summarized to be a four-step procedure, presented schematically in Figure 8.

Fig. 8
figure 8

Steps followed for the EBSD analysis of a sample sintered at 1010 °C: (a) pattern quality image showing the individuation of longitudinal and transverse particle boundaries based on pores at triple junctions; (b) EBSD scan of the pre-selected regions; (c) GND density map around the particle boundaries; (d) particle boundary selection refinement with an 8 × 4 µm box

Full size image

The EBSD imaging was conducted on a FEI Quanta 600 SEM, equipped with a Bruker e-Flash EBSD system, operated at 20 kV, with a 1 × 1 binning size and working distance of ~ 15 mm. The inter-particle boundaries were pre-selected on the forward-scattered electron image collected by a detector roughly 25 mm from the sample surface. Typically, as demonstrated in Figure 8, particle contacts could be readily identified near two pointed-pores (namely particle boundary triple-points). Then, the detector distance was reduced to ~ 15 mm and masked areas were placed on the selected particle boundaries for EBSD scans. Three scans per sample were taken at a step size of 200 nm at different locations in the proximities of the center of the sample. Approximately 15 horizontal and 15 vertical particles contacts were considered for every sample (30 for every sample, 120 in total). To minimize the EBSD scan time, instead of analyzing the entire sample, EBSD scans were performed only on selected area in the masked zones over the contact boundaries. In each case of particle boundaries selection, the masking boxes were set to have the short side (across the particle contact) equal to 4 µm, and the long side (along the contact) equal to 8 µm, as shown in Figure 8. The selection of the short side size was the most critical, because it implied an assumption on the extent of the plastically deformed zone. Qualitative considerations based on the previously conducted nanoindentation analyses were combined to the image analyses results, until 2cp (≈ 4 μm), with cp being the smallest geometric parameter involved in this study. Centering of the boxes on the particle boundaries was performed manually from the EBSD images to accurately locate the particle boundary in the EBSD scan.

4 Results and Discussion

4.1 Dilatometric Analysis

From the dilatometric curves, the shrinkage rates in the transverse and longitudinal directions, \( \dot{\varepsilon }_{x}^{f.s.} \) and \( \dot{\varepsilon }_{y}^{f.s.} \), could be calculated. Figure 9 shows that the absolute values of shrinkage rates progressively decrease during the isothermal holding time-step.

Fig. 9
figure 9

Example of derivation of isothermal shrinkage rate in the transverse direction, \( \dot{\varepsilon }_{x} (t) \), for a sample sintered at 960 °C. (a) Dilatometric curve with isothermal part highlighted between dashed lines; (b) corresponding isothermal shrinkage rate

Full size image

An integral average was therefore calculated as

$$ \left\{ {\begin{array}{*{20}l} {\bar{\dot{\varepsilon }}_{x}^{f.s} = {\raise0.7ex\hbox{${\int_{0}^{t} {\dot{\varepsilon }_{x}^{f.s} \left( t \right) \cdot {\text{d}}t} }$} \!\mathord{\left/ {\vphantom {{\int_{0}^{t} {\dot{\varepsilon }_{x}^{f.s} \left( t \right) \cdot {\text{d}}t} } {t^{*} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${t^{*} }$}}} \\ {\bar{\dot{\varepsilon }}_{y}^{f.s} = {\raise0.7ex\hbox{${\int_{0}^{t} {\dot{\varepsilon }_{y}^{f.s} \left( t \right) \cdot {\text{d}}t} }$} \!\mathord{\left/ {\vphantom {{\int_{0}^{t} {\dot{\varepsilon }_{y}^{f.s} \left( t \right) \cdot {\text{d}}t} } {t^{*} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${t^{*} }$}}} \\ \end{array} } \right. $$
(20)

where t* (s) is the time at which \( \dot{\varepsilon }_{x}^{f.s} \left( t \right) \) and \( \dot{\varepsilon }_{y}^{f.s} \left( t \right) \) approach zero.

The obtained values for the various sintering temperatures are given in Table I.

Table I Shrinkage Rates Along x (Transverse) and y (Longitudinal) Directions at the Different Sintering Temperatures
Full size table

As expected, the shrinkage rates are higher along the compaction direction (in absolute value) than along the transverse one. Below the Curie point (T = 770 °C), the difference between the two directions is most noticeable and shows a significant increase with increasing temperatures. Such a difference is still evident between the Curie point and the temperature of the austenitic transformation (T = 910 °C), while it becomes almost negligible in the austenitic field, probably due to recrystallization and recovery phenomena. Increasing sintering temperatures also lead to noticeable changes in the value of the average shrinkage rates. Between 640 °C and 860 °C, the expected increase in absolute densification rate is observed when the isothermal temperature increases. The increase is particularly sharp between 640 °C and 730 °C, due to the magnetic transformation, which greatly increases the self-diffusivity of iron starting around 680 °C.[66] The shrinkage rates subsequently drop when 960 °C is reached, due to the austenitic transformation. Switching from a BCC to an FCC phase acts as an annihilation of plastic deformation, and thus the pipe-diffusion effect is reduced. Raising the temperature to 1010 °C then provides additional energy to the process, and therefore enhances densification again. Since in Table I only the densification rate during isothermal hold is considered, another reason for the observed changes in the absolute densification rate is that the starting density at the onset of isothermal sintering is different in all these samples (increasing as the temperature increases). The focus on the isothermal segment allowed the exclusion of thermal expansion effects from the present study. Nevertheless, the partial shrinkage occurring during the heating ramp led to the varying densities at the onset of the isothermal hold, depending on the selected processing temperatures. The concurrent effects of shrinkage and thermal expansion upon heating is the object of an extension of the present study. The cooling stage was also analyzed, and it was assessed that only the expected thermal contraction occurred, with no traces of additional shrinkage and anisotropic behavior in the samples.

4.2 Image Analysis

Figures 10 and 11 present the estimations of inter-particle contacts and pores semi-axes lengths, respectively.

Fig. 10
figure 10

Inter-particle contact lengths a and c at the various sintering temperatures

Full size image
Fig. 11
figure 11

Pores semi-axes lengths, ap and cp, at the different temperatures (a) values obtained from image analyses; (b) average values \( \bar{a}_{\text{p}} \) and \( \bar{c}_{\text{p}} \)

Full size image

In all the considered cases, the semi-axes perpendicular to the compaction direction are greater than those parallel to it, confirming the anisotropy of the porous material due to the pre-sintering cold pressing. This is more evident for the inter-particle contacts than in the pores semi-axes case. For clarity, Figure 11 also shows the average of the measured values, \( \bar{a}_{\text{p}} \) and \( \bar{c}_{\text{p}} \). It can be seen that the extension of the pores in the compaction direction is lower than in the transverse plane at every sintering temperature, but the distinction is less prominent at higher sintering temperatures, in accordance with dilatometry results. The pore semi-axes data have been used to calculate the curvature of the pores through Eq. [1], leading to values in the range of 2.1 to 2.8 μm, depending on the direction and temperature.

4.3 Nanoindentation

The first set of dislocation density data needed to verify the reliability of our model was derived from the nanoindentation results, through the indentation size effect (ISE) model developed by Nix and Gao.[60,61,71] This method uses Taylor’s non-local theory of plasticity,[72] according to which, for indentation tests at the micro and nano-scales, the depth of the indent and the measured hardness values show a strong correlation for depths less than 1-μm. In crystalline materials, this correlation is influenced by a characteristic material parameter, called material length scale, which depends on the deformation state of the sample under examination.[73,74]

The nanoindentation hardness values and the corresponding indentation depths, reported in Table II, served as input for the main ISE equation, namely

$$ \frac{H}{{H_{0} }} = \sqrt {1 + {\raise0.7ex\hbox{${h^{*} }$} \!\mathord{\left/ {\vphantom {{h^{*} } h}}\right.\kern-0pt} \!\lower0.7ex\hbox{$h$}}} $$
(21)

where H (GPa) is the experimental value of the hardness; H0 (GPa) is the hardness due to pre-existing dislocations, namely not due to the indentation process itself, h (μm) is the depth of the indentation, and h* (μm) is the characteristic depth of the material above which we do not have any correlation between indentation depth and nanoindentation hardness values. The highest and lowest measured H values are discarded, in order to utilize more reliable experimental data.

Table II Nanoindentation Hardness (H) and Indentation Depth (h) Values Measured by Nanoindentation at the Inter-Particle Contact Areas
Full size table

Equation [21] can be rearranged as

$$ H^{2} = H_{0}^{2} \cdot \left( {1 + {\raise0.7ex\hbox{${h^{*} }$} \!\mathord{\left/ {\vphantom {{h^{*} } h}}\right.\kern-0pt} \!\lower0.7ex\hbox{$h$}}} \right) $$
(22)

such that the plot of H2vs 1/h will consist of a straight line with slope proportional to h* and intercept corresponding to H 20 .

The dislocation density can subsequently be estimated with the following relation[73,74]:

$$ d_{d} = 3 \cdot \bar{r} \cdot {\raise0.7ex\hbox{${\tan^{2} \theta }$} \!\mathord{\left/ {\vphantom {{\tan^{2} \theta } {\left( {2 \cdot b \cdot h^{*} } \right)}}}\right.\kern-0pt} \!\lower0.7ex\hbox{${\left( {2 \cdot b \cdot h^{*} } \right)}$}} $$
(23)

where \( \bar{r} \) is the Nye factor (set as 1 due to negligible macroscopic plastic strain gradient produced during cold compaction of powder); ϴ is the angle of contact between the indenter tip and the sample surface (24.73 deg for Berkovich tips, utilized in the present case) and b is the Burger’s vector modulus (2.49 Å).

The results obtained for the different temperatures and directions are presented in Table III and Figure 12.

Table III Dislocation Densities for Transverse Contacts (ddx) and for Longitudinal Contacts (ddy)
Full size table
Fig. 12
figure 12

Dislocation density values: (a) comparison between experimental and modeling results; (b) ratio between experimental and modeling results

Full size image

The expected higher amount of plastic deformation in the plane perpendicular to the cold compaction direction is confirmed: the dislocation densities are higher in the transverse contacts (ddx, responsible for the shrinkage along the y direction) than in the longitudinal ones (ddy, responsible for the shrinkage along x). With increasing sintering temperatures, the dislocation densities tend to increase until the Curie point is reached, because of the local deformations induced by the rising sintering stresses at the necks between the ductile iron powder particles, as reported also by Schatt and Friedrich.[14] After the Curie point, dislocation densities steadily decrease, likely because of the annealing and consequent recrystallization and recovery of the deformed material, leading to a less marked anisotropy. These observations further confirmed the dilatometry and image analysis observations.

4.4 EBSD

The second set of dislocation density data was obtained from the EBSD analysis.[64] With this technique, only geometrically necessary dislocations (GNDs) are detected, and not statistically stored dislocations (SSDs). Nevertheless, it is expected valuable information can be obtained, since the high ductility of iron and the elevated load applied during cold compaction generally lead to the generation of significant amounts of GNDs, compared to the pre-existing SSDs.

The theory for the determination of GND density from EBSD data was adopted from the work of Nye, relating lattice curvature to the dislocation density tensor, often itself denoted as the Nye tensor.[75] Taking into account the elastic strain (ɛ el ij ), according to Kröner, the Nye/Kröner relation can be written as[76]

$$ \alpha_{ik} = k_{ki} - \delta_{ki} k_{mm} - \epsilon_{klj} \frac{{\partial \varepsilon_{ij}^{\text{el}} }}{{\partial x_{l} }} $$
(24)

where kij is the lattice curvature tensor and αij is the Nye tensor. In cases where the plastic distortion of the lattice is assumed to be fully accommodated by lattice rotation, i.e., negligible elastic strain, the dislocation density tensor can be approximated as the curl of the lattice misorientation matrix (Δϕ = gBg −1 A ) in the following way[77]:

$$ \alpha_{ik} \approx - \epsilon_{kli}\Delta \phi_{ij,l} $$
(25)

The lattice orientation tensor (g) is directly measurable by the electron backscatter diffraction (EBSD) technique in the SEM. For 2-D EBSD analysis, the orientation gradient in the out of plane direction is assumed to be zero, such that the GND density calculated here is a lower bound solution of the actual GND density. Techniques such as serial-sectioning[78] and X-ray microbeams[79] have been developed to extract lattice orientation in the out of plane direction, but these techniques are usually complex and time-consuming.

On the other hand, the total GND density tensor can also be represented as the sum of individual dislocations, where each type of dislocation is geometrically characterized by a combination of the unit line vector \( \hat{l} \) and its Burger’s vector b:

$$ \alpha_{ij} = \sum\nolimits_{n = 1}^{N = 16} {\rho_{\text{GND}}^{n} \cdot b_{i}^{n} \hat{l}_{j}^{n} } $$
(26)

The dislocation configurations available in a bcc crystal are 4 screw 〈111〉 dislocations and 12 edge 〈111〉 {110} dislocations. This allows the number of dislocations present in the lattice to accommodate the measured lattice curvature to be calculated. The solution to this underdetermined system of equations, with 16 unknowns and 9 equations, is obtained through a so-called ‘interior-point’ method based on an L1 dislocation energy minimization scheme.[80] In practice, the 32 unknowns of dislocations densities (both positive and negative dislocations) are calculated, and the solution folded back to give the 16-component dislocation density vector.

Lattice misorientation data were obtained in images of the type presented in Figure 8. Image post-processing, as well as GND calculations, were then conducted with the Optimization Toolbox of the MathWorks™ Matlab Program. The mean value of the GND density at the particle boundaries was obtained by dividing the sum of the total GND density for all data points near the particle boundary by number of data points with non-zero GND density. This approach allows the dislocation gradient from the particle interior to the boundary to be calculated. To rule out large statistical variations, we have ignored the maximum and minimum obtained values of mean GND density. Average dislocation density values in the x and y directions and for each sintering temperature were then computed. The obtained results are displayed in Figure 12. A good correlation with the dislocation density from the nanoindentation hardness data is observed, with EBSD-estimated dislocation density values just below the nanoindentation hardness values. This is in accordance with the fact that EBSD does not detect statistically stored dislocations. In addition, pipe diffusion is mainly through SSDs at low sintering temperatures and through GNDs at high sintering temperatures. This is due to the higher mobility of SSDs (which create a less severe atomic lattice distortion with respect to GNDs), while diffusion through GNDs requires more elevated temperatures in order to have a significant impact on densification. The total dislocation density approached the GND’s density at increasing temperatures, which implies thermal annihilation of SSDs. At such temperatures, the mobility of GND’s is also high enough for them to contribute to the material’s sintering, while the SSD’s role becomes decreasingly important. It is interesting to point out that, different from nanoindentation results, all EBSD-derived dislocation density values are close to the 1015 value, because GNDs are less sensitive to changes in sintering temperature. This appears to be a maximum for most EBSD analyses, and is in reasonable agreement with the fact that inter-particle boundaries can only allow a limited amount of local lattice misorientation.

4.5 Model Validation

The micromechanical model can now be validated by comparing the experimentally obtained dislocation density data with those calculated from the model using Eq. [17]. Experimental data on shrinkage rates (Section IV–A) and geometric parameters (Section IV–B) along x and y were obtained previously. The other input data needed are iron material properties taken from the literature. Specifically, the inputs are

  • γsv, surface tension: 2.525 N/m[81,82];

  • γss, grain boundary energy: 0.985 N/m[83];

  • δ, inter-particle boundary thickness, estimated as grain boundary thickness: 0.5 nm[84];

  • rp, pipe radius: 0.5 nm[66];

  • Ω, atomic volume: 8.38 × 10−30 m3.[85]

Additionally, the values of the volume diffusion coefficient Dv as a function of the sintering temperature were taken from Reference 86 and are given in Table IV. In Table IV, the dislocation pipe diffusion coefficient Dp values, obtained from Stechauner and Kozeschnik,[87] based on an Arrhenius-type relation, Dp = D0 exp(− Q/RT), with activation energy Q (kJ/mol) and pre-exponential factor D0 (m2/s) changing for the different iron phases, are reported. The calculated pipe diffusivity values are comparable with the experimental results measured by Shima et al.[66] at all temperatures, with the only exception of the austenitic field, for which, to the best of our knowledge, data are not available in the literature.

Table IV Volume and Pipe Diffusivities at Equilibrium
Full size table

Note that, even though the model here is assessed with data on the free pressureless sintering of pure iron, its applicability can be extended to a variety of material systems, provided the data listed above. The utilization of the continuum theory of sintering also allows for the possibility of including the role of externally applied pressures, by adding the opportune term to the equations set. Thanks to its structure, it is also possible to compare or connect with other sintering models present in literature, for instance, with the meso-scale discrete element-based simulations.[88]

Substituting all the data listed above in Eq. [17], we could calculate estimations of dislocation densities in the transverse (x) and longitudinal (y) directions for the various sintering temperatures. Figure 12 shows the comparison between the calculated and experimentally obtained dislocation densities at different temperatures. A good agreement is observed, with the only exception of T = 860 °C, at which nanoindentation and EBSD results are fairly close to each other, while the model seems to underestimate the amount of plastic deformation. This particular temperature, however, belongs to a range between Curie and austenitic point in which the literature data for dislocation pipe diffusivity are widely scattered and most likely often overestimated. Such uncertainty might be responsible for the mismatch between modeling and experimental framework at this particular temperature.

In order to verify this hypothesis, a parametric study was performed, by assessing the effect of a change in the different variables involved in Eq. [17] on the calculated dislocation density values. This analysis allows analyzing the sensitivity of the model to the various parameters involved, by observing the changes in its response when the parameters are altered within a reasonable range. The parameters taken into consideration for this part of the study were pipe diffusivity, bulk diffusivity, strain rates, particle semi-contact lengths and pore semi-axes. They were all changed within a certain range, selected as follows. For the parameters that had been measured experimentally (shrinkage rates, particle semi-contact lengths and pore semi-axes), the standard deviation was taken as a reference for the variation of each parameter. Specifically, the value of each parameter was altered by adding or subtracting 50 and 100 pct of their standard deviation value. For the parameters that had been taken from the literature (pipe and bulk diffusivities), the data were changed by taking 10, 50, and 80 pct of the reported values. Since the model was giving an underestimation of expected dislocation densities, each study parameter was altered in such a way as to lead to their increase.

Figures 13 and 14 confirm that pipe diffusivity is the parameter with the highest influence on the model.

Fig. 13
figure 13

Parametric study outcomes for varying pipe diffusion coefficient

Full size image
Fig. 14
figure 14

Parametric study outcomes for varying (a) bulk diffusion coefficient; (b) strain rates; (c) particle semi-axes; (d) pore semi-axes

Full size image

In all Figures, the y-axis represents the ratio between dislocation density calculated with the modified parameter value (abbreviated as “mod”) and the one calculated with the original value (“nom” as for nominal for data from the literature and “avrg” as for average for experimental data). The legend specifies if we are referring to the x (transverse) or y (longitudinal) direction. It also provides the entity of the change in the considered parameter, namely the percentage of nominal value for the diffusivities and the addition or subtraction of the standard deviation (“stdv”, 50 or 100 pct) for strain rates and geometric features. The pipe diffusivity case is shown in Figure 13, while Figure 14 is for all other parameters.

A decrease in pipe diffusivity up to 10 pct of the value reported in the literature leads to an increase in dislocation densities that reaches one order of magnitude. None of the other parameters has such a significant effect, with a maximum factor of 3.5 reached in the particle semi-axes study (noticeably, still at 860 °C). Note how a variation in the value of pipe diffusivity causes a change in calculated dislocation densities that is consistent for all the temperatures considered (Figure 13), while the other parameters (Figure 14) present a more unstable trend. The most striking case is the one relative to bulk diffusivity (part (a) of Figure 14), where, again at 860 °C, we can observe a peak in the dislocation densities ratio, in contrast with the other temperatures. This can be explained by looking at the bulk diffusivity values and recalling how the parametric study procedure was set up. For data extracted from the literature (diffusivities), we anticipated that various percentages of the reported values were used. Since the bulk diffusivity reaches a maximum at 860 °C, and since its value at this temperature is orders of magnitude higher than at the other temperatures considered in this study, its variation leads to a more significant oscillation in the calculated dislocation densities. Such a trend is not as evident when considering parameters that were measured experimentally, because here the standard deviation was used as parameter variation range. It is, nevertheless, worth pointing out that the 860 °C samples were shown to be the most sensitive to the parametric variations in the majority of cases. In part (b) of Figure 14, only for 860 °C it is possible to discern all the plotted data points, while for the other temperatures they overlap.

A final verification of the model’s sensitivity to the involved parameters was performed by assessing the influence of the pores’ curvature radii on the pipe diffusion coefficient. This translates into checking the suitability of the method used to estimate the pores curvature (shape approximated as ellipsoid) and verifying its effects on the mass transfer mechanism. Our analysis shows that even much smaller values of pore radii (reduced of up to more than one order of magnitude with respect to the initially measured ones) do not alter significantly the pipe diffusivity values. This confirms the applicability of the ellipsoidal shape-based approximation. An even higher accuracy can be achieved in the prosecution of this work by evaluating the curvature of the pores via high resolution SEM visualization.

The uncertainty in pipe diffusivity data is thus confirmed to be the most likely cause of the inaccuracy of the model at 860 °C. In light of this finding, the literature data that had been previously utilized were re-examined. In the work of Stechauner and Kozeschnik,[87] there are no experimental data available for temperatures above about 650 °C. They propose an extension of the curve until above 1400 °C, most likely in analogy with the grain boundary diffusivity data provided in the same plot, on which the data given in Table IV are based. However, it is reasonable to assume that the slope of the curve for dislocation pipe diffusivity as a function of temperature should decrease with increasing temperatures, and not vice versa, as higher temperatures lead to a progressive annihilation of the dislocations present in a material.

By back-calculating the values of pipe diffusivity based on our experimental data, a modification to the Stechauner and Kozeschnik’s fitting is proposed in Figure 15.

Fig. 15
figure 15

Adapted from Ref. [87]

Proposed modification of the dislocation pipe diffusivity fitting reported by Stechauner and Kozeschnik. (a) α-Fe, (b) γ-Fe.

Full size image

The dislocation densities used for these back-calculations were the ones derived from the nanoindentation measurements, since the overall amount is needed, including both GNDs and SSDs. Figure 15 is a reproduction of Figure 2 from the original paper,[87] with the addition of the back-calculation outcomes (in bright blue). Specifically, the pipe diffusivities resulted to be 6.01 × 10−14 m2/s for 640 °C, 1.53 × 10−13 m2/s for 730 °C, 3.20 × 10−13 m2/s for 860 °C, and 2.14 × 10−12 m2/s for 960 °C. Note that the pipe diffusivity at 640 °C is in very good agreement with the experimental data provided in the original paper, and that the extrapolated curve approaches the bulk diffusivity one with increasing temperatures, as expected with the progressive annealing of a cold-worked material. Note also that, at the current state of this work, the distinction between edge and screw dislocations has not been introduced. These two kinds of dislocations generally lead to different values of pipe diffusivity. Such an investigation can be the object of future work.

The generally good agreement between the model and the experiments, together with the subsequent parametric study, confirms the reliability and applicability of our micromechanical model for the study of shrinkage anisotropy during sintering of cold-pressed metallic powders.

5 Conclusions

The anisotropy of shrinkage during sintering of iron powders pre-compacted with a uniaxial load is investigated. A micromechanical model is proposed for the shrinkage rates along and perpendicular to the uniaxial load direction. Due to plastic deformation introduced by the pre-sintering cold compaction, volume diffusion enhanced by dislocation pipe diffusion is considered to be the dominant mass transfer mechanism during post-cold-compaction sintering. The model parameters include the geometric characteristics of the deformed porous structure, the structural defects (dislocations), diffusivities and sintering temperatures. Dilatometric tests provide data on the shrinkage rates, and image analysis is employed to quantify the characteristic dimensions of inter-particle contacts and pores. Plugging these experimental results into this model, dislocation densities are calculated at the inter-particle contacts in the longitudinal and transverse directions. The applicability of the model is verified by comparing these calculated values with dislocation densities estimated by means of nanoindentation and EBSD techniques. Good agreement is found between experiments and our micromechanical model, with the only exception of the temperature range between Curie and austenitic points, in which the experimental value of dislocation pipe diffusion coefficient is highly scattered. A parametric study confirms that pipe diffusivity is the parameter that has the strongest influence on the model results, and offers the possibility to back-calculate these pipe diffusivity values. As expected, higher dislocation densities are found along the contacts perpendicular to the cold pressing direction, suggesting that the pre-sintering cold compaction is the main cause for shrinkage anisotropy during sintering.