Skip to main content
SpringerLink
Log in

An Evolutionary Reduction Principle for Mutation Rates at Multiple Loci

  • Original Article
  • Published:
Bulletin of Mathematical Biology Aims and scope Submit manuscript

Abstract

A model of mutation rate evolution for multiple loci under arbitrary selection is analyzed. Results are obtained using techniques from Karlin (Evolutionary Biology, vol. 14, pp. 61–204, 1982) that overcome the weak selection constraints needed for tractability in prior studies of multilocus event models.

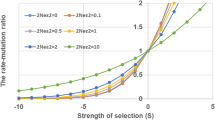
A multivariate form of the reduction principle is found: reduction results at individual loci combine topologically to produce a surface of mutation rate alterations that are neutral for a new modifier allele. New mutation rates survive if and only if they fall below this surface—a generalization of the hyperplane found by Zhivotovsky et al. (Proc. Natl. Acad. Sci. USA 91, 1079–1083, 1994) for a multilocus recombination modifier. Increases in mutation rates at some loci may evolve if compensated for by decreases at other loci. The strength of selection on the modifier scales in proportion to the number of germline cell divisions, and increases with the number of loci affected. Loci that do not make a difference to marginal fitnesses at equilibrium are not subject to the reduction principle, and under fine tuning of mutation rates would be expected to have higher mutation rates than loci in mutation-selection balance.

Other results include the nonexistence of ‘viability analogous, Hardy–Weinberg’ modifier polymorphisms under multiplicative mutation, and the sufficiency of average transmission rates to encapsulate the effect of modifier polymorphisms on the transmission of loci under selection. A conjecture is offered regarding situations, like recombination in the presence of mutation, that exhibit departures from the reduction principle. Constraints for tractability are: tight linkage of all loci, initial fixation at the modifier locus, and mutation distributions comprising transition probabilities of reversible Markov chains.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Ababneh, F., Jermiin, L. S., & Robinson, J. (2006). Generation of the exact distribution and simulation of matched nucleotide sequences on a phylogenetic tree. J. Math. Model. Algorithms, 5, 291–308.

    Article  MathSciNet  MATH  Google Scholar 

  • Altenberg, L. (1984). A generalization of theory on the evolution of modifier genes. Ph.D. thesis, Stanford University. Searchable online and available from University Microfilms, Ann Arbor, MI.

  • Altenberg, L. (2009). The evolutionary reduction principle for linear variation in genetic transmission. Bull. Math. Biol., 71, 1264–1284.

    Article  MathSciNet  MATH  Google Scholar 

  • Altenberg, L., & Feldman, M. W. (1987). Selection, generalized transmission, and the evolution of modifier genes. I. The reduction principle. Genetics, 117, 559–572.

    Google Scholar 

  • Baer, C. F., Miyamoto, M. M., & Denver, D. R. (2007). Mutation rate variation in multicellular eukaryotes: causes and consequences. Nat. Rev. Genet., 8, 619–631.

    Article  Google Scholar 

  • Balkau, B., & Feldman, M. W. (1973). Selection for migration modification. Genetics, 74, 171–174.

    MathSciNet  Google Scholar 

  • Brandon, R. N. (1982). The levels of selection. In P. Asquith & T. Nickles (Eds.), PSA 1982 (Vol. 1, pp. 315–323). East Lansing: Philosophy of Science Association.

    Google Scholar 

  • Charlesworth, B. (1990). Mutation-selection balance and the evolutionary advantage of sex and recombination. Genet. Res., 55, 199–221.

    Article  Google Scholar 

  • Charlesworth, B., & Charlesworth, D. (1979). Selection on recombination in clines. Genetics, 91, 581–589.

    MathSciNet  Google Scholar 

  • Charlesworth, B., Charlesworth, D., & Strobeck, C. (1979). Selection for recombination in partially self-fertilizing populations. Genetics, 93, 237–244.

    MathSciNet  Google Scholar 

  • Deutsch, E., & Neumann, M. (1984). Derivatives of the Perron root at an essentially nonnegative matrix and the group inverse of an M-matrix. J. Math. Anal. Appl., 102, 1–29.

    Article  MathSciNet  MATH  Google Scholar 

  • Duistermaat, J. J., & Kolk, J. A. C. (2004). Cambridge studies in advanced mathematics: Vol. 86. Multidimensional real analysis I: Differentiation. Cambridge: Cambridge University Press. ISBN 9780521551144.

    Book  MATH  Google Scholar 

  • Eyre-Walker, A., & Keightley, P. D. (2007). The distribution of fitness effects of new mutations. Nat. Rev. Genet., 8, 610–618.

    Article  Google Scholar 

  • Feldman, M. W. (1972). Selection for linkage modification: I. Random mating populations. Theor. Popul. Biol., 3, 324–346.

    Article  Google Scholar 

  • Feldman, M. W., & Balkau, B. (1973). Selection for linkage modification II. A recombination balance for neutral modifiers. Genetics, 74, 713–726.

    MathSciNet  Google Scholar 

  • Feldman, M. W., & Krakauer, J. (1976). Genetic modification and modifier polymorphisms. In S. Karlin & E. Nevo (Eds.), Population genetics and ecology (pp. 547–583). New York: Academic Press.

    Google Scholar 

  • Feldman, M. W., & Liberman, U. (1986). An evolutionary reduction principle for genetic modifiers. Proc. Natl. Acad. Sci. USA, 83, 4824–4827.

    Article  MathSciNet  MATH  Google Scholar 

  • Feldman, M. W., Christiansen, F. B., & Brooks, L. D. (1980). Evolution of recombination in a constant environment. Proc. Natl. Acad. Sci. USA, 77, 4838–4841.

    Article  MathSciNet  Google Scholar 

  • Feller, W. (1971). An introduction to probability theory and its applications, Vol. I (3rd ed.). New York: Wiley.

    MATH  Google Scholar 

  • Fox, A., Tuch, B., & Chuang, J. (2008). Measuring the prevalence of regional mutation rates: an analysis of silent substitutions in mammals, fungi, and insects. BMC Evol. Biol., 8, 186.

    Article  Google Scholar 

  • Giraud, A., Matic, I., Tenaillon, O., Clara, A., Radman, M., Fons, M., & Taddei, F. (2001). Costs and benefits of high mutation rates: adaptive evolution of bacteria in the mouse gut. Science, 291.

  • Guillemin, V., & Pollack, A. (1974). Differential topology. Prentice-Hall: Englewood Cliffs.

    MATH  Google Scholar 

  • Hirsch, M. W. (1976). Differential topology. New York: Springer.

    MATH  Google Scholar 

  • Hoede, C., Denamur, E., & Tenaillon, O. (2006). Selection acts on DNA secondary structures to decrease transcriptional mutagenesis. PLoS Genet., 2, e176. http://dx.plos.org/10.1371%2Fjournal.pgen.0020176.

    Article  Google Scholar 

  • Holsinger, K., Feldman, M. W., & Altenberg, L. (1986). Selection for increased mutation rates with fertility differences between matings. Genetics, 112, 909–922.

    Google Scholar 

  • Holsinger, K. E., & Feldman, M. W. (1983a). Linkage modification with mixed random mating and selfing: a numerical study. Genetics, 103, 323–333.

    Google Scholar 

  • Holsinger, K. E., & Feldman, M. W. (1983b). Modifiers of mutation rate: evolutionary optimum with complete selfing. Proc. Natl. Acad. Sci. USA, 80, 6732–6734.

    Article  MathSciNet  MATH  Google Scholar 

  • Horn, R. A., & Johnson, C. R. (1985). Matrix analysis. Cambridge: Cambridge University Press.

    MATH  Google Scholar 

  • Iosifescu, M. (1980). Finite Markov processes and their applications. Bucharest: Wiley.

    MATH  Google Scholar 

  • Jayaswal, V., Jermiin, L. S., & Robinson, J. (2005). Estimation of phylogeny using a general Markov model. Evol. Bioinform. Online, 1, 62–80.

    Google Scholar 

  • Karlin, S. (1976). Population subdivision and selection migration interaction. In S. Karlin & E. Nevo (Eds.), Population genetics and ecology (pp. 616–657). New York: Academic Press.

    Google Scholar 

  • Karlin, S. (1982). Classification of selection-migration structures and conditions for a protected polymorphism. In M. K. Hecht, B. Wallace, & G. T. Prance (Eds.), Evolutionary biology (Vol. 14, pp. 61–204). New York: Plenum.

    Google Scholar 

  • Karlin, S., & McGregor, J. (1972a). Application of method of small parameters to multi-niche population genetic models. Theor. Popul. Biol., 3, 186–209.

    Article  MathSciNet  Google Scholar 

  • Karlin, S., & McGregor, J. (1972b). The evolutionary development of modifier genes. Proc. Natl. Acad. Sci. USA, 69, 3611–3614.

    Article  Google Scholar 

  • Karlin, S., & McGregor, J. (1974). Towards a theory of the evolution of modifier genes. Theor. Popul. Biol., 5, 59–103.

    Article  MathSciNet  Google Scholar 

  • Keilson, J. (1979). Markov chain models: rarity and exponentiality. New York: Springer.

    MATH  Google Scholar 

  • King, D. G., & Kashi, Y. (2007). Mutation rate variation in eukaryotes: evolutionary implications of site-specific mechanisms. Nat. Rev. Genet., 8.

  • Kingman, J. F. C. (1978). A simple model for the balance between selection and mutation. J. Appl. Probab., 15, 1–12.

    Article  MathSciNet  MATH  Google Scholar 

  • Kingman, J. F. C. (1980). Mathematics of genetic diversity. Philadelphia: Society for Industrial and Applied Mathematics. ISBN 0-89871-166-5.

    Google Scholar 

  • Kondrashov, A. S. (1982). Selection against harmful mutations in large sexual and asexual populations. Genet. Res., 40, 325–332.

    Article  Google Scholar 

  • Kondrashov, A. S. (1984). Deleterious mutations as an evolutionary factor. I. The advantage of recombination. Genet. Res., 44, 199–217.

    Article  Google Scholar 

  • Kondrashov, A. S. (1995). Modifiers of mutation-selection balance: general approach and the evolution of mutation rates. Genet. Res., 66, 53–69.

    Article  Google Scholar 

  • Kondrashov, F. A., & Kondrashov, A. S. (2010). Measurements of spontaneous rates of mutations in the recent past and the near future. Philos. Trans. R. Soc. B, 365, 1169–1176.

    Article  Google Scholar 

  • Lewontin, R. C. (1974). The genetic basis of evolutionary change. New York: Columbia University Press.

    Google Scholar 

  • Liberman, U., & Feldman, M. W. (1986a). A general reduction principle for genetic modifiers of recombination. Theor. Popul. Biol., 30, 341–371.

    Article  MathSciNet  MATH  Google Scholar 

  • Liberman, U., & Feldman, M. W. (1986b). Modifiers of mutation rate: A general reduction principle. Theor. Popul. Biol., 30, 125–142.

    Article  MathSciNet  MATH  Google Scholar 

  • Lynch, M. (2010). Rate molecular spectrum, and consequences of human mutation. Proc. Natl. Acad. Sci. USA, 107, 961–968.

    Article  Google Scholar 

  • Lynch, M., Sung, W., Morris, K., Coffey, N., Landry, C. R., Dopman, E. B., Dickinson, W. J., Okamoto, K., Kulkarni, S., Hartl, D. L., & Thomas, W. K. (2008). A genome-wide view of the spectrum of spontaneous mutations in yeast. Proc. Natl. Acad. Sci., 105, 9272–9277.

    Article  Google Scholar 

  • Munkres, J. R. (1975). Topology: a first course. Prentice-Hall: Englewood Cliffs. ISBN 0-13-925495-1.

    MATH  Google Scholar 

  • Otto, S. P., & Feldman, M. W. (1997). Deleterious mutations, variable epistatic interactions, and the evolution of recombination. Theor. Popul. Biol., 51, 34–47.

    Article  Google Scholar 

  • Pylkov, K. V., Zhivotovsky, L. A., & Feldman, M. W. (1998). Migration versus mutation in the evolution of recombination under multilocus selection. Genet. Res., 71, 247–256.

    Article  Google Scholar 

  • Roach, J. C., Glusman, G., Smit, A. F. A., Huff, C. D., Hubley, R., Shannon, P. T., Rowen, L., Pant, K. P., Goodman, N., Bamshad, M., Shendure, J., Drmanac, R., Jorde, L. B., Hood, L., & Galas, D. J. (2010). Analysis of genetic inheritance in a family quartet by whole-genome sequencing. Science. http://dx.doi.org/10.1126/science.1186802.

  • Rodríguez, F., Oliver, J., Marín, A., & Medina, J. (1990). The general stochastic model of nucleotide substitution. J. Theor. Biol., 142, 485–501.

    Article  Google Scholar 

  • Salmon, W. C. (1971). Statistical explanation and statistical relevance. Pittsburgh: University of Pittsburgh Press.

    MATH  Google Scholar 

  • Salmon, W. C. (1984). Scientific explanation and the causal structure of the world. Princeton: Princeton University Press.

    Google Scholar 

  • Singer, I. M., & Thorpe, J. A. (1967). Lecture notes on elementary topology and geometry. New York: Springer. ISBN 0-387-90202-3.

    MATH  Google Scholar 

  • Squartini, F., & Arndt, P. F. (2008). Quantifying the stationarity and time reversibility of the nucleotide substitution process. Mol. Biol. Evol., 25, 2525–2535.

    Article  Google Scholar 

  • Teague, R. (1977). A model of migration modification. Theor. Popul. Biol., 12, 86–94.

    Article  Google Scholar 

  • Whelan, S., & Goldman, N. (2004). Estimating the frequency of events that cause multiple-nucleotide changes. Genetics, 167, 2027–2043.

    Article  Google Scholar 

  • Wilkinson, J. H. (1965). The algebraic eigenvalue problem. Oxford: Clarendon Press.

    MATH  Google Scholar 

  • Yang, Z. (1995). On the general reversible Markov process model of nucleotide substitution: a reply to Saccone et al. J. Mol. Evol., 41, 254–255.

    Google Scholar 

  • Yang, Z., & Nielsen, R. (2002). Codon-substitution models for detecting molecular adaptation at individual sites along specific lineages. Mol. Biol. Evol., 19, 908–917.

    Google Scholar 

  • Zhivotovsky, L. A., & Feldman, M. W. (1995). The reduction principle for recombination under density-dependent selection. Theor. Popul. Biol., 47, 244–256.

    Article  Google Scholar 

  • Zhivotovsky, L. A., Feldman, M. W., & Christiansen, F. B. (1994). Evolution of recombination among multiple selected loci: A generalized reduction principle. Proc. Natl. Acad. Sci. USA, 91, 1079–1083.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. University of Hawai‘i at Manoa, Honolulu, USA

    Lee Altenberg

Authors
  1. Lee Altenberg
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lee Altenberg.

Additional information

Dedicated to the memory of Sam Karlin, whose theorems continue to bear new fruit.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Altenberg, L. An Evolutionary Reduction Principle for Mutation Rates at Multiple Loci. Bull Math Biol 73, 1227–1270 (2011). https://doi.org/10.1007/s11538-010-9557-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11538-010-9557-9

Keywords

Search

Navigation