Skip to main content
SpringerLink
Log in

Anomalous Reactive Transport in the Framework of the Theory of Chromatography

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

Abstract

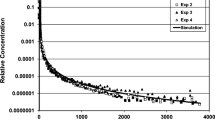
The anomalous reactive transport considered here is the migration of contaminants through strongly sorbing permeable media without significant retardation. It has been observed in the case of heavy metals, organic compounds, and radionuclides, and it has critical implications on the spreading of contaminant plumes and on the design of remediation strategies. Even in the absence of the well-known fast migration pathways, associated with fractures and colloids, anomalous reactive transport arises in numerical simulations of reactive flow. It is due to the presence of highly pH-dependent adsorption and the broadening of the concentration front by hydrodynamic dispersion. This leads to the emergence of an isolated pulse or wave of a contaminant traveling at the average flow velocity ahead of the retarded main contamination front. This wave is considered anomalous because it is not predicted by the classical theory of chromatography, unlike the retardation of the main contamination front. In this study, we use the theory of chromatography to study a simple pH-dependent surface complexation model to derive the mathematical framework for the anomalous transport. We analyze the particular case of strontium (Sr2+) transport and define the conditions under which the anomalous transport arises. We model incompressible one-dimensional (1D) flow through a reactive porous medium for a fluid containing four aqueous species: H+, Sr2+, Na+, and Cl−. The mathematical problem reduces to a strictly hyperbolic 2 × 2 system of conservation laws for effective anions and Sr2+, coupled through a competitive Langmuir isotherm. One characteristic field is linearly degenerate while the other is not genuinely nonlinear due to an inflection point in the pH-dependent isotherm. We present the complete set of analytical solutions to the Riemann problem, consisting of only three combinations of a slow wave comprising either a rarefaction, a shock, or a shock–rarefaction with fast wave comprising only a contact discontinuity. Highly resolved numerical solutions at large Péclet numbers show excellent agreement with the analytic solutions in the hyperbolic limit. In the Riemann problem, the anomalous wave forms only if: hydrodynamic dispersion is present, the slow wave crosses the inflection locus, and the effective anion concentration increases along the fast path.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Ancona F., Marson A.: A note on the Riemann problem for general n × n conservation laws. J. Math. Anal. Appl. 260(1), 279–293 (2001). doi:10.1006/jmaa.2000.6721

    Article  Google Scholar 

  • Appelo, C.: Multicomponent ion exchange and chromatography in natural systems. In: Lichtner P.C., Steefel C. I., Oelkers E. H. (eds.) Reactive Transport in Porous Media, Reviews in Mineralogy, Vol. 34, pp. 193–227. Mineralogical Society of America (1996) Short Course on Reactive Transport in Porous Media, Golden, CO, Oct 25–27, 1996

  • Appelo C., Hendriks J., Van Veldhuizen M.: Flushing factors and a sharp front solution for solute transport with multicomponent ion-exchange. J. Hydrol. 146(1–4), 89–113 (1993). doi:10.1016/0022-1694(93)90271-A

    Article  Google Scholar 

  • Aziz K., Settari T.: Petroleum Reservoir Simulation. Blitzprint Ltd., Calgary (2002)

    Google Scholar 

  • Bankston T.E., Dattolo L., Carta G.: pH Transients in hydroxyapatite chromatography columns—experimental evidence and phenomenological modeling. J. Chromatogr. A 1217(14), 2123–2131 (2010). doi:10.1016/j.chroma.2010.02.004

    Article  Google Scholar 

  • Berkowitz, B., Cortis, A., Dentz, M., Scher, H.: Modeling non-Fickian transport in geological formations as a continuous time random walk. Rev. Geophys. 44(2) (2006). doi:10.1029/2005RG000178

  • Bryant S., Dawson C., van Duijn C.: Dispersion-induced chromatographic waves. Ind. Eng. Chem. Res. 39(8), 2682–2691 (2000). doi:10.1021/ie990796e

    Article  Google Scholar 

  • Cantwell B.: Introduction to Symmetry Analysis. Vol. II. Cambridge University Press, Cambridge (2002)

    Google Scholar 

  • Charbeneau R.: Groundwater contaminant transport with adsorption and ion-exchange chemistry—method of characteristics for the case without dispersion. Water Resour. Res. 17(3), 705–713 (1981). doi:10.1029/WR017i003p00705

    Article  Google Scholar 

  • Charbeneau R.: Multicomponent exchange and subsurface solute transport—characteristics, coherence, and the Riemann problem. Water Resour. Res. 24(1), 57–64 (1988). doi:10.1029/WR024i001p00057

    Article  Google Scholar 

  • Das S., Hendry M.J., Essilfie-Dughan J.: Transformation of two-line ferrihydrite to goethite and hematite as a function of pH and temperature. Environ. Sci. Technol. 45(1), 268–275 (2011). doi:10.1021/es101903y

    Article  Google Scholar 

  • Dattolo L., Keller E.L., Carta G.: pH Transients in hydroxyapatite chromatography columns—effects of operating conditions and media properties. J. Chromatogr. A 1217(48), 7573–7578 (2010). doi:10.1016/j.chroma.2010.10.026

    Article  Google Scholar 

  • Glueckauf, E.: Theory of chromatography; chromatograms of a single solute. J. Chem. Soc. 1302–1308 (1947). doi:10.1039/jr9470001302

  • Greskowiak J., Hay M., Prommer H., Liu C., Pos V., Ma R., Davis J., Zheng C., Zachara J.: Simulating adsorption of U(VI) under transient groundwater flow and hydrochemistry: physical versus chemical nonequilibrium model. Water Resour. Res. 47, 403–409 (2011). doi:10.1029/2010WR010118

    Article  Google Scholar 

  • Haggerty R., Gorelick S.: Multiple-rate mass-transfer for modeling diffusion and surface-reactions in media with pore-scale heterogeneity. Water Resour. Res. 31(10), 2383–2400 (1995). doi:10.1029/95WR10583

    Google Scholar 

  • Helfferich F., Klein G.: Multicomponent Chromatography. Marcel Dekker, New York (1970)

    Google Scholar 

  • Hiemstra T., Van Riemsdijk W.: A surface structural approach to ion adsorption: the charge distribution (CD) model. J. Colloid Interface Sci. 179(2), 488–508 (1996). doi:10.1006/jcis.1996.0242

    Article  Google Scholar 

  • Hiemstra T., Dewit J., Van Riemsdijk W.: Multisite proton adsorption modeling at the solid–solution interface of (hydr)oxides—a new approach. II. Application to various important (hydr)oxides. J. Colloid Interface Sci. 133(1), 105–117 (1989). doi:10.1016/0021-9797(89)90285-3

    Article  Google Scholar 

  • Hiemstra T., Rahnemaie R., Van Riemsdijk W.: Surface complexation of carbonate on goethite: IR spectroscopy, structure and charge distribution. J. Colloid Interface Sci. 278(2), 282–290 (2004). doi:10.1016/j.jcis.2004.06.014

    Article  Google Scholar 

  • Juanes R., Patzek T.: Analytical solution to the Riemann problem of three-phase flow in porous media. Transp. Porous Media 55(1), 47–70 (2004). doi:10.1023/B:TIPM.0000007316.43871.1e

    Article  Google Scholar 

  • Knapp R.: Spatial and temporal scales of local equilibrium in dynamic fluid-rock systems. Geochim. Cosmochim. Acta 53(8), 1955–1964 (1989). doi:10.1016/0016-7037(89)90316-5

    Article  Google Scholar 

  • Lake L., Bryant S., Araque-Martinez A.: Geochemistry and Fluid Flow. 1st edn. Elsevier, Amsterdam (2002)

    Google Scholar 

  • Lax P.: Hyperbolic systems of conservation laws, II. Commun. Pure Appl. Math. 10, 537–566 (1957)

    Article  Google Scholar 

  • LeVeque R.J.: Numerical Methods for Conservation Laws. 2nd edn. Birkhäuser, Berlin (2008)

    Google Scholar 

  • Liu, T.: Riemann problem for general 2 × 2 conservation laws. Trans. Am. Math. Soc. 199, 89–112 (1974)

    Google Scholar 

  • Mazzotti M.: Local equilibrium theory for the binary chromatography of species subject to a generalized Langmuir isotherm. Ind. Eng. Chem. Res. 45(15), 5332–5350 (2006). doi:10.1021/ie060297v

    Article  Google Scholar 

  • Orr F.J.: Theory of Gas Injection Processes. Vol. II. Tie-Line Publications, Holte (2007)

    Google Scholar 

  • Pabst T.M., Carta G.: pH Transitions in cation exchange chromatographic columns containing weak acid groups. J. Chromatogr. A 1142(1), 19–31 (2007). doi:10.1016/j.chroma.2006.08.066. 19th International Symposium on Preparative and Process Chromatography, Baltimore, MD, May 14–17, 2006

  • Pope G., Lake L., Helfferich F.: Cation-exchange in chemical flooding. I. Basic theory without dispersion. Soc. Pet. Eng. J. 18(6), 418–434 (1978)

    Google Scholar 

  • Rhee H., Amundson N.: Study of shock layer in nonequilibrium exchange systems. Chem. Eng. Sci. 27(2), 199 (1972). doi:10.1016/0009-2509(72)85057-7

    Article  Google Scholar 

  • Rhee H., Bodin B.F., Amundson N.: Study of the shock layer in equilibrium exchange systems. Chem. Eng. Sci. 26, 1571–1580 (1971)

    Article  Google Scholar 

  • Rhee H.K., Aris A., Amudson N.: First-Order Partial Differential Equations, Theory and Application of Hyperbolic Systems of Quasilinear Equations. Vol. II. Prentice-Hall, Englewood Cliffs, NJ (1989)

    Google Scholar 

  • Saunders J., Toran L.: Modeling of radionuclide and heavy metal sorption around low- and high-pH waste disposal sites at Oak Ridge, Tennessee. Appl. Geochem. 10(6), 673–684 (1995). doi:10.1016/0883-2927(95)00036-4

    Article  Google Scholar 

  • Scher H., Margolin G., Berkowitz B.: Towards a unified framework for anomalous transport in heterogeneous media. Chem. Phys. 284(1–2, SI), 349–359 (2002). doi:10.1016/S0301-0104(02)00558-X

    Article  Google Scholar 

  • Spalding B., Spalding I.: Chemical equilibria model of strontium-90 adsorption and transport in soil in response to dynamic alkaline conditions. Environ. Sci. Technol. 35(2), 365–373 (2001). doi:10.1021/es001445q

    Article  Google Scholar 

  • Temple B.: Systems of conservation laws with coinciding shock and rarefaction curves. Contemp. Math. 17, 143–151 (1983)

    Article  Google Scholar 

  • Toran L., Bryant S., Saunders J., Wheeler M.: A two-tiered approach to reactive transport: application to Sr mobility under variable pH. Ground Water 36(3), 404–408 (1998). doi:10.1111/j.1745-6584.1998.tb02810.x

    Article  Google Scholar 

  • US EPA. Radiation Protection. Strontium: http://epa.gov/radiation/radionuclides/strontium.html (2011)

  • Valocchi A., Street R., Roberts P.: Transport of ion-exchanging solutes in groundwater—chromatographic theory and field simulation. Water Resour. Res. 17(5), 1517–1527 (1981). doi:10.1029/WR017i005p01517

    Article  Google Scholar 

  • van Beinum W., Hofmann A., Meeussen J., Kretzschmar R.: Sorption kinetics of strontium in porous hydrous ferric oxide aggregates I. The Donnan diffusion model. J. Colloid Interface Sci. 283(1), 18–28 (2005). doi:10.1016/j.jcis.2004.08.067

    Article  Google Scholar 

  • Wieland E., Tits J., Kunz D., Daehn R.: Strontium uptake by cementitious materials. Environ. Sci. Technol. 42(2), 403–409 (2008). doi:10.1021/es071227y

    Article  Google Scholar 

  • Zhu C., Schwartz F.W.: Hydrogeochemical processes and controls on water quality and water management. Elements 7(3), 169–174 (2011). doi:10.2113/gselements.7.3.169

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Petroleum and Geosystems Engineering, University of Texas at Austin, 1 University Station, Austin, TX, 78712, USA

    Valentina Prigiobbe & Steven L. Bryant

  2. Department of Geological Sciences, University of Texas at Austin, 1 University Station, Austin, TX, 78712, USA

    Marc A. Hesse

  3. Institute for Computational Engineering and Sciences, University of Texas at Austin, 1 University Station, Austin, TX, 78712, USA

    Marc A. Hesse & Steven L. Bryant

Authors
  1. Valentina Prigiobbe
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Marc A. Hesse
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Steven L. Bryant
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Valentina Prigiobbe.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Prigiobbe, V., Hesse, M.A. & Bryant, S.L. Anomalous Reactive Transport in the Framework of the Theory of Chromatography. Transp Porous Med 93, 127–145 (2012). https://doi.org/10.1007/s11242-012-9947-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11242-012-9947-6

Keywords

Search

Navigation