Non-smooth dynamics for an efficient simulation of the grand piano action

Abstract

Models with impact or dry friction, yielding discontinuous velocities or accelerations, have motivated research for appropriate numerical methods in the community of non-smooth dynamics. In this work, we apply such methods on the grand piano action. This multibody system has two properties of interest in terms of modelling and simulation: it is extremely sensitive to small misadjustments, and its functioning strongly relies on dry friction and stick–slip transitions—known to be crucial for the touch of the pianist. Using numerical methods of non-smooth contact dynamics, the non-smooth character of dry friction was conserved, in contrast to classical approaches based on regularization which additionally impose the somewhat arbitrary choice of a regularizing parameter. The use of such numerical method resulted in computations about a few hundred times faster than those reported in recent literature. For the first time, the presented predictions of the piano action’s simulations are forces (in particular, the reaction force of the key on the pianist’s finger), instead of displacements which filter out most of the dynamical subtleties of the mechanism. The comparisons between measured and simulated forces in response to a given motion are successful, which constitutes an excellent validation of the model, from the dynamical and the haptic points of view. Altogether, numerical methods for non-smooth contact dynamics applied to a non-smooth model of the piano action proved to be both accurate and efficient, opening doors to industrial and haptic applications of sensitive multibody systems for which dry friction is essential.

Appendix: Models for estimating the rigid body approximation for the key and the hammer

The purpose of this appendix is to give the details of the estimation, for the key and the hammer, of:

• their first modal frequency (dynamics);

• their flexibility as linear elastic bodies compared the flexibility of the felts (statics).

Four different models were considered in total: two for each piece, see Fig. 13.

Fig. 13

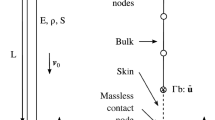
Models used for investigating the rigid body approximation of the key and the hammer. a Beam model of the key, b beam model of the hammer shank, c rigid key in contact with the felt of the blocked whippen, d rigid hammer in contact with the felt of the knuckle and the blocked jack. The sliding hinge joint element in a and b blocks translation in the out-of-plane direction only: it represents the whippen–key contact for the key (left) and the hammer roller–jack contact for the hammer (right). a Elastic key model, b elastic hammer model, c rigid key model, d rigid hammer model

The first mode of vibration was approximated using the Rayleigh–Ritz method (see for example [18]). The mass of the key was assumed to be equally distributed while for the hammer, it was assumed to be concentrated at the center of the hammer head. The static deformations $\varphi _{{\text {key}}}$, $\varphi _{{\text {hammer}}}$ in response to the corresponding weight were calculated and used as the shape functions for the Rayleigh quotient. With point O corresponding to the origin of the x-axis, the calculated expressions are:

$$\begin{aligned} \varphi _{{\text {key}}}(x)= \left\{\begin{array}{ll} \dfrac{1}{24 EI\,L_2}(-2L_1^2(L_2^2-x^2)+L_2\left(L_2^3-2L_2x^2+x^3\right) &{}\quad {\text {for}}\quad x\in [0,L_2] \\ \dfrac{1}{24EI}(L_2-x)\left(2L_2^3+2L_1^2(L_2-3x)+4L_1(L_2-x)^2-3L_2^2x+3L_2x^2-x^3\right)&{}\quad {\text {for }}\quad x\in (L_2,L1+L2] \end{array}\right. \end{aligned}$$

(20)

and

$$\begin{aligned} \varphi _{{\text {hammer}}}(x)= \left\{ \begin{array}{ll} \dfrac{L_1}{6 EI\,L_2}x(L_2-x)(L_2+x) &{}\quad {\text {for}}\quad x\in [0,L_2] \\ \dfrac{-1}{6EI}\left(L_1(L_2-3x)+(L_2-x)^2\right)(L_2-x)&{}\quad {\text {for}}\quad x\in (L_2,L1+L2] \end{array}\right. \end{aligned}$$

(21)

An approximation of the frequency of the first eigenmode is $\sqrt{R}/(2\pi )$ where R is the Rayleigh quotient

$$\begin{aligned} R=\dfrac{\int _{0}^{L_1+L_2}EI\varphi ''(x)^2{\text {d}}x}{\int _{0}^{L_1+L_2}\rho S\varphi (x)^2{\text {d}}x} \end{aligned}$$

(22)

The calculations yield $f_{{\text {1,key}}}={355}\,{\,{\hbox {Hz}}}$ and $f_{{\text {1,hammer}}}={39}\,{\,{\hbox {Hz}}}$.

The flexibilities $\psi ^{{\text {e}}}_{{\text {key, hammer}}}$ of the key as seen from the finger, or of the hammer as seen from the hammer head, and due to the elasticity of wood, can be estimated with the same models (see Fig. 13a, b). The strain energy U of the beams for a force F applied at position $L_1+L_2$ is given by the integration of the squared moment over the length, divided by 2EI; it comes:

$$\begin{aligned} U=\dfrac{F^2}{6EI}{L_1}^2(L_1+L_2) \end{aligned}$$

(23)

The flexibility $\psi ^{{\text {e}}}$ is given by Castigliano theorem (see for example [31]):

$$\begin{aligned} \psi ^{{\text {e}}}=\dfrac{1}{3EI}{L_1}^2(L_1+L_2) \end{aligned}$$

(24)

With $L_1={0.18}\,{\hbox {m}}$, $L_2={0.13}\,{\hbox {m}}$ for the key and $L_1={0.13}\,{\hbox {m}}$, $L_2={0.016}\,{\hbox {m}}$ for the hammer, the flexibilities for the elastic model with rigid boundary conditions are $\psi ^{{\text {e}}}_{{\text {key}}}={1.5 \times 10^{-5}}{\,{\hbox {m}\,\mathrm{N}}^{-1}}$ and $\psi ^{{\text {e}}}_{{\text {hammer}}}={1.2 \times 10^{-3}}{\,{\hbox {m}\,\mathrm{N}}^{-1}}$ (the value of the other parameters is given in Table 3).

The flexibilities $\psi ^{{\text {f}}}_{{\text {location}}}$ due to felts only, estimated at a given location, come as follows: each beam is considered rigid, with its motion limited by nearby felts (see Fig. 13c, d). For the nonlinear felt law given in Eq. (6), the average flexibility is

$$\begin{aligned} \psi ^{{\text {f}}}_{{\text {felt}}}=\frac{\delta }{F_{{\text {felt}}}}=k^{(-1/r)}F_{{\text {felt}}}^{(1-r)/r} \end{aligned}$$

(25)

The ratio of the flexibilities at the finger end of the key (respectively, at the hammer’s head) and at the corresponding felt (whippen and knuckle, respectively) is $\psi ^{{\text {f}}}_{{\text {key, hammer}}}/\psi ^{{\text {f}}}_{{\text {felt}}}=\lambda ^2$ where $\lambda =L_1/L_2$ (respectively $(L_1+L_2)/L_2$). Since the force applied at the finger end (respectively at the hammer’s head position) is $F_{{\text {key, hammer}}}=F_{{\text {felt}}}/\lambda$, it comes

$$\begin{aligned} \psi ^{{\text {f}}}_{{\text {key, hammer}}}=k^{(-1/r)}\lambda ^{(r+1)/r}F_{{\text {key, hammer}}}^{(1-r)/r} \end{aligned}$$

(26)

Since the felts are nonlinear springs, it is normal that the flexibility depends on the force level.

For the key, 5 N represents a typical force level exerted by the finger. For the hammer, the mass is concentrated at its head. With a mass of 12 g, typical of the bass hammers, a forte keystroke corresponds to a displacement of ${5\,\hbox {cm}}$ of the head in about ${20\,\hbox {ms}}$, hence an average inertia force $F={3\,\hbox {N}}$ at the head position. With the parameters values $k=1.6\times 10^{10}$ uSI and $r=2.7$ for the whippen-key felt and $k=7\times 10^9$ uSI and $r=3$ for the knuckle’s felt, the flexibilities are $\psi ^{{\text {f}}}_{{\text {key}}}={9.5 \times 10^{-5}}{\,{\hbox {m}\,\mathrm{N}}^{-1}}$ and $\psi ^{{\text {f}}}_{{\text {hammer}}}={4.8 \times 10^{-3}}{\,{\hbox {m}\,\mathrm{N}}^{-1}}$. For lower force levels, the flexibilities are larger since the felts are hardening springs.