Skip to main content
SpringerLink
Log in

Bose–Einstein Condensation of Nonideal Cooper Pairs in the Hartree–Fock–Popov Theory

  • Published:
Foundations of Physics Aims and scope Submit manuscript

Abstract

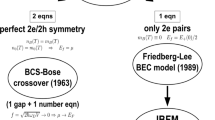
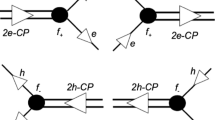
The Hartree–Fock–Popov theory of interacting Bose particles is generalized to the Cooper-pair system with a screened Coulomb repulsive interaction in high-temperature superconductors. At zero temperature, it is found that the condensate density \(n_c(0)\) of Cooper pairs is of the order \(n_c(0)\simeq 10^{18}\) cm\(^{-3}\), consistently with the fact that a small fraction of the total p holes participate in pairing. We find that the phonon velocity c(0) at zero temperature is of the order \(c(0)\simeq 10\) km s\(^{-1}\). The computation shows that the transition temperature \(T_c\) is a dome-shaped function of the p hole concentration \(\delta \), which is consistent with experiments. At finite temperature, we find that the condensate fraction \(n_c(T)/n\) decreases continuously from \(n_c(0)/n\) to zero as the temperature increases from zero to the transition temperature \(T_c\). For higher temperatures, we find that the repulsive interaction between Cooper pairs drives more Cooper pairs into the condensate. The computation reveals that the phonon velocity c(T) decreases continuously from c(0) to zero as the temperature increases from zero to the transition temperature \(T_c\). The Cooper-pair system undergoes a first-order phase transition from the normal state to the BEC state.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Cooper, L.N.: Bound electron pairs in a degenerate Fermi gas. Phys. Rev. 104, 1189 (1956)

    Article  ADS  MATH  Google Scholar 

  2. Bardeen, J., Cooper, L.N., Schrieffer, J.R.: Theory of superconductivity. Phys. Rev. 108, 1175 (1957)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Bednorz, J.G., Müller, K.A.: Possible high T\(_c\) superconductivity in the Ba–La–Cu–O system. Z. Phys. B 64, 189 (1986)

    Article  ADS  Google Scholar 

  4. Xu, Z.A., Ong, N.P., Wang, Y., Kakeshita, T., Uchida, S.: Vortex-like excitations and the onset of superconducting phase fluctuation in underdoped La\(_{2-x}\). Nature (London) 406, 486 (2000)

    Article  ADS  Google Scholar 

  5. Wang, Y., Li, L., Ong, N.P.: Nernst effect in high-T\(_c\) superconductors. Phys. Rev. B 73, 024510 (2006)

    Article  ADS  Google Scholar 

  6. Li, L., Wang, Y., Komiya, S., Ono, S., Ando, Y., Gu, G.D., Ong, N.P.: Diamagnetism and Cooper pairing above T\(_c\) in cuprates. Phys. Rev. B 81, 054510 (2010)

    Article  ADS  Google Scholar 

  7. Corson, J., Mallozzi, R., Orenstein, J., Eckstein, J.N., Bozovic, I.: Vanishing of phase coherence in underdoped Bi\(_2\). Nature (London) 398, 221 (1999)

    Article  ADS  Google Scholar 

  8. Meingast, C., Pasler, V., Nagel, P., Rykov, A., Tajima, S., Olsson, P.: Phase fluctuations and the pseudogap in YBa\(_2\)Cu\(_3\)O\(_x\). Phys. Rev. Lett. 86, 1606 (2001)

    Article  ADS  Google Scholar 

  9. Wen, H.H., Mu, G., Luo, H.Q., Yang, H., Shan, L., Ren, C., Cheng, P., Yan, J., Fang, L.: Specific-heat measurement of a residual superconducting state in the normal state of underdoped Bi\(_2\)Sr\(_{2-x}\)La\(_x\)CuO\(_{6+\delta }\) cuprate superconductors. Phys. Rev. Lett. 103, 067002 (2009)

    Article  ADS  Google Scholar 

  10. Stewart Jr., M.D., Yin, A.J., Xu, J.M., Valles Jr., J.M.: Superconducting pair correlations in an amorphous insulating nanohoneycomb film. Science 318, 1273 (2007)

    Article  ADS  Google Scholar 

  11. Friedberg, R., Lee, T.D.: Gap energy and long-range order in the boson-fermion model of superconductivity. Phys. Rev. B 40, 6745 (1989)

    Article  ADS  Google Scholar 

  12. Cheng, Ze: Bipolaronic superconductivity in antiferromagnets. Z. Phys. B 91, 161 (1993)

    Article  ADS  Google Scholar 

  13. de Llano, M., Annett, J.F.: Generalized Cooper pairing in superconductors. Int. J. Mod. Phys. B 21, 3657 (2007)

    Article  ADS  MATH  Google Scholar 

  14. Mamedov, T., de Llano, M.: Superconducting pseudogap in a Boson–Fermion model. J. Phys. Soc. Jpn. 79, 044706 (2010)

    Article  ADS  Google Scholar 

  15. Mamedov, T., de Llano, M.: Generalized superconducting gap in an anisotropic boson-fermion mixture with a uniform Coulomb field. J. Phys. Soc. Jpn. 80, 074718 (2011)

    Article  ADS  Google Scholar 

  16. Fujita, S.: On the Bose–Einstein condensation of nonzero-momentum cooper pairs. A second-order phase transition. J. Supercond. 4, 297 (1991)

    Article  ADS  Google Scholar 

  17. Tolmachev, V.V.: Superconducting Bose–Einstein condensates of Cooper pairs interacting with electrons. Phys. Lett. A 266, 400 (2000)

    Article  ADS  Google Scholar 

  18. Rosencwaig, A.: Bose–Einstein condensation model for high-temperature superconductivity. Phys. Rev. B 67, 184514 (2003)

    Article  ADS  Google Scholar 

  19. Wollman, D.A., Van Harlingen, D.J., Lee, W.C., Ginsberg, D.M., Leggett, A.J.: Experimental determination of the superconducting pairing state in YBCO from the phase coherence of YBCO-Pb dc SQUIDs. Phys. Rev. Lett. 71, 2134 (1993)

    Article  ADS  Google Scholar 

  20. Tsuei, C.C., Kirtley, J.R., Chi, C.C., Yu-Jahnes, L.S., Gupta, A., Shaw, T., Sun, J.Z., Ketchen, M.B.: Pairing symmetry and flux quantization in a tricrystal superconducting ring of YBa\(_2\)Cu\(_3\)O\(_{7-\delta }\). Phys. Rev. Lett. 73, 593 (1994)

    Article  ADS  Google Scholar 

  21. Valla, T., Fedorov, A.V., Lee, J., Davis, J.C., Gu, G.D.: The ground state of the pseudogap in cuprate superconductors. Science 314, 1914 (2006)

    Article  ADS  Google Scholar 

  22. Lee, P.A., Nagaosa, N., Wen, X.G.: Doping a Mott insulator: physics of high-temperature superconductivity. Rev. Mod. Phys. 78, 17 (2006)

    Article  ADS  Google Scholar 

  23. Norman, M.R., Pines, D., Kallin, C.: The pseudogap: friend or foe of high T\(_c\)? Adv. Phys. 54, 715 (2005)

    Article  ADS  Google Scholar 

  24. Anderson, P.W., Lee, P.A., Randeria, M., Rice, T.M., Trivedi, N., Zhang, F.C.: The physics behind high-temperature superconducting cuprates: the ’plain vanilla’ version of RVB. J. Phys. Condens. Matter 16, R755 (2004)

    Article  ADS  Google Scholar 

  25. Emery, V.J., Kivelson, S.A.: Importance of phase fluctuations in superconductors with small superfluid density. Nature (London) 374, 434 (1995)

    Article  ADS  Google Scholar 

  26. Weng, Z.Y., Sheng, D.N., Ting, C.S.: Bosonic resonating-valence-bond description of a doped antiferromagnet. Phys. Rev. Lett. 80, 5401 (1998)

    Article  ADS  Google Scholar 

  27. Wang, Y., Li, L., Naughton, M.J., Gu, G.D., Uchida, S., Ong, N.P.: Field-enhanced diamagnetism in the pseudogap state of the cuprate Bi\(_2\)Sr\(_2\)CaCu\(_2\)O\(_{8+\delta }\) superconductor in an intense magnetic field. Phys. Rev. Lett. 95, 247002 (2005)

    Article  ADS  Google Scholar 

  28. Chen, Q.J., Stajic, J., Tan, S.N., Levin, K.: BCS-BEC crossover: from high temperature superconductors to ultracold superfluids. Phys. Rep. 412, 1 (2005)

    Article  ADS  Google Scholar 

  29. Chen, Q.J., Kosztin, I., Jankó, B., Levin, K.: Pairing fluctuation theory of superconducting properties in underdoped to overdoped cuprates. Phys. Rev. Lett. 81, 4708 (1998)

    Article  ADS  Google Scholar 

  30. Adhikari, S.K., de Llano, M., Sevilla, F.J., Solís, M.A., Valencia, J.J.: The BCS-Bose crossover theory. Physica C 453, 37 (2007)

    Article  ADS  Google Scholar 

  31. Nozières, P., Schmitt-Rink, S.: Bose condensation in an attractive fermion gas: from weak to strong coupling superconductivity. J. Low Temp. Phys. 59, 195 (1985)

    Article  ADS  Google Scholar 

  32. Fetter, A.L., Walecka, J.D.: Quantum Theory of Many-Particle Systems, p. 198. McGraw-Hill, New York (1971)

    Google Scholar 

  33. Shi, H., Griffin, A.: Finite-temperature excitations in a dilute Bose-condensed gas. Phys. Rep. 304, 1 (1998)

    Article  ADS  Google Scholar 

  34. Beliaev, S.T.: Application of the methods of quantum field theory to a system of Bosons. Sov. Phys. JETP 7, 289 (1958)

    MathSciNet  Google Scholar 

  35. Hugenholtz, N.M., Pines, D.: Ground-state energy and excitation spectrum of a system of interacting Bosons. Phys. Rev. 116, 489 (1959)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  36. Popov, V.N.: Green functions and thermodynamic functions of a non-ideal Bose gas. Sov. Phys. JETP 20, 1185 (1965)

    MathSciNet  Google Scholar 

  37. Huang, K.S.: Statistical Mechanics, 2nd edn, p. 286. Wiley, New York (1987)

    Google Scholar 

  38. Sleight, A.W.: Chemistry of high-temperature superconductors. Science 242, 1519 (1988)

    Article  ADS  Google Scholar 

  39. Ranninger, J., Robaszkiewicz, S.: Superconductivity of locally paired electrons. Physica B 135, 468 (1985)

    Article  Google Scholar 

  40. Kostyrko, T., Ranninger, J.: Spectral properties of the boson-fermion model in the superconducting state. Phys. Rev. B 54, 13105 (1996)

    Article  ADS  Google Scholar 

Download references

Acknowledgments

This work was supported by the National Natural Science Foundation of China under Grants Nos. 10174024 and 10474025.

Author information

Authors and Affiliations

  1. School of Physics, Huazhong University of Science and Technology, Wuhan, 430074, People’s Republic of China

    Ze Cheng

Authors
  1. Ze Cheng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ze Cheng.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Cheng, Z. Bose–Einstein Condensation of Nonideal Cooper Pairs in the Hartree–Fock–Popov Theory. Found Phys 46, 915–942 (2016). https://doi.org/10.1007/s10701-016-9993-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10701-016-9993-y

Keywords

Search

Navigation