Abstract
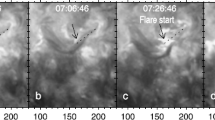
We present a study of a mini-filament erupting in association with a circular ribbon flare observed by NVST and SDO/AIA on 2014 March 17. The filament was located at one footpoint region of a large loops. The potential field extrapolation shows that it was embedded under a magnetic null point configuration. First, we observed a brightening of the filament at the corresponding EUV images, close to one end of the filament. With time evolution, a circular flare ribbon was observed around the filament at the onset of the eruption, which is regarded as a signature of reconnection at the null point. After the filament activation, its eruption took the form of a surge, which ejected along one end of a large-scale closed coronal loops with a curtain-like shape. We conjecture that the null point reconnection may facilitate the eruption of the filament.





Similar content being viewed by others
References
Alissandrakis, C.E.: Astron. Astrophys. 100, 197 (1981)
Antiochos, S.K.: Astrophys. J. 502, L181 (1998)
Antiochos, S.K., DeVore, C.R., Klimchuk, J.A.: Astrophys. J. 510, 485 (1999)
Aulanier, G., Pariat, E., Dèmoulin, P.: Astron. Astrophys. 444, 961 (2005)
Bi, Y., Jiang, Y., Yang, J., et al.: Astrophys. J. 805, 48 (2015)
Demoulin, P., Bagala, L.G., Mandrini, C.H., Henoux, J.C., Rovira, M.G.: Astron. Astrophys. 325, 305 (1997)
Feng, S., Deng, L., Shu, G., et al.: Advanced Computational Intelligence (ICACI). In: 2012 IEEE Fifth International Conference, p. 626 (2012)
Gekelman, W., Lawrence, E., Van Compernolle, B.: Astrophys. J. 753, 131 (2012)
Haynes, A.L., Parnell, C.E.: Phys. Plasmas 14, 082107 (2007)
Hong, J., Jiang, Y., Zheng, R., et al.: Astrophys. J. Lett. 738, L20 (2011)
Hong, J., Jiang, Y., Yang, J., et al.: Astrophys. J. 830, 60 (2016)
Hong, J., Jiang, Y., Yang, J., et al.: Astrophys. J. 835, 35 (2017)
Jiang, C., Feng, X., Wu, S.T., Hu, Q.: Astrophys. J. Lett. 771, L30 (2013)
Joshi, N.C., Liu, C., Sun, X., et al.: Astrophys. J. 812, 50 (2015)
Lawrence, E.E., Gekelman, W.: Phys. Rev. Lett. 103, 105002 (2009)
Lemen, J.R., Title, A.M., Akin, D.J., et al.: Sol. Phys. 275, 17 (2012)
Li, H., Jiang, Y., Yang, J., et al.: Astrophys. Space Sci. 359, 4 (2015a)
Li, X., Yang, S., Chen, H., Li, T., Zhang, J.: Astrophys. J. Lett. 814, L13 (2015b)
Li, H., Jiang, Y., Yang, J., et al.: Astrophys. J. 836, 235 (2017a)
Li, H., Jiang, Y., Yang, J., et al.: Astrophys. J. Lett. 842, L20 (2017b)
Liu, Z., Beckers, J.M.: Sol. Phys. 198, 197 (2001)
Liu, C., Lee, J., Jing, J., et al.: Astrophys. J. Lett. 721, L193 (2010)
Liu, Z., Xu, J., Gu, B., et al.: Res. Astron. Astrophys. 14, 705 (2014)
Liu, C., Deng, N., Liu, R., et al.: Astrophys. J. Lett. 812, L19 (2015)
Liu, R., Kliem, B., Titov, V.S., Chen, J., Wang, Y., Wang, H., Liu, C., Xu, Y., Wiegelmann, T.: Astrophys. J. 818, 148 (2016)
Mandrini, C.H., Demoulin, P., Henoux, J.C., Machado, M.E.: Astron. Astrophys. 250, 541 (1991)
Masson, S., Pariat, E., Aulanier, G., Schrijver, C.J.: Astrophys. J. 700, 559 (2009)
Milano, L.J., Dmitruk, P., Mandrini, C.H., Gèomez, D.O., Dèmoulin, P.: Astrophys. J. 521, 889 (1999)
Moore, R.L., Cirtain, J.W., Sterling, A.C., Falconer, D.A.: Astrophys. J. 720, 757 (2010)
Pariat, E., Antiochos, S.K., DeVore, C.R.: Astrophys. J. 691, 61 (2009)
Pesnell, W.D., Thompson, B.J., Chamberlin, P.C.: Sol. Phys. 275, 3 (2012)
Priest, E.R., Forbes, T.G.: Astron. Astrophys. 10, 313 (2002)
Rachmeler, L.A., Pariat, E., DeForest, C.E., Antiochos, S., Torok, T.: Astrophys. J. 715, 1556 (2010)
Reid, H.A.S., Vilmer, N., Aulanier, G., Pariat, E.: Astron. Astrophys. 547, A52 (2012)
Roy, J.R.: Sol. Phys. 32, 139 (1973)
Schou, J., Scherrer, P.H., Bush, R.I., et al.: Sol. Phys. 275, 229 (2012)
Shen, Y., Liu, Y., Su, J., Ibrahim, A.: Astrophys. J. 735, L43 (2011)
Shen, Y., Liu, Y., Su, J.: Astrophys. J. 750, 12 (2012a)
Shen, Y., Liu, Y., Su, J., Deng, Y.: Astrophys. J. 745, 164 (2012b)
Shen, Y., Liu, Y., Liu, Y.D., et al.: Astrophys. J. Lett. 814, L17 (2015)
Shen, Y., Liu, Y.D., Su, J., Qu, Z., Tian, Z.: Astrophys. J. 851, 67 (2017)
Sterling, A.C., Moore, R.L., Falconer, D.A., Adams, M.: Nature 523, 437 (2015)
Sun, X., Hoeksema, J.T., Liu, Y., et al.: Astrophys. J. 778, 139 (2013)
Tian, Z., Liu, Y., Shen, Y., et al.: Astrophys. J. 845, 94 (2017)
Titov, V.S., Hornig, G., Dèmoulin, P.: J. Geophys. Res. Space Phys. 107, 1164 (2002)
Titov, V.S., Miki, Z., Linker, J.A., Lionello, R., Antiochos, S.K.: Astrophys. J. 731, 111 (2011)
Wang, H., Liu, C.: Astrophys. J. 760, 101 (2012)
Wang, J., Li, W., Denker, C., et al.: Astrophys. J. 530, 1071 (2000)
Wang, J., Yan, X., Qu, Z., et al.: Astrophys. J. 817, 156 (2016)
Weigelt, G.P.: Opt. Commun. 21, 55 (1977)
Wilmot-Smith, A.L., Pontin, D.I., Hornig, G.: Astron. Astrophys. 516, A5 (2010)
Xiang, Y-y., Liu, Z., Jin, Z-y.: New Astron. 49, 8 (2016)
Xu, Z., Yang, K., Guo, Y., et al.: Astrophys. J. 851, 30 (2017)
Xue, Z., Yan, X., Cheng, X., et al.: Nat. Commun. 7, 11837 (2016)
Yan, X.L., Xue, Z.K., Pan, G.M., et al.: Astrophys. J. Suppl. Ser. 219, 17 (2015)
Yang, J., Jiang, Y., Yang, B., et al.: New Astron. 17, 732 (2012)
Yang, S., Zhang, J., Xiang, Y.: Astrophys. J. Lett. 793, L28 (2014)
Yang, K., Guo, Y., Ding, M.D.: Astrophys. J. 806, 171 (2015a)
Yang, Y.-F., Qu, H.-X., Ji, K.-F., et al.: Res. Astron. Astrophys. 15, 569 (2015b)
Yang, S., Zhang, J., Xiang, Y.: Astrophys. J. Lett. 798, L11 (2015c)
Yang, L., Yan, X., Li, T., et al.: Astrophys. J. 838, 131 (2017)
Zhang, Q.M., Ning, Z.J., Guo, Y., et al.: Astrophys. J. 805, 4 (2015)
Acknowledgements
We thank an anonymous referee for providing detailed suggestions and comments that significantly improved the presentation of this paper. We are grateful to the NVST, AIA, and HMI teams for data support. This work is supported by the National Natural Science Foundation of China under grants 11633008, 11333007, 11703084, 11503081, 11573012, and 11503082, by the CAS programs “Light of West China” and “QYZDJ-SSW-SLH012”, by Foundation of Sichuan University of Science and Engineering grant 2015RC43, and by the grant associated with the Project of the Group for Innovation of Yunnan Province.
Author information
Authors and Affiliations
Corresponding author
Electronic Supplementary Material
Below is the link to the electronic supplementary material.
Rights and permissions
About this article
Cite this article
Li, H., Yang, J., Jiang, Y. et al. The surge-like eruption of a miniature filament associated with circular flare ribbon. Astrophys Space Sci 363, 26 (2018). https://doi.org/10.1007/s10509-017-3244-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10509-017-3244-3